In 1959, Lytteton and Bondi suggest that the expansion of the Universe could be explained fi matter carried a net charge. Suppose that the Universe is made up of hydrogen atoms with a number density N, which is mainted a constant. Let the charge on the proton be , ep=−(1+q) e where e si the electronic charge.
(a) Find the critical value of y such that expansion may start.
(b) Show that the velocity of expansion is propertional to the distance from the center.
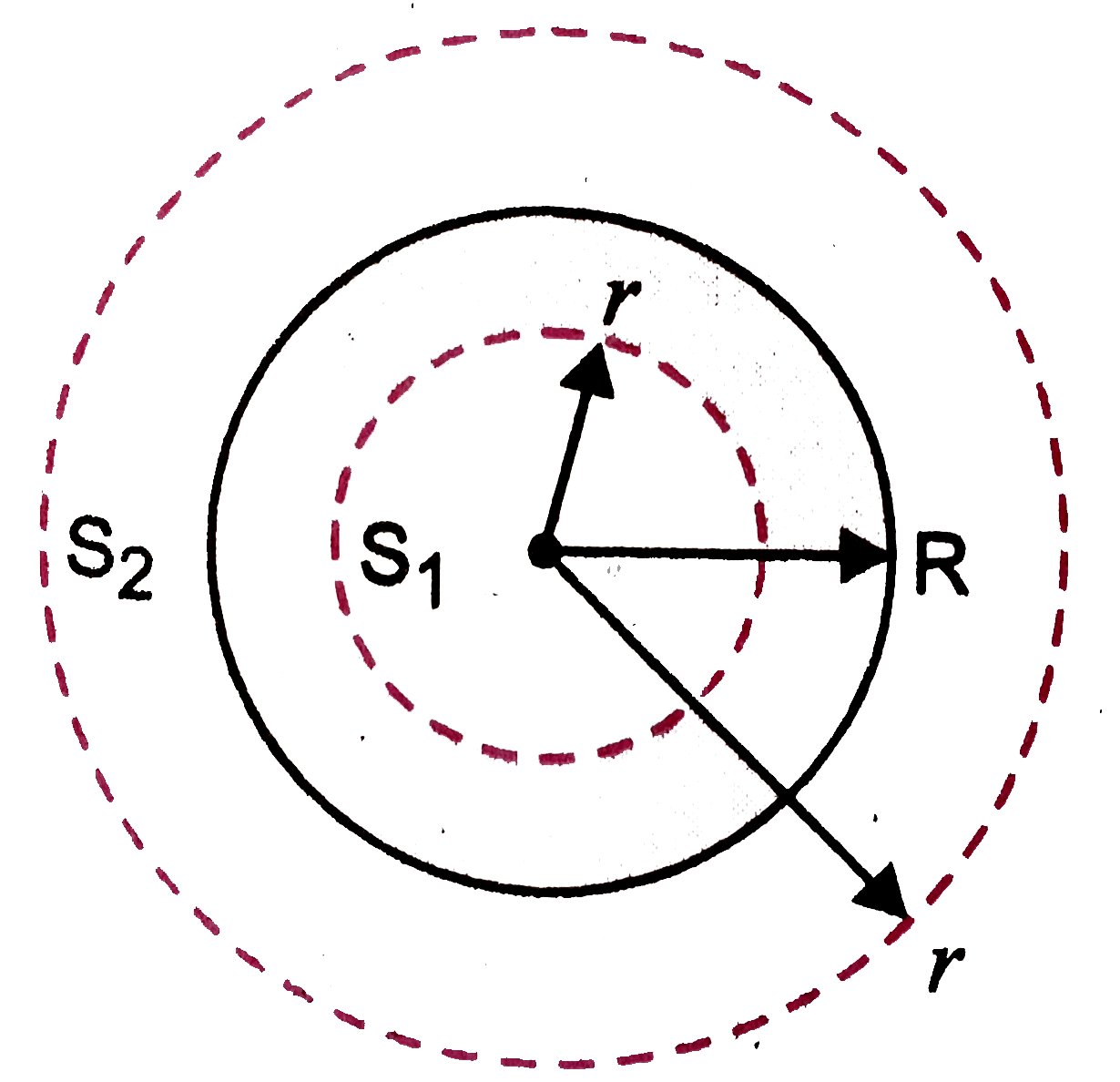
(a) Suppose the universe is a sphere of radius R and its constituent hydrogen atoms are distributed uniformly in the sphere.
As hydrogen atom contains one proton and one electron, therefore, charge on each hydrogen atom,
eH=ep+e=−(1+y)e+e=−ye=(ye)
if E electric field intensity at distance R, on the surface of the sphere, then according to Gauss's theroem,
∮→E.→ds=q∈0,i.e.,E(4πR2)=43πR2N|ye|∈0
E=13N|ye|R∈0 ...(i)
Now, mass of each hydrogen atom = mp = mass of a proton.
If GR is gravitational field at distnace R on the surface of the sphere, then
−4πR2GR=4πG,mp(43πR3N)orGR=−43πGmpNR ...(ii)
∴ Gravitational force on this atom is F1=mp×GR=−4π3Gm2pNR ...(iii)
and coulomb force on hydrogen atom at R is F2=(ye)E=13Ny2e2R∈0 using (i)
The expansion would start when coulomb repulsion (F2) on hydrogen atom is larger than the gravitational force of attraction (F1) on the hydrogen atom. The critical value of y to start expansion would be when
F2=F1i.e.,13Ny2e2R∈0=4π3Gm2pNR
or y2=(4π∈0)G(mpe)2=19×109=(6.67×10−11)(1.66×10−27)2(1.6×10−19)2=79.8×10−38
y=√79.8×10−38=8.9×10−19=10−18
This is the critical value of y corresponding to which expansion of universe would start.
(b) Net force experienced by the hydrogen atom, F=F2−F1=13Ny2e2R∈0−4π3Gm2pNR
If acceleration of hydrogen atom is represended by d2R/dr2, then
mpd2Rdr2=F=13Ny2e2∈0R−4π3Gm2pNR=(13Ny2e2∈0−4π3Gm2pN)R
∴d2Rdr2=1mp[13Ny2e2∈0−4π3Gm2pN]R=α2R
where α2=1mp[13Ny2e2∈0−4π3Gm2pN]
The genral solution of eqn. (iv) is R=Aeαt+Be−αt
As we are looking for expansion, B=0.∴R=Aeαt
velocity of expansions, v=dRdr=Aeαt(α)=αAeαt=αR
Hence, v∝R, which was to be proved.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - VALUE BASED QUESTIONS
7 Videos
- ELECTROSTATICSBOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - Exercise
414 Videos
- ELECTROSTATICSBOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL QUESTIONS
2 Videos
- ELECTRONIC DEVICESBOOK - PRADEEPCHAPTER - ELECTRONIC DEVICESEXERCISE - Fill in the Blanks
1 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Competition Focus (Multiple Choice Questions)
2 Videos
Similar Questions
In 1959 Lyttleton and Bondi suggested that the expansion of the universe could be explained if matter carried a ent charge. Suppose that the universe is made up of hydrogen atoms with a number density N, which is maintained a constant. Let the charge on the proton be ep=−(1+y)e where e is the electronic charge.
(a). find the critical value of y such that expansion may start. ltBrgt (b) show that the velocity of expansion is proportional tot he distance from the centre.
View SolutionConsider a sphere of radius R with charge density distributed as ρ(R)=kr for r≤Rand=0 for r>R.
(a) Find the electric field at all points r.
(b) suppose the total charge on the sphere is 2e, where e is the electron charge. Where can two protons be embedded such that the force on each of them is zero. Assume that the introduction of the proton does not alter the negative charge distribution.
View Solution
Knowledge Check
The kinetic energy of the electron in an orbit of radius r in hydrogen atom is (e= electronic charge)
Ae2r2Be22rCe2rDe22r2The dimensions of h/e (h= Plank's constant and e= electronic charge ) are same as that of
Amagnetic fieldBelectric fluxCelectric fieldDmagnetic fieldIf m and e are the mass and charge of the revolving electron in the orbit of radius r for hydrogen atom, the total energy of the revolving electron will be :
A12e2rB−e2rCme2rD−12e2r
Consider a sphere of radius R with harge density distributed as p(r)=kr fro r≤R=0 for r>R.
(a). Find the electric field as all points r.
(b) Suppose the total charge on the sphere is 2e where e is the electron charge. Where can two protons be embedded such that the force on each of them is zero. Assume that the introduction of the proton does not alter the negative charge distribution.
View SolutionThe dimensions of h/e (h= Plank's constant and e= electronic charge ) are same as that of
View SolutionTwo point objects carry positive charges of magnitude 5e and 20e . Separation between the charges is 1 cm. Find the location of points on the line joining the two charges where the electric field intensity is zero.
View SolutionThe electron in the ground state of hydrogen atom produces a magnetic field B ạt the nucleus. This magnetic field' depends on the value of many constants. If e is electronic charge, B is found to be proportional to en. Find n.
View SolutionCharge is distributed within a sphere of radius R with a volume charge density p(r)=Ar2e−2r/a, where A and a are constants. If Q is the total charge of this charge distribution, the radius R is :
View Solution
