A point charge q=1C and mass 1kg is projected with speed 10m/s in the perpendicular direction of unifrom electric field E=100V/m. The value of latus rectum of the path followed by charged particle (in meter) is :
The correct Answer is:2
Here, q=1C,m=1kg
v=10m/s,E=100V/m.
In fig, E is along OX and velocity v is along OY.
Let P(x,y) be the position of point charge at any time t∴Y=vt or t=yv=y10
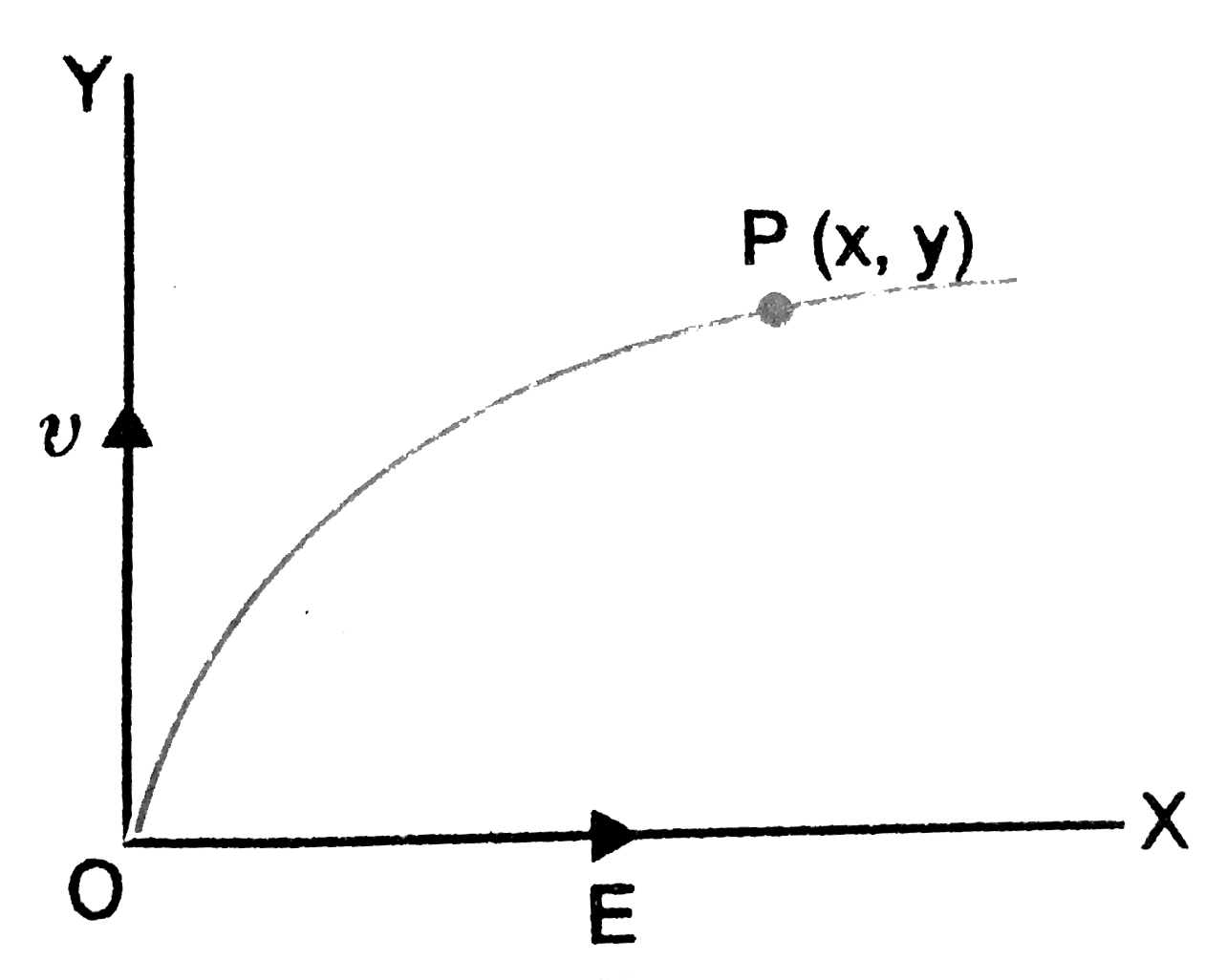
Acceleration along X-axis
ax=qEm=1×1001=100m/s2
As x=12axt2
∴x=12×100t2=50(y10)2=y22
∴y2=2x
Which is the equation of a parabola. Comapare with the stantdard equaction of parabola , y2=4ax, we find 4a=2m, which is the length of latus rectum of parabola.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - FILL IN THE BLANKS
8 Videos
- ELECTROSTATICSBOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - PROBLEMS FOR PRACTICE
8 Videos
- ELECTROSTATICSBOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - VALUE BASED QUESTIONS
7 Videos
- ELECTRONIC DEVICESBOOK - PRADEEPCHAPTER - ELECTRONIC DEVICESEXERCISE - Fill in the Blanks
1 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Competition Focus (Multiple Choice Questions)
2 Videos
Similar Questions
A particle having a charge of 100μC and a mass of 10mg is projected in a uniform magnetic field of 25m T with a speed of 10ms−1. If the velocity is perpendicular to the magnetic field , how long will it take for the particle to come back to its original position for the first time after being projected.
View SolutionA uniform magnetic field of 30 mT exists in the +X direction. A particle of charge +ve and mass 1.67×10−27 kg is projected through the field in the + y direction with a speed of 4.8×106 m/s
(a) find the force on the charged particle in magnitude and direction
(b) find the force if the particle were negatively charged.
(c ) Describe the nature of path followed by the particle in both the cases
View Solution
Knowledge Check
A charged particle is projected perpendicular to the direction of uniform electric field. It's path in the region of the field will be
AellipticBlinearCcircularDparabolicA particle of mass m and charge q is thrown in the vertical direction with a velocity v0 in a uniform horizontal electric field E. Assuming the gravity force to be negligible ,the equation of the path followed by the particle will be
Aparabola ,x=(qE2mv20)y2Bhyperbola , x=qE2mv20yCstraight line ,x=qEy2mv20Dnone of the aboveA particel of charge 1μC& mass 1mg moving with a velocity of 4m/s is subjected to a unifrm electric field of magnitude 300Vm for 10sece. Then it s final speed cannot be :
A0.5m/sB5m/sC3m/sD6m/s
A particle having mass m and charge q is projected with a velocity v making an angle q with the direction of a uniform magnetic field B. Calculate the magnitude of change in velocity of the particle after time t=πm2qB.
View SolutionA particle of mass m=1kg is projected with speed u=20√2m/s at angle θ=45∘ with horizontal find the torque of the weight of the particle about the point of projection when the particle is at the highest point.
View SolutionA particle of mass m=0.1kg and having positive charge q=75μC is suspended from a point by a thread of length l=10cm. In the space, a uniform horizontal electric field E=104NC−1 exists. The particle is drawn aside so that the thread becomes vertical and then it is projected horizontally with velocity v such that the particle starts to move along a circle with the same constant speed V. Calculate the radius of the circle and speed v(g=10ms−2).
View SolutionA positive charge particle with charge q is moving with speed v in a region of uniform magnetic field B at the instant shown in figure. An external electric field so to be applied so that the charged particle follows a straight line path. The magnitude and direction of electric field required are respectively.
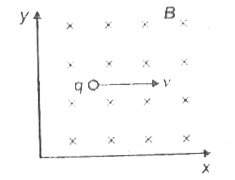
View SolutionAn infinitely long current carrying wire carries current i. A charge of mass m and charge q is projected with speed v parallel to the direction of current at a diatance r from it. Then, the radius of curvature at the point of projection is
View Solution
