Two long parallel wires of negligible resistance are connected at one end to a reasitance R and at the other end to a dc voltage source. The distance between the axes of the wires is η=20 times greater than the cross-sectional radius of each wire. At what value of resistance R does the resultant force of interaction between the wires turn into zero ?
There are excess surface charges on each wire (irrespective of whether the current is flowing through then or net). Hence in addtiion to the magnetic force →Fm, we must take into account the electric force →Fe. Suppose that an excess chagre λ corresponds to a unit length of the wire, then electric force exerted per unit length of the wire by other wire can be found with the help of Gauss's theorem.
Fe=λE=λ14πε02λl=2λ24πε0l ....(1)
where l is the distance between the area of the wires. The magentic force acting per unit length of the wire can be found with the help of the theroem on circularion of vector →B
Fm=μ04π2i2l,
where i is the current in the wire. ..(2)
Now, from the relation,
λ=Cφ, where C is the capacitance of the wires per unit lengths and is given in problem 3.108 and φ=iR
λ=(πε0)'lnηiR or, iλlnηπε0R .....(3)
Dividing (2) by (1) and then substuting the values of iλ from (3) we get,
FmFe=μ0ε0(lnη)2π2R2
The resultant force of interaction vanishes when this ratio unity. This is possible when R=R0, where
R0=√μ0ε0lnηπ=0.36kΩ

Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electromagnetic Induction
84 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Motion Of Charged Particle In Magnetic Field
36 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Current
72 Videos
- ELECTRICITY AND MAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions
6 Videos
- ELECTROMAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions
24 Videos
Similar Questions
Two long parallel wires of negligible resistance are connected at one end to a resistance R and at the other end to a constant voltage source of voltage V. The distance between·the axes of the wires is η times greater than the cross-sectional radiµs of each wire. At what value of resistance R, does the resultant force of interaction between the wires will become zero?
View SolutionTwo long parallel wires are located in a poorly conducting medium with respectivity ρ. The distance between the axes of the wires is equal to l, the cross -section radius of each wire equals a. In the case a<<l find,
(a) the current density at the point equally removed from the axes of the wire by a distance r if the potential difference between teh wires is equal to V,
(b) the electric resistances of the medium per unit length of the wires.
View Solution
Knowledge Check
The resistance of a wire is R. It is bent at the middle by 180∘ and both the ends are twisted together to make a shorter wire. The resistance of the new wire is
A2RBR/2CR/4DR/8Twelve wires of equal length and same cross-section are connected in the form of a cube. If the resistance of each of the wires is R , then the effective resistance between the two diagonal ends would be
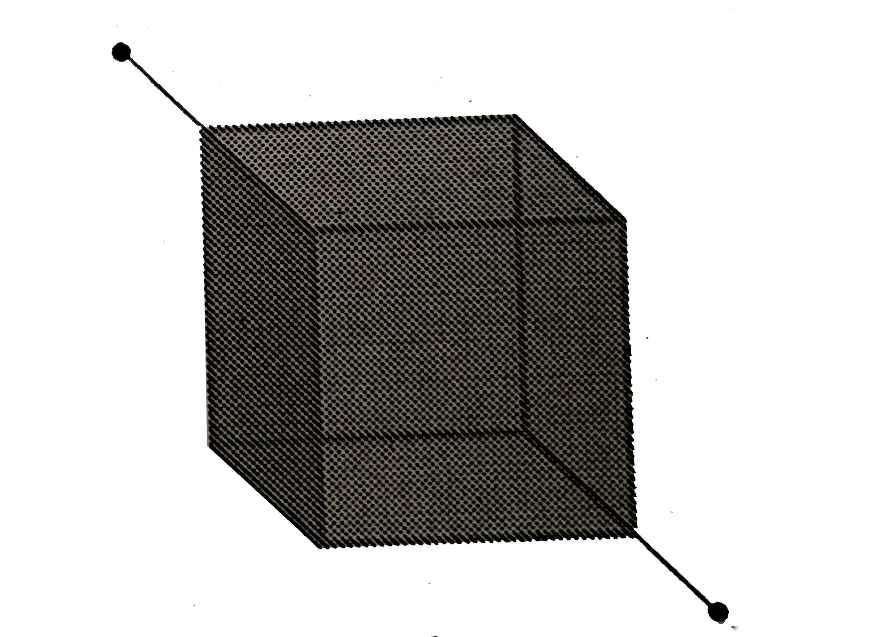 A2RB12RC56RD8R
A2RB12RC56RD8R
Twelve wires of equal resistacne R are connected to form a cube . The effective resistance between two diagonal ends A and E will be .
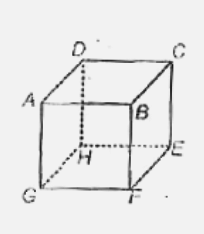
View SolutionThe resistance of a wire is R. It is bent at the middle by 180∘ and both the ends are twisted together to make a shorter wire. The resistance of the new wire is
View Solution" A wire of length L and resistance "R" is stretched to get the radius of cross-section halved.What is the new resistance "
View SolutionTwelve wires of equal length and same cross-section are connected in the form of a cube. If the resistance of each of the wires is R, and the effective resistance between the two diagonal ends is Re, then the ratio RRe is equal to __.
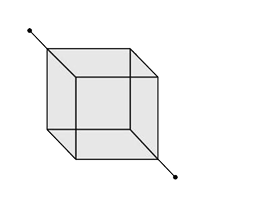
View SolutionTwelve wires of equal length and same cross-section are connected in the form of a cube. If the resistance of each of the wires is R, and the effective resistance between the two diagonal ends is Re, then the ratio RRe is equal to __.

View SolutionAssertion : Two parallel conducting wires carrying currents in opposite direction, come close to each other.
Reason : Parallel currents repel and anti parallel currents attract.
View Solution
