A non-relativistic point charge q moves with a constant velocity v. Using the field transformation formula. Find the magnetic induction B produced by this charge at the point whose position relative to the charge is determind by the radius vector r.
In the reference frame K' moving with the particle,
→E=→E+→v0×→B=q→r4πε0r3
→B=→B−→v0×→E/c2=0
Here, →v0 = velocity of K' relative to the K frame in which the particle has velocity →v Clearly, →v0=→v. From the secound equaction,
→B=→v×→Ec2=ε0μ0×q4πε0→v×→rr3=μ04πq(→v×→r)r3
Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Motion Of Charged Particle In Magnetic Field
36 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Constant Magnetic Fiels - Magnetics
69 Videos
- ELECTRICITY AND MAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions
6 Videos
- ELECTROMAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions
24 Videos
Similar Questions
A point charge q moves unifromly and rectilnearly with a relativistic with a relativistic velocity of light (β=v/c). Find the electric field strength E produced by the charge at the point whose radius vector relatives to the charge is equal to r and forms an angle θ with its velocity vector.
View SolutionA point charge is moving in clockwise direction in a circle with constant speed. Consider the magnetic field produced by the charge at a fiexed point P (not at the centre of circle ) on the axis of the circle. Then,
View Solution
Knowledge Check
The force acting on a charge 'q' moving with a velocity V in a magnetic field of induction B is given by
Aq→V×→BB→V×→B)qCq(→V×→B)D(→V.→B)qA point charge is moving in clockwise direction in a circle with constant speed. Consider the magnetic field produced by the charge at a fiexed point P (not at the centre of circle ) on the axis of the circle. Then,
Ait is constant in magnitude onlyBit is constant in direction onlyCit is constant both in direction and magnitudeDIt is constant neither in magnitude nor in directionA point charge is moving in clockwise direction in a circle with constant speed. Consider the magnetic field produced by the charge at a fixed point P (not at the center of circle) on the axis of the circle. Then,
Ait is constant in magnitude onlyBit is constant in direction onlyCit is constant both in direction and magnitudeDit is constant neither in magnitude nor in direction
A point charge q moves with a non-relativestic velocity v = const. Applying the theorem for the circulation of the vector H around the dotted circle shown in Fig, H at the point A as a function of a radius vector r and velocity v of the charge.
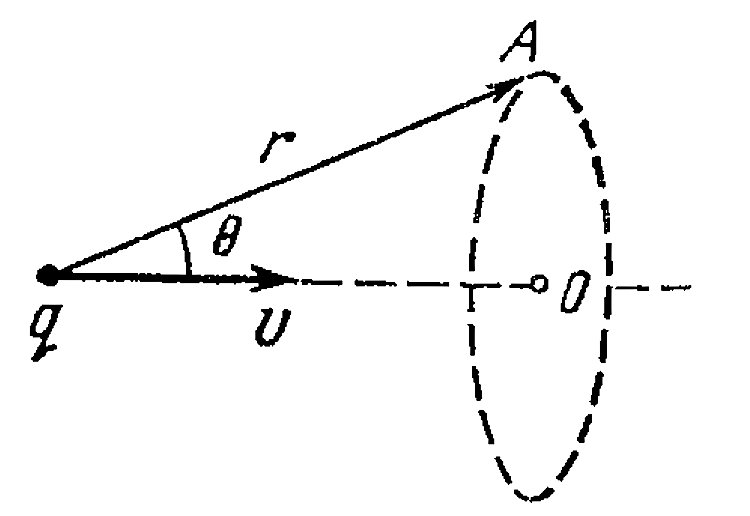
View SolutionA non-relativistic charge q of mass m originates at a point A lying on x-axis and moves with velocity v at an angle to the x-axis. A screen is located at a distance l from A. Find the distance r from the x-axis to the point on the screen into which the charge strikes.
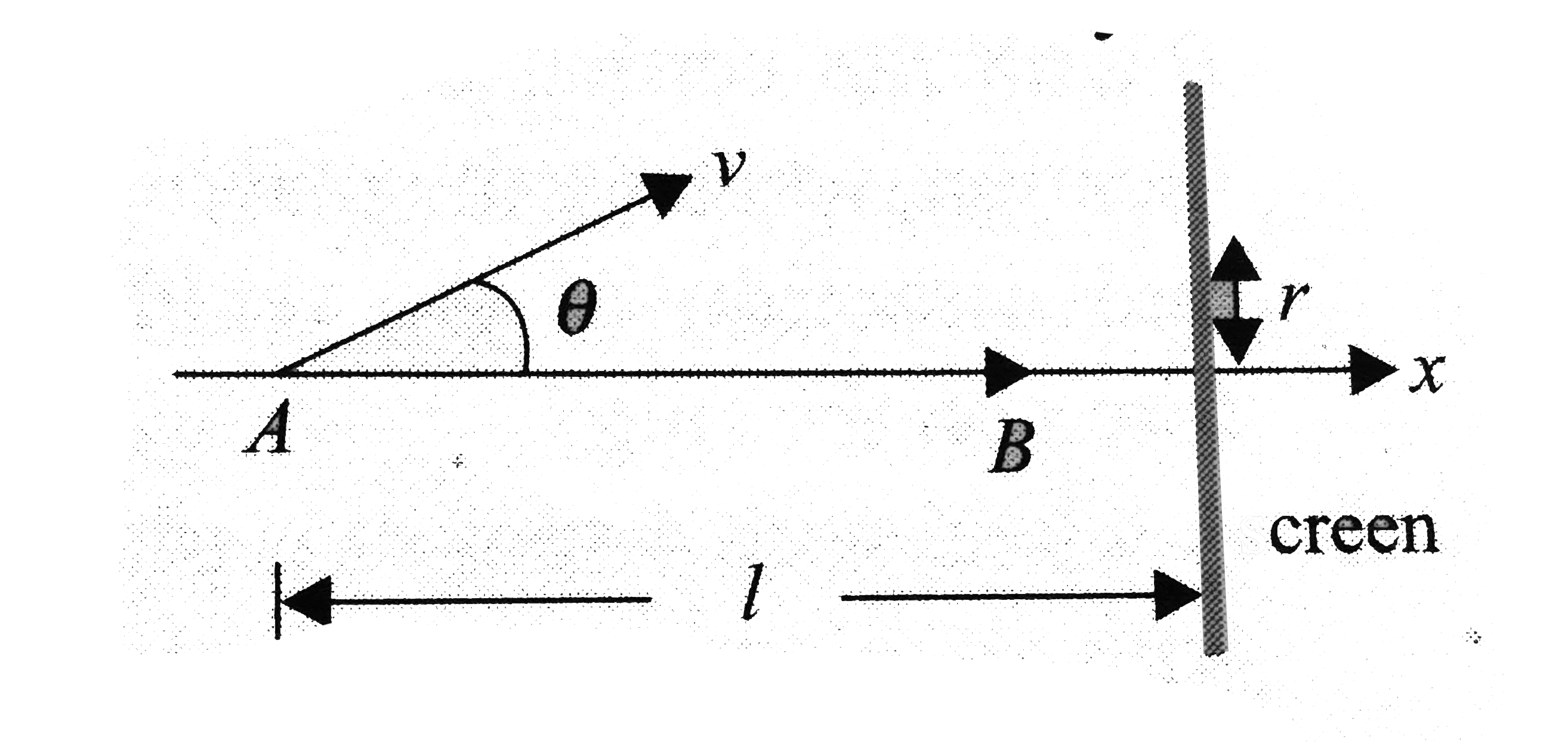
View SolutionA non-relativistic charge q of mass m originates at a point A lying on x-axis and moves with velocity v at an angle to the x-axis. A screen is located at a distance l from A. Find the distance r from the x-axis to the point on the screen into which the charge strikes.

View SolutionAn electric charge q is moving with a velocity w in the direction of a magnetic field B. The magnetic force acting on the charge is
View SolutionA charge moving with velocity v in X-direction is subjected to a field of magnetic induction in the negative X-direction. As a result, the charge will
View Solution
