A plane light wave with wavelength λ=0.50μm falls normally on the face of a glass wedge with an angle Θ=30∘. On the opposite face of the wedge a transparent diffraction grating with period, d=2.00μm is inscibed, whose lines are parallel to the wedge's edge. Find the angles that the direction of incident light froms with the directions to the principle Fraunhofer maxima of the zero and the first order. waht is the highest order of the spectrum? At what angle to the direction of incident light of the observed?
The diffraction formula is easily obtaind on taking account of the fact that the optical path in the glass wedge acquires a factor n(refractive index). We get
d(nsinΘ−sin(Θθk))=kλ
Isnce n>0,Θ−θ0>Θ and so θ0 must be nagative. we get, using Θ=30∘
32×12=sin(30∘−θ0)=sin48.6∘
Thus θ0=−18.6∘
Also for k=1
34−sin(30∘−θ+1)=λd=0.52.0=14
Thus θ+1=0∘
We calaculate θk for varoius k by the above fromula. For k=6.
sin(θk−30∘)=34⇒θk=78.6∘
For k=7
sin(θk−30∘)=+1⇒θk=120∘
This is in admissible. Thus the highest order that can be observed is
k=6
corresponding toθk=78.6∘
(for k=7 the diffracted ray will be grazing the wedge).

Topper's Solved these Questions
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Polarization Of Light
43 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Dispersion And Absorption Of Light
24 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Interference Of Light
33 Videos
- MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems
92 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
Similar Questions
A plane light wave with wavelength λ=0.60μm falls normally on the face of a glass wedge with refracting angle Θ=15∘. The opposite face of the wedge is opaque and has a slit of width b=10μm parallel to the edge. Final:
(a) the angle Δθbetween the direction to the Fqaunhofer maximum of zeroth order and that of incident light,
(b) the angular width of the frqunhofer maximum of the zeroth order.
View SolutionLight with wavelength λ=0.50μm falls on a slit of width b=10μm at an angle θ0=30∘ to its normal. Find the angular position of the first minima located on both sides of the central Fraunhofer maximum.
View SolutionA plane monochromatic light wave with wavelength λ falls on the surface of a glass wedge whose faces from an angle α<<1. The plane of incidence is perpendicualr to the edge, the angle of incidence is θ1. Find the distance between the neighbouring fringe maxima on the screen placed at right angles to reflected light.
View SolutionLight with wavelength λ=589.0nm falls normally on a diffraction grating with period d=2.5μm, comprising N=10000 lines. Find the angular width of the diffraction maximum of second order.
View SolutionLight with wavelength λ=0.55μm from a distant point source falls normally on the surface of a glass wedge. A fringe pattern whose neighbouring maxima on the surface of the wedge are separated by a distance Δx=0.21mm is observed in reflected light. Find:
(a) the angle between the wedge faces,
(b) the degree of light monochromatism (Δλ/λ) if the fringes disappear at a distance l≈1.5cm from the wedge's edge.
View SolutionLight with wavelength 535nm falls normally on a diffraction grating. Find its period if the diffraction angle 35∘ corresponds to one of the Fraunhofer maxima and the highest order of spectrum is equal to five.
View SolutionLight with wavelength 530nm falls on a transparent diffraction grating with period 1.50μm. Find the angle, relative to the grating normal. At which the Fraunhofer maximum of highest order is observed provided the light falls on the grating
(a) at right angles,
(b) at the angle 60∘ to the normal.
View SolutionFind the wavelength of monochromatic light falling normally on a diffraction grating with period d=2.2μm if the angle between the directions to the Fraunhofer maxima of the first and the second order is equal to Δθ=15∘.
View SolutionA plane light wave with wavelength λ falls normally on a phase diffraction grating whose side view is shown in Fig. The grating is cut on a glass plate with refractive index n. Find the depth h of the lines at which the intensity of the central Fraunhofer maximum is equal to zero. what is in this case the diffraction angle corresponding to the first maximum?
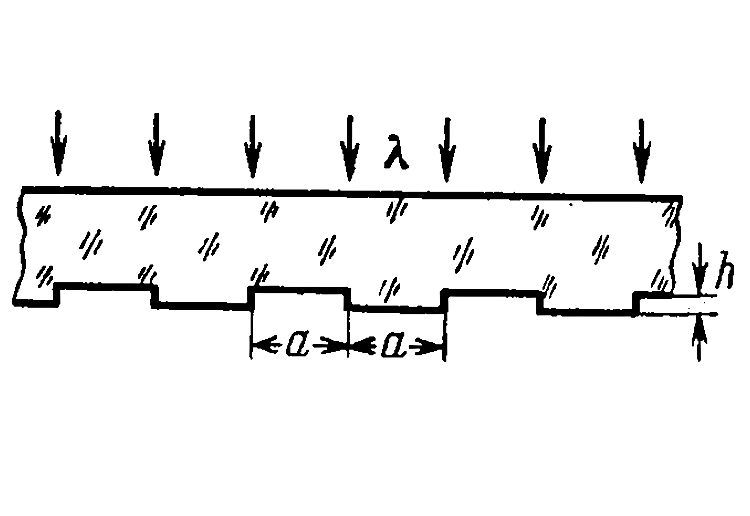
View SolutionA beam of monochromatic light of wavelength λ falls normally on a diffraction grating of line spacing d. If θ is the angle between the second-order diffracted beam and the direction of the incident light, what is the value of sinθ ?
View Solution
