A circular conducting coil of radius a and resistance R is placed with its plane perpendicular to a magnetic field. The magnetic field varies with time according to the equation B=B0sinωt. Obtain the expression for the induced current in the coil.Solution in Bengali
As we know, induced current,
I=induced emf(e)resistance (R)
Again, e=−dϕdt = rate of change of magnetic flux with time
∴I=−dϕdtR=−1R.ddt(BAcos0∘)
or, I=−AR.ddt(B0sinωt)[∵B=B0sinωt]
=−AωB0Rcosωt=−πa2ωB0Rcosωt
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - NCERTS TEXTBOOK QUESTIONS WITH ANSWER HINT
21 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - NCERT EXEMPLAR QUESTIONS WITH ANSWER HINT (MCQ-1)
5 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - SECTION RELATED QUESTIONS
29 Videos
- ELECTRIC POTENTIAL BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC POTENTIAL EXERCISE - CBSE Scanner
13 Videos
- ELECTROMAGNETIC WAVES BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVES EXERCISE - CBSE SCANNER
17 Videos
Similar Questions
Use Lenz's law to determine the direction of induced current in the situations described in the figure :
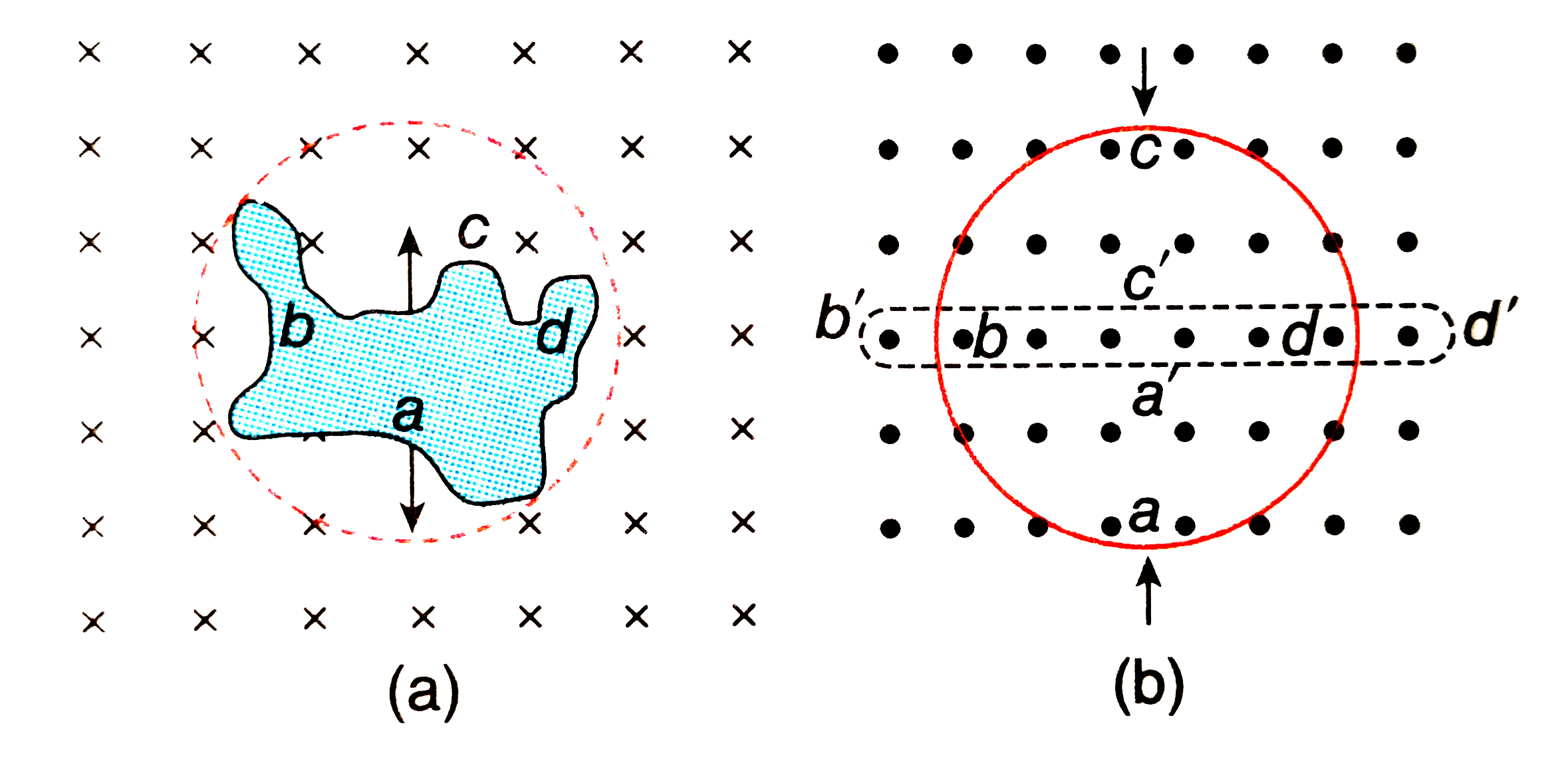
A wire of irregular shape turning into a circular shape.
View SolutionA cylinder bar magnet is rotated about its axis. A wire is connected from the axis and is made to touch the cylindrical surface through a contact. Then
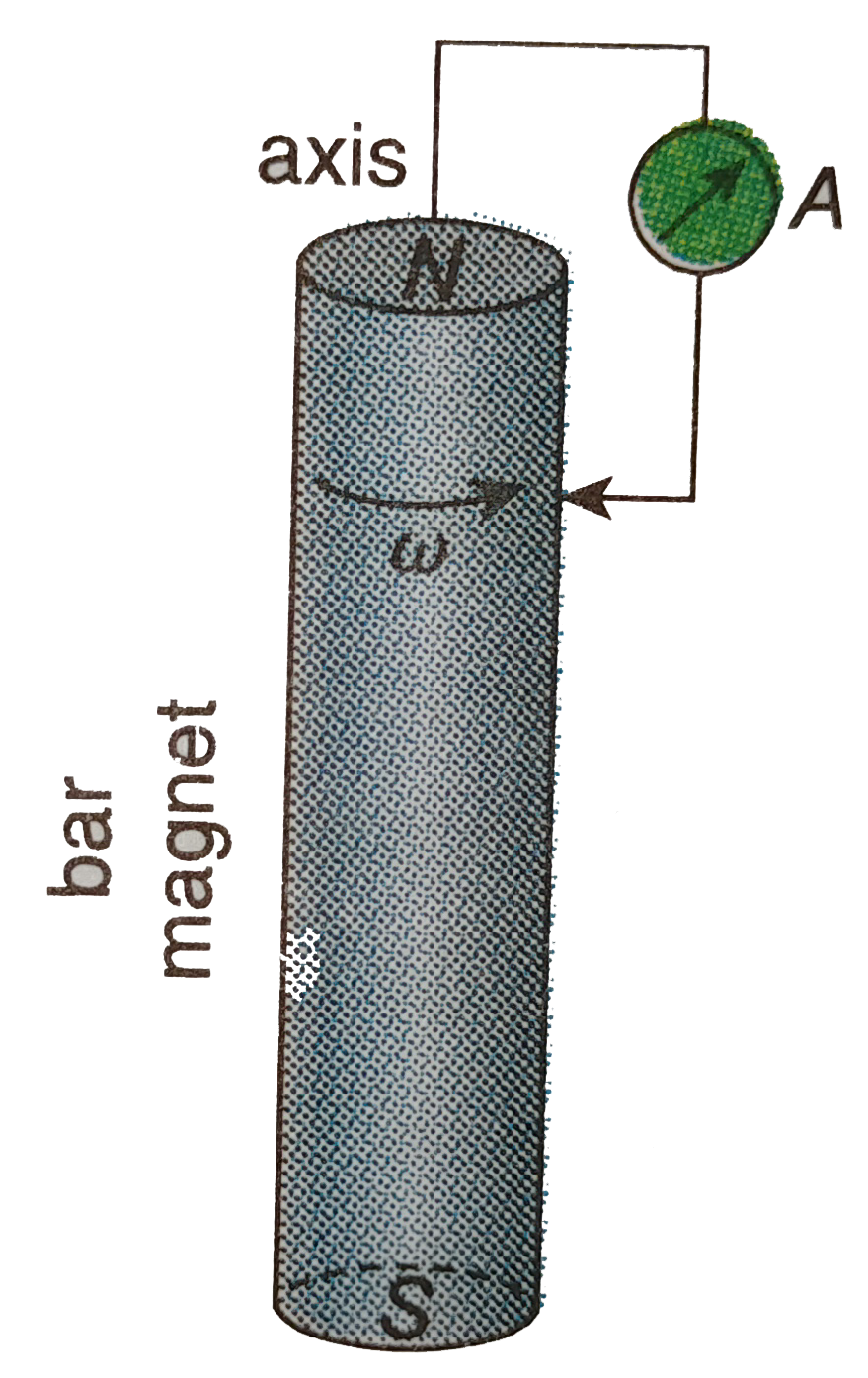
View SolutionWhat do you mean by electromagnetic induction?
View SolutionA test charge q is moved without acceleration along the path from A to B from B to C in electric field E as shown in fig.

(i) Calculate the potential difference between A and C.
View SolutionWhat are the directions of electric and magnetic field vectors relative to each other and relative to the direction of propagation of electromagnetic waves?
View SolutionA circular brass loop of radius a and resistance R is placed with it plane perpendicular to a magnetic field, which varies with time as B=B0sinωt. Obtain the expression for the induced current in the loop.
View SolutionA rectangular coil is placed in a region having a uniform magnetic field B perpendicular to the plane of the coil. An emf will not be induced ion the coil if the

View SolutionCurrent I is flowing through a circular coil of radius r. It is placed in a magnetic field B0 in such a way that the plane of the circular coil is perpendicular to B0. The force acting in it will be -
View SolutionA uniform magnetic field B=B_(0)that"i" in a region exists. A circular conducting loop of radius r and resistance R is placed with its plane in yz-plane. Determine the current through the loop and sense of the current.
View SolutionA conducting ring of radius r and resistance R is placed in region of uniform time varying magnetic field B which is perpendicular to the plane of the ring. It the magnetic field is changing at a rate α, then the current induced in the ring is
View Solutionएक वृत्ताकार धारावाही कुण्डली के केंद्र पर उत्पन्न चुम्बकीय क्षेत्र का मान B0 है। इसी कुण्डली के अक्षीय बिंदु पर, इसकी त्रिज्या के बराबर दूरी पर चुम्बकीय क्षेत्र B है तो B/B0 का मान होगा
View Solutionr त्रिज्या की धारावाही कुंडली के केंद्र पर चुंबकीय क्षेत्र B0 है। इस कुंडली के अक्ष पर उसके केंद्र से कितनी दूरी पर चुंबकीय क्षेत्र 127B0 होगा ?
View SolutionA circular coil of radius r is placed in a uniform magnetic field B. The magnetic field is normal to the plane of the coil is rotated at an angular speed of omega , about its own axis , then the induced emf in the coil is __
View SolutionA rectangular coil is placed in a region having a uniform magnetic field B perpendicular to the plane of the coil. An emf will not be induced ion the coil if the

View SolutionA current carrying loop of radius r carries a current I. It is placed in a magnetic field B0 such that the plane of the coil is perpendicular to the magnetic field. The net force on the coil is
View Solution
