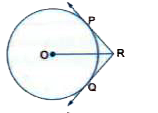
two tangents RQ and RP are drawn from an external point R to the circle with centre O. If ∠PRQ=120∘ , then prove that OR= PR+ RQ
Video Solution
Text Solution
In△ORQ
tan65=rQR
√3=rQR
QR=r√3
In△OPR
tan60=OPPR
√3=rRP
RP=r√3
OR=RP+RQ
RP+RQ=r√3+r√3=2r√3=OR
|
Similar Questions
Knowledge Check
In the given figure tangents PQ and PR are drawn from an external point P to a circle with centre O, such that ∠RPO=30∘. A chord RS is drawn parallel to the tangent PQ. Find ∠RQS.
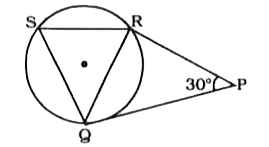 A30∘B50∘C45∘D60∘
A30∘B50∘C45∘D60∘2 equal tangents PA and PB are drawn from an external point P on a circle with centre O. What is the length of each tangent, if P Is 12 cm from the centre and the angle between the tangents is 120∘ ?
A24 cm.B6 cm.C8 cm.DCannot be determinedIf PQ and PR be the two tangents to a circle with centre O such that ∠QPR=120∘ then ∠POQ is
A90∘B45∘C30∘D60∘
Two tangents PQ and PR are drawn from an external point to a circle with centre 0. Prove that QORP is cyclic quadrileral.
View SolutionIn the figure , PQ and PR are tangents drawn from and external point P to the circle with centre O. Q and R are the points of contact. If PQ = 5 cm then what is the length of segment PR ? Why ?
View SolutionProve that the lengths of tangents drawn from an external point to a circle are equal.
View SolutionIf from an external point P of a circle with centre O, two tangents PQ and PR are drawn such ∠QPR=120∘, prove that 2PQ=PO.
View SolutionIn From an external point P, Two tangents PQ and PR are drawn to a circle of radius 4 cm with centre O . If ∠QPR=90∘ then length of PQ is
View Solution