A transverse sinusoidal wave of amplitude A, wavelength λ and frequency f is travelling along a stretch string. The maximum speed of any point on the string is v10, where, V is the velocity of wave propagation. If A=10−3m,andV=10ms−1, then λ and f are given by
λ=2π×10−2m,n=103Hz
λ=2πm,n1032πHz
λ=2π×10−2mandn=1032πHz
λ=π×10−2mandn=104Hz
The correct Answer is:C
For the transverse wave a=10−3mandv=10m/s
The maximum particle velocity vmax=aω
but vmax=v10
∴v10=a.2πn
∴1010=10−3×2πn ∴2πn=103orn=1032π
and v=nλ
∴λ=vn=10103/2π=2π×10−2m
Thus λ=2π×10−2mandm=103/2π
Topper's Solved these Questions
- WAVE MOTIONBOOK - MARVEL PUBLICATIONCHAPTER - WAVE MOTIONEXERCISE - Test your grasp
15 Videos
- SURFACE TENSION BOOK - MARVEL PUBLICATIONCHAPTER - SURFACE TENSION EXERCISE - MULTIPLE CHOICE QUESTIONS . (TEST YOUR GRASP )
10 Videos
- WAVE THEORY OF LIGHT AND POLARISATION BOOK - MARVEL PUBLICATIONCHAPTER - WAVE THEORY OF LIGHT AND POLARISATION EXERCISE - TEST YOUR GRASP -10
15 Videos
Similar Questions
A sinusoidal transverse wave of amplitude 10−2 m, wavelength λ, frequency f is travelling with speed (V = 20 m/s) on a stretched string. If the maximum speed at any point of the string is V5 then find the frequency and wavelength of the wave.
View SolutionA transverse wave of amplitude 2 m, wavelength 4 m and frequency 6 Hz is propagating in a string toward positive y-direction. The equation of the wave may be (y is in m and t is in s)
View Solution
Knowledge Check
A transverse sinusoidal wave of amplitude a, wavelength λ and frequency f is travelling on a stretched string. The maximum speed of any point in the string is v/10, where v is the speed of propagation if the wave. If a=10−3m and v=10ms−1, then lanbda and f are given by
A(a) λ=2π×10−2mB(b) λ=10−3mC( c ) f=103Hz/(2π)D(d) f=104HzA transverse wave, whose amplitude is 0.5 m wavelength is 1 m and frequency is 2Hz. is travelling along positive x direction. The equation of this wave will be-
Ay=0.5cos(2πx−4πt)By=0.5cos(2πx−4πt)Cy=0.5sin(πx−2πt)Dy=0.5cos(2πx−2πt)A transverse wave of amplitude 0.5m and wavelength 1 m and frequency 2 Hz is propagating in a string in the negative x - direction. The expression for this wave is [
Ay(x,t)=0.5sin(2πx−4πt)By(x,t)=0.5cos(2πx+4πt)Cy(x,t)=0.5sin(πx+2πt)Dy(x,t)=0.5cos(2πx+2πt)
The speed of a sound wave depends on its wavelength λ, and frequency v. Find an expression for the spped of sound.
View SolutionA transverse wave of amplitude 0.5m and wavelength 1 m and frequency 2 Hz is propagating in a string in the negative x - direction. The expression for this wave is
View SolutionThe equation of a wave of amplitude 0.02 m and period 0.04 s travelling along a stretched string with a velocity of 25m/s will be
View SolutionA transverse sinusoidal wave of wavelength 20 cm is moving along a string towards increasing x. the transverse displacement of the string particle at x=0 as a function of time is shown in figure.
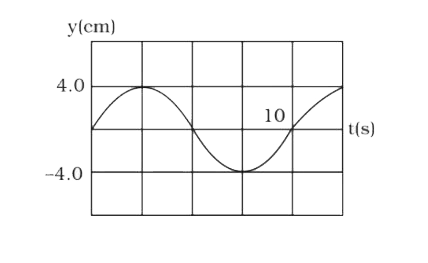
Q. The velocity of propagation of the wave is ?
View SolutionTwo identical sinusoidal waves each of amplitude 10 mm with a phase difference of 90∘ are travelling in the same direction in a string. The amplitude of the resultant wave is
View Solution
