A line meets the coordinate axes at A and B. A circle is circumscribed obout the triangle OAB. If the distance of the points A and B from the tangent at O, the origin, to the circle are m and n respectively, find the diameter of the circle.
2m+n2
(m+n)
mnm+n
m+2n2
The correct Answer is:B
Step by step video, text & image solution for A line meets the coordinate axes at A and B. A circle is circumscribed obout the triangle OAB. If the distance of the points A and B from the tangent at O, the origin, to the circle are m and n respectively, find the diameter of the circle. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ARIHANT MATHSCHAPTER - CIRCLE EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)16videos
CIRCLE
- BOOK - ARIHANT MATHSCHAPTER - CIRCLE EXERCISE - Exercise (Statement I And Ii Type Questions)8videos
CIRCLE
- BOOK - ARIHANT MATHSCHAPTER - BIONOMIAL THEOREMEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)21videos
BIONOMIAL THEOREM
- BOOK - ARIHANT MATHSCHAPTER - COMPLEX NUMBERSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)43videos
COMPLEX NUMBERS
Similar Questions
A line meets the coordinate axes at A and B . A circle is circumscribed about the triangle OAB. If d1andd2 are distances of the tangents to the circle at the origin O from the points AandB , respectively, then the diameter of the circle is 2d1+d22 (b) d1+2d22 d1+d2 (d) d1d2d1+d2
View SolutionPoint O is the centre of a circle . Line a and line b are parallel tangents to the circle at P and Q. Prove that segment PQ is a diameter of the circle.
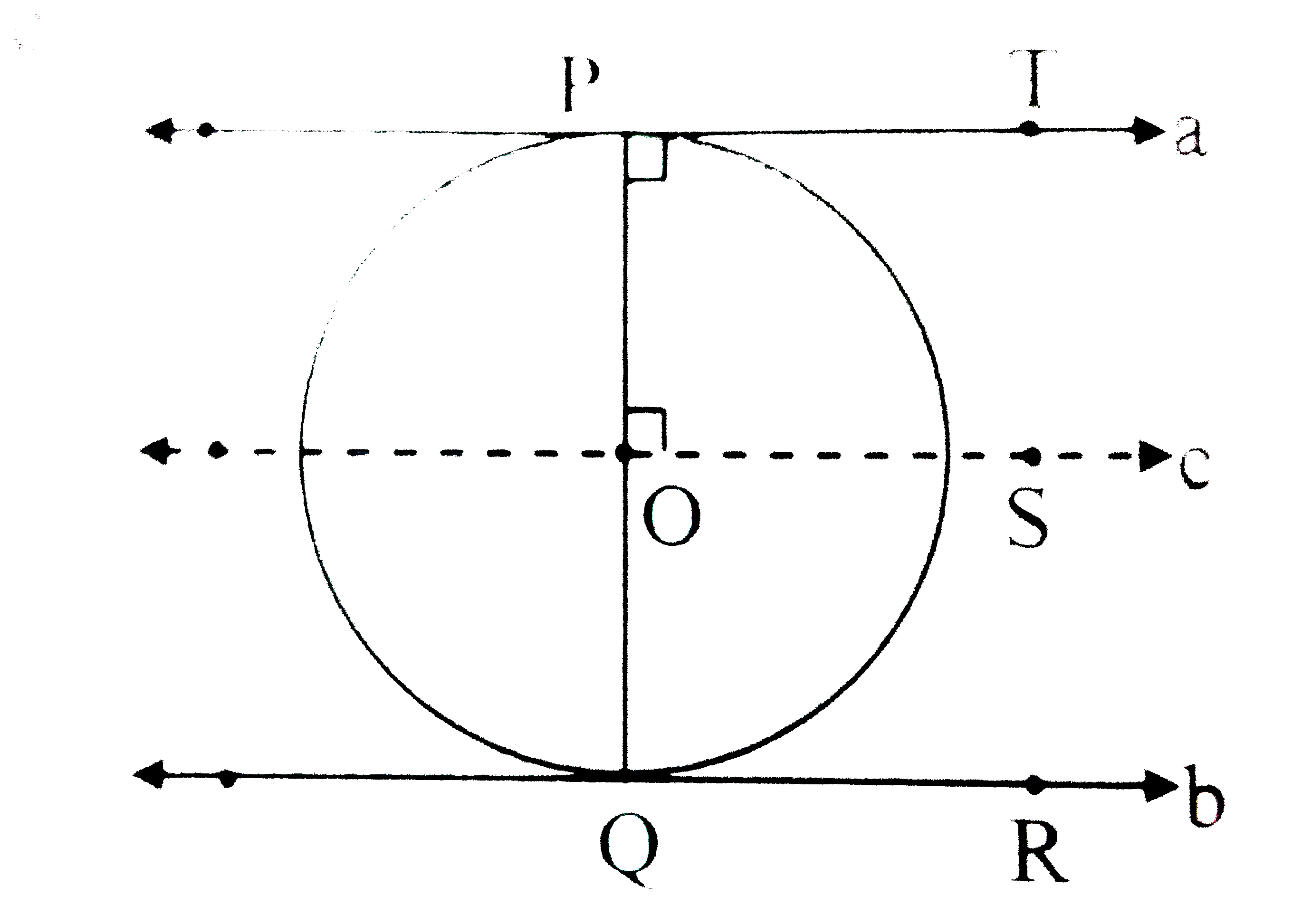 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A line meets the co-ordinates axes at A(a, 0) and B(0, b) A circle is circumscribed about the triangle OAB. If the distance of the points A and B from the tangent at origin to the circle are 3 and 4 repectively, then the value of a2+b2+1 is
A20B30C40D50 - Question 2 - Select One
Aline meets the co-ordinate axes in A and B.A circle is circumscribed about the triangle OAB. The distances from the end points of the side AB to the line touching the circle at the origin O are equal to pand g respectively. The diameter of the circle is
Ap(p+q)Balp+q)Cp+qD12(p+q) - Question 3 - Select One
A line makes equal intercepts of length a on the coordinate axes. A circle is circumscribed about the triangle which the line makes with the coordinate axes. The sum of the distance of the vertices of the triangle from the tangent to this circle at the origin is
Aa/√2BaCa√2Da+√2
A circle makes intercepts of length 2a and 3b on the coordinates axes. The equation ofthe tangent from the origin to the circle is
View SolutionPA and PB are tangents to the circle with centre O from an external point P, touching the circle at A and B respectively. Show that the quadrilateral AOBP is cyclic.
View SolutionThe area of the triangle formed by the tangent at the point (a,b) to the circle x2+y2=r2 and the co-ordinate axes is:
View SolutionThe straight line x + 2y = 1 meets the coordinate axes at A and B. A circle is drawn through A,B and the origin. Then, the sum of perpendicular distances from A and B on the tangent to the circle at the origin is
View SolutionThe area of the triangle formed by the tangent at the point (a, b) to the circle x2+y2=r2 and the coordinate axes, is
View Solution

