For a random variable X, if Var (X) = 4 and E(X2)=13, the value of E(X) is
3
4
5
6
The correct Answer is:A
To find the value of E(X) given that Var(X)=4 and E(X2)=13, we can use the relationship between variance, expected value, and the expected value of the square of a random variable.
1. Recall the formula for variance:
The variance of a random variable X is given by the formula:
Var(X)=E(X2)−(E(X))2
2. Substitute the known values into the formula:
We know that Var(X)=4 and E(X2)=13. Plugging these values into the variance formula gives:
4=13−(E(X))2
3. Rearrange the equation to solve for (E(X))2:
Rearranging the equation, we get:
(E(X))2=13−4
(E(X))2=9
4. Take the square root to find E(X):
To find E(X), take the square root of both sides:
E(X)=√9
E(X)=3
Thus, the value of E(X) is 3.
The variance of a random variable X is given by the formula:
Var(X)=E(X2)−(E(X))2
We know that Var(X)=4 and E(X2)=13. Plugging these values into the variance formula gives:
4=13−(E(X))2
Rearranging the equation, we get:
(E(X))2=13−4
(E(X))2=9
To find E(X), take the square root of both sides:
E(X)=√9
E(X)=3
Topper's Solved these Questions
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Critical Thinking
33 Videos
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Competitive Thinking
9 Videos
- PLANEBOOK - TARGET PUBLICATIONCHAPTER - PLANEEXERCISE - EVALUATION TEST
14 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - TARGET PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - Evaluation Test
9 Videos
Similar Questions
Knowledge Check
For a random variable X, E(X)=3andE(X2)=11. Then, variance X is
A8B5C2D1For a random variable X, E(X)=3 and E(X2)=11 Then, variance of X is
A8B5C2D1For a random variable X, if E(X) = 5 and V(X) = 6, then E(X2) is equal to
A19B31C61D11
The probability distribution of the random variable X is given by
Then, the value of V(X) is equal to
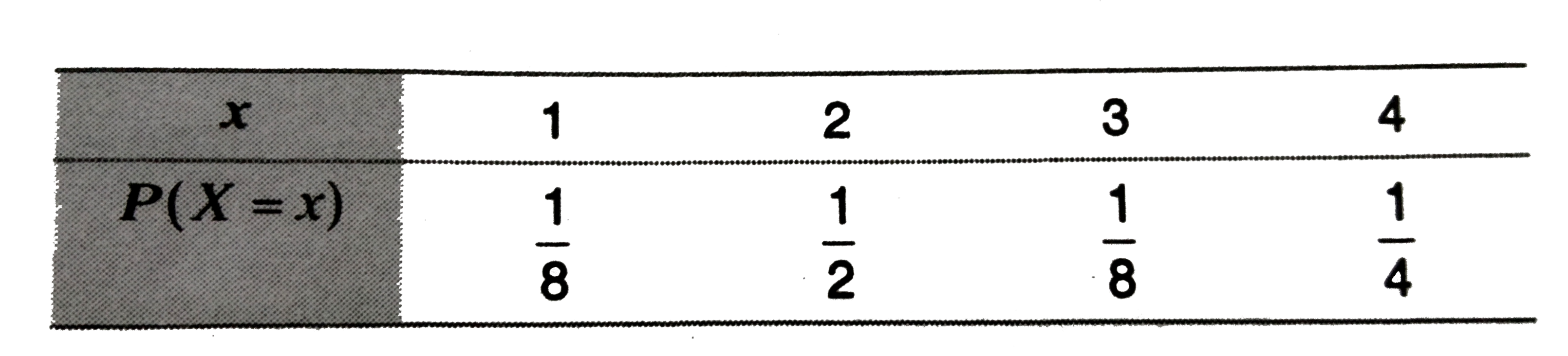
View SolutionFor the random variable X , if V(X) = 4 , E(X) = 3 , then E(X2) is …
View SolutionThe probability distribution of a random variable X is given as follows:
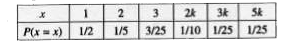
If E(X)=2.3 , then value of k is
View SolutionFor a random variable X, if E(X2) = 31 , Var(X) = 6, then E(X)=.........
View SolutionIf the probability mass function of a discrete random variable X is P(x)=Cx3,x=1,2,3=0, otherwise. Then, E(X) is equal to
View Solution
