Mass M is split into two parts m and (M−m), which are then separated by a certain distance. What is the ratio of (m/M) which maximises the gravitational force between the parts ?
To find the ratio of mM that maximizes the gravitational force between two parts of mass M split into m and (M−m), we can follow these steps:
Step 1: Write the expression for gravitational force
The gravitational force F between two masses m and (M−m) separated by a distance R is given by Newton's law of gravitation:
F=G⋅m⋅(M−m)R2
where G is the gravitational constant.
Step 2: Differentiate the force with respect to m
To find the maximum force, we need to differentiate F with respect to m and set the derivative equal to zero:
dFdm=GR2⋅((M−m)−m)=GR2⋅(M−2m)
Step 3: Set the derivative equal to zero
Setting the derivative equal to zero gives us:
M−2m=0
Step 4: Solve for m
From the equation M−2m=0, we can solve for m:
2m=M⟹m=M2
Step 5: Find the ratio mM
Now, we find the ratio of m to M:
mM=M2M=12
Conclusion
Thus, the ratio mM that maximizes the gravitational force between the two parts is:
mM=12
---
The gravitational force F between two masses m and (M−m) separated by a distance R is given by Newton's law of gravitation:
To find the maximum force, we need to differentiate F with respect to m and set the derivative equal to zero:
Setting the derivative equal to zero gives us:
From the equation M−2m=0, we can solve for m:
Now, we find the ratio of m to M:
Thus, the ratio mM that maximizes the gravitational force between the two parts is:
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - C.U.Q220videos
GRAVITATION
- BOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - LEVEL I(C.W.)51videos
GRAVITATION
- BOOK - NARAYNACHAPTER - FRICTIONEXERCISE - Passage type of questions I6videos
FRICTION
- BOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - LEVEL-III(C.W)52videos
KINETIC THEORY OF GASES
Similar Questions
A mass M is split into two parts m and (M-m) which are then separated by certain distance. Find ratio (m/M) to maximise the gravitational force F=Gm(M−m)r2 between the parts.
Here G = gravitational constant and r is the distance between m and (M-m).View SolutionMass M is divided into two parts m1, and m2, For a given separation the ratio of m1M for which the gravitational attraction between the two parts becomes maximum is
View Solution
Knowledge Check
- Question 1 - Select One
A mass M splits into two parts m and (M - m) , which are then separated by a certain distance. Wha ratio (m/M) maximize the gravitational force between the path ?
A23B34C12D13 - Question 2 - Select One
A mass M splits into two parts m and (M-m), which are separated by a cetain distance. The ratio m/M which maximizes the gravitational force between the parts is
A1:4B1:3C1:2D1:1 - Question 3 - Select One
A mass M at rest is broken into two pieces having masses m and (M-m). The two masses are then separated by a distance r. The gravitational force between them will be maximum when the ratio of the masses [m : (M-m) of the two parts is
A1:1B1:2C1:3D1:4
A body of mass (2M) splits into four masses {m,M−m,m,M−m}, which are rearranged to form a square as shown in the figure. The ratio of Mm for which, the gravitational potential energy of the system becomes maximum is x : 1 . The value of x is ________.
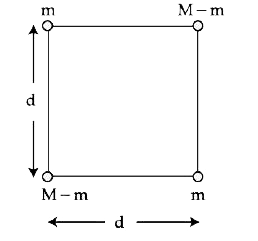 View Solution
View SolutionTwo spheres of masses (m) and (M - m) are prepared from a sphere of mass M. They are kept at a distance x. For what ratio of mM will the gravitational attraction between them be maximum ?
View SolutionTwo point masses m and M are separated by a distance L . The distance of the centre of mass of the system from m is
View SolutionA particle of mass m is located inside a spherical shell of mass M and radius R. The gravitational force of attraction between them is
View SolutionTwo bodies of masses M1 and M2 are kept separeated by a distance d. The potential at the point where the gravitational field produced by them is zero, is :-
View Solution

