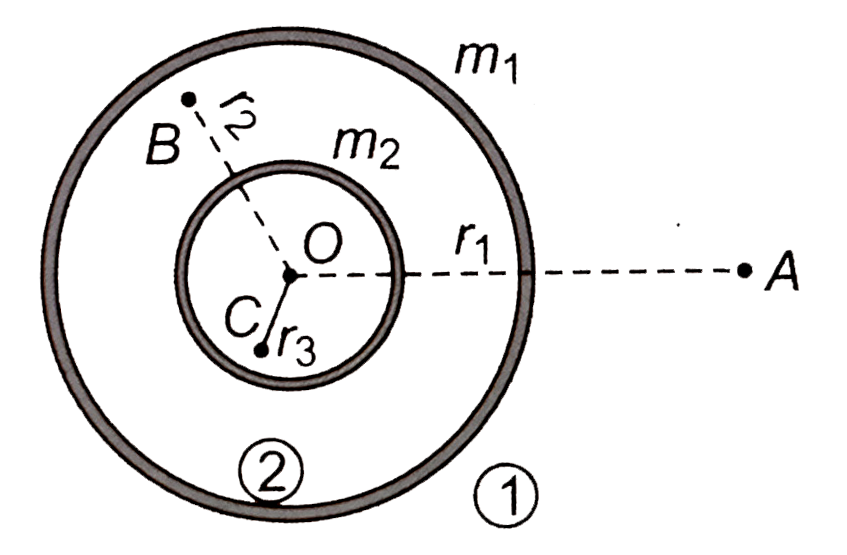
Two concnetric shells of different masses m1 and m2 are having a sliding particle of mass m. The forces on the particle at position I, II and II are

0,Gm1r22,G(m1+m2)mr21
Gm2r22,0,Gm1r21
G(m1+m2)mr21,Gm2r22,0
G(m1+m2)mr21,G(m2)mr22,0
The correct Answer is:D
Positoni I, F=Gm(m1+m2)r21
(here the particle lies outside of both the shells)
Positon II. F=Gm2r22
Here the particle lies outside of the shell of mass m1 positon III. Her the particle lies inside of both of th shells of F=0.
Topper's Solved these Questions
Similar Questions
Two concentric shells of mass m1 and m2 are situated as shown. Find the force on a particle of mass m when the particles is located at (a)r=r1,(b)r=r2,(c)r=r3. The distance r is measured from the centre of the shell.
View SolutionConsider a system of two particles having masses m1 and m2. If the particle of mass m1 is pushed towards the centre of mass of particles through a distance d, by what distance would the particle of mass m2 move so as to keep the mass centre of particles at the original position?
View Solution
Knowledge Check
Two concentric shells of different mass m1 and m2 are having a sliding particle of mass m. The forces on the sliding at positions I,II and III are respectively
 A0,Gm1r22,G(m1+m2)mr21BGm2r22,0,Gm1r21CG(m1+m2)mr21,Gm2r22,0DG(m1+m2)mr21,G(m2)mr22,0
A0,Gm1r22,G(m1+m2)mr21BGm2r22,0,Gm1r21CG(m1+m2)mr21,Gm2r22,0DG(m1+m2)mr21,G(m2)mr22,0The reduce mass of two particles having masses m and 2 m is
A2 mB3 mC2 m/3Dm/2The reduce mass of two particles having masses m and 2 m is
A2 mB3 mC2 m/3Dm/2
Consider a sytem of two particles having masses m1 and m2. If the particle of mass m1 is pushed towards the centre of mass of particles through a distance d, by what distance would the particle of mass m2 move so as to keep the mass centre of particles at the original position?
View SolutionTwo particles of mass m1andm2 are in space at separation →r [vector from m(1) to m2]. The only force that the two particles experience is the mutual gravitational pull. The force applied by m1onm2is→F. Prove that μd2→rdt2=→F where μm1m2m1+m2 is known as reduced mass for the two particle system .

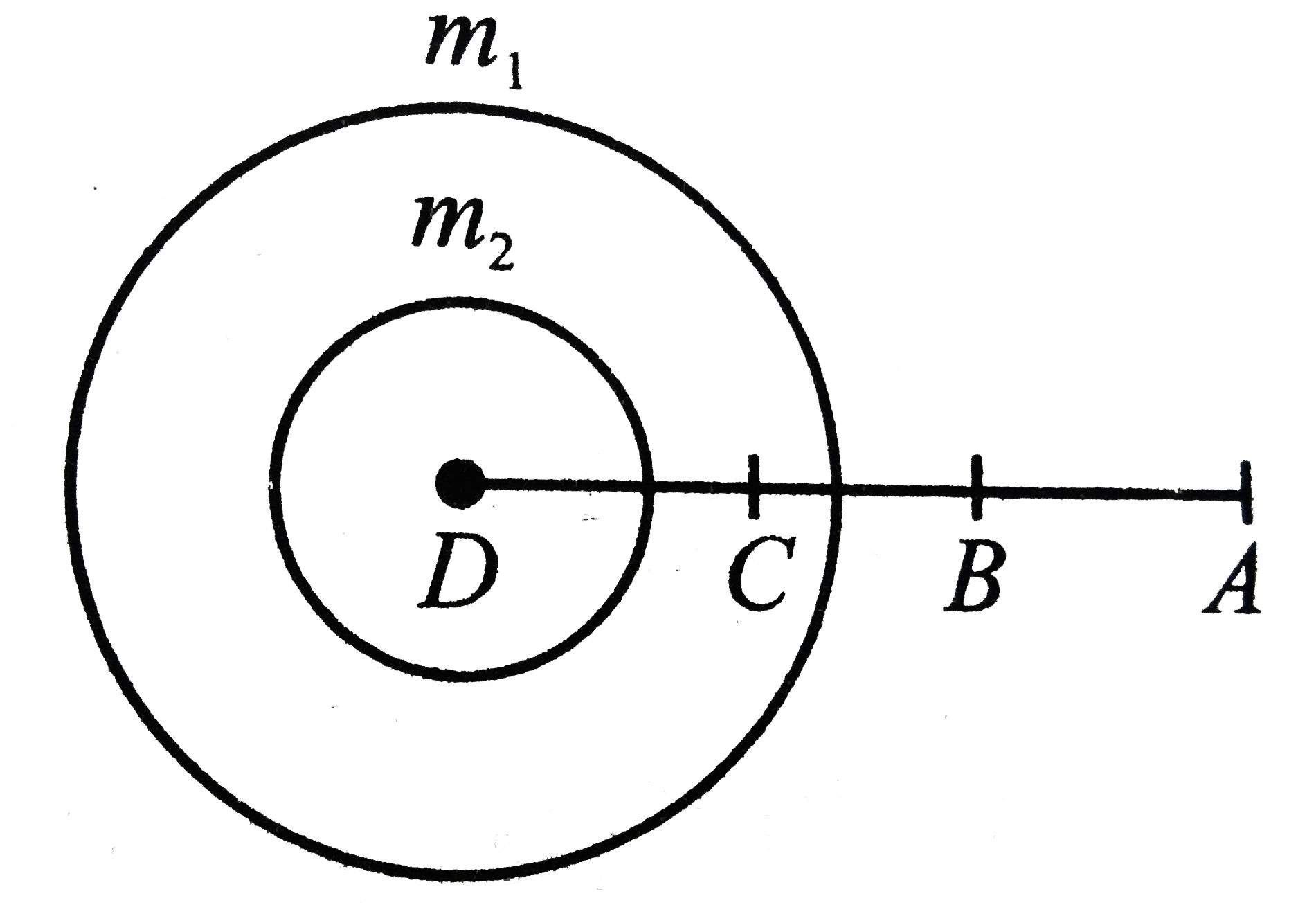
View SolutionFigure shows two shells of masses m1 and m2. The shells are concentric. At which point, a particle of mass m shall experience zero force?
View SolutionConsider a system of two particles having masses m1 and m2. If the particle of mass m1 is pushed towards the centre of mass of particles through a distance d, by what distance would the particle of mass m2 move so as to keep the mass centre of particles at the original position?
View SolutionFigure shows two shells of masses m1 and m2. The shells are concentric. At which point, a particle of mass m shall experience zero force?

View Solution
