A rod AB of mass 3m and length 4a is falling freely in a horizontal position and c is a point distance a from A. When the speed of the rod is u, the point c collides with a particle of mass m which is moving vertically upwards with speed u. if the impact between the particle and the rod is perfectely elastic find
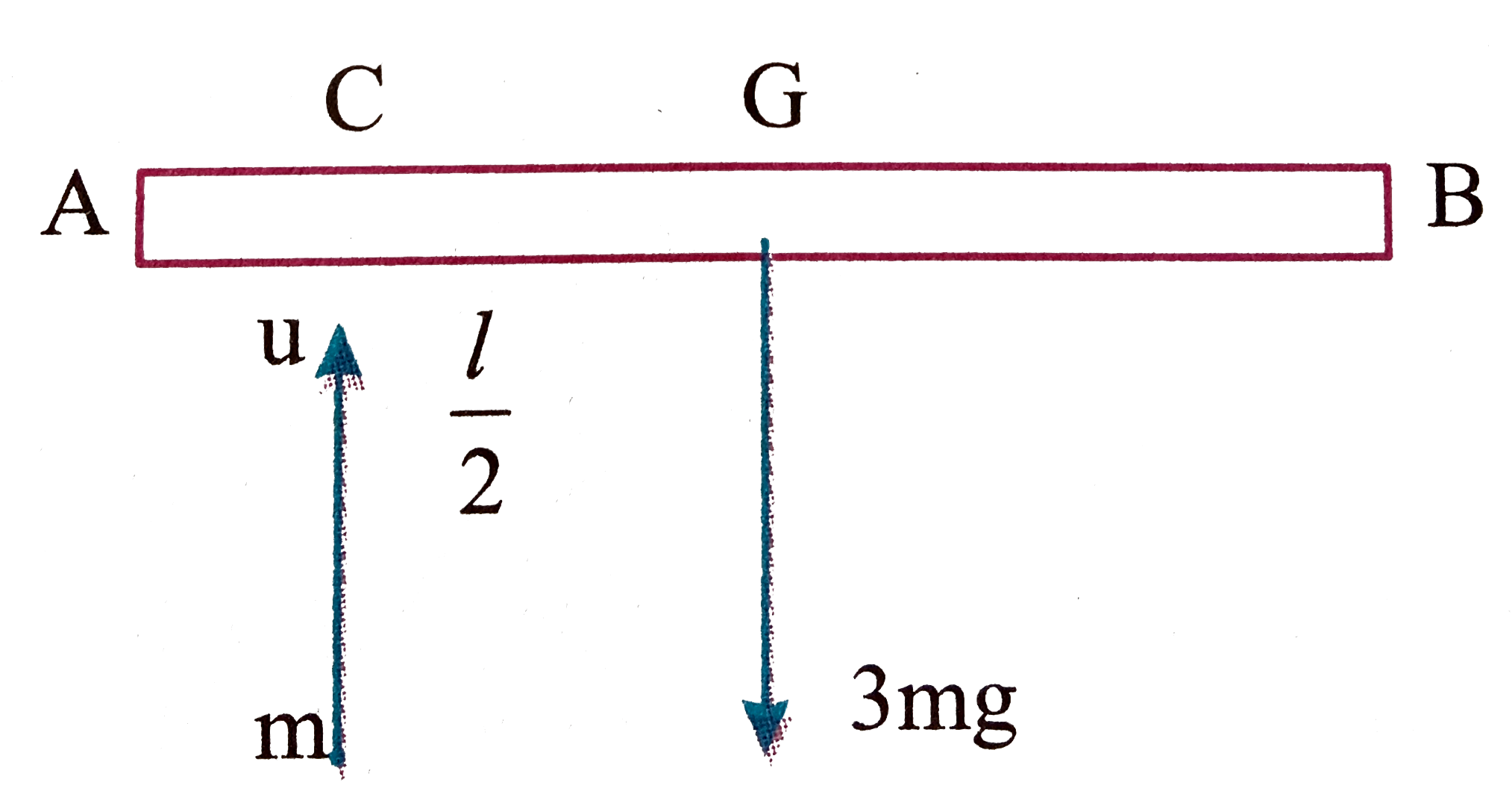
The angular velocity of the rod immediately after the impact
19u12a
12u19a
29u19a
19u29a
The correct Answer is:B
In order to use the law of restitution. We need the speed of point C, which is u1−aω (down wards)
law of restitution now given relative velocity of separation at point of impact =e(relative velcity of approch) or u2−(u1−aω)=e(u+u)
hence 2u=u2−u1+aω
For the rod the speed of the particle is 2919υ (down wards)
Topper's Solved these Questions
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-V
72 Videos
- PHYSICAL WORLDBOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q
10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - IV
39 Videos
Similar Questions
A rod of mass M and length L is falling vertically with speed v. Suddenly its one end gets stuck in a friciitonless hook. Find the angular velocity of the rod just after its end gets struck.

View SolutionA rod of mass M and length L is kept on a frictionless horizontal floor. A particle of mass m moving perpendicular to the rod with a speed v strikes at the end of the rod and sticks there. Calculate angular velocity acquired by the combined system.
View Solution
Knowledge Check
A rod AB of mass 3m and length 4a is falling freely in a horizontal position and c is a point distance a from A. When the speed of the rod is u, the point c collides with a particle of mass m which is moving vertically upwards with speed u. if the impact between the particle and the rod is perfectely elastic find
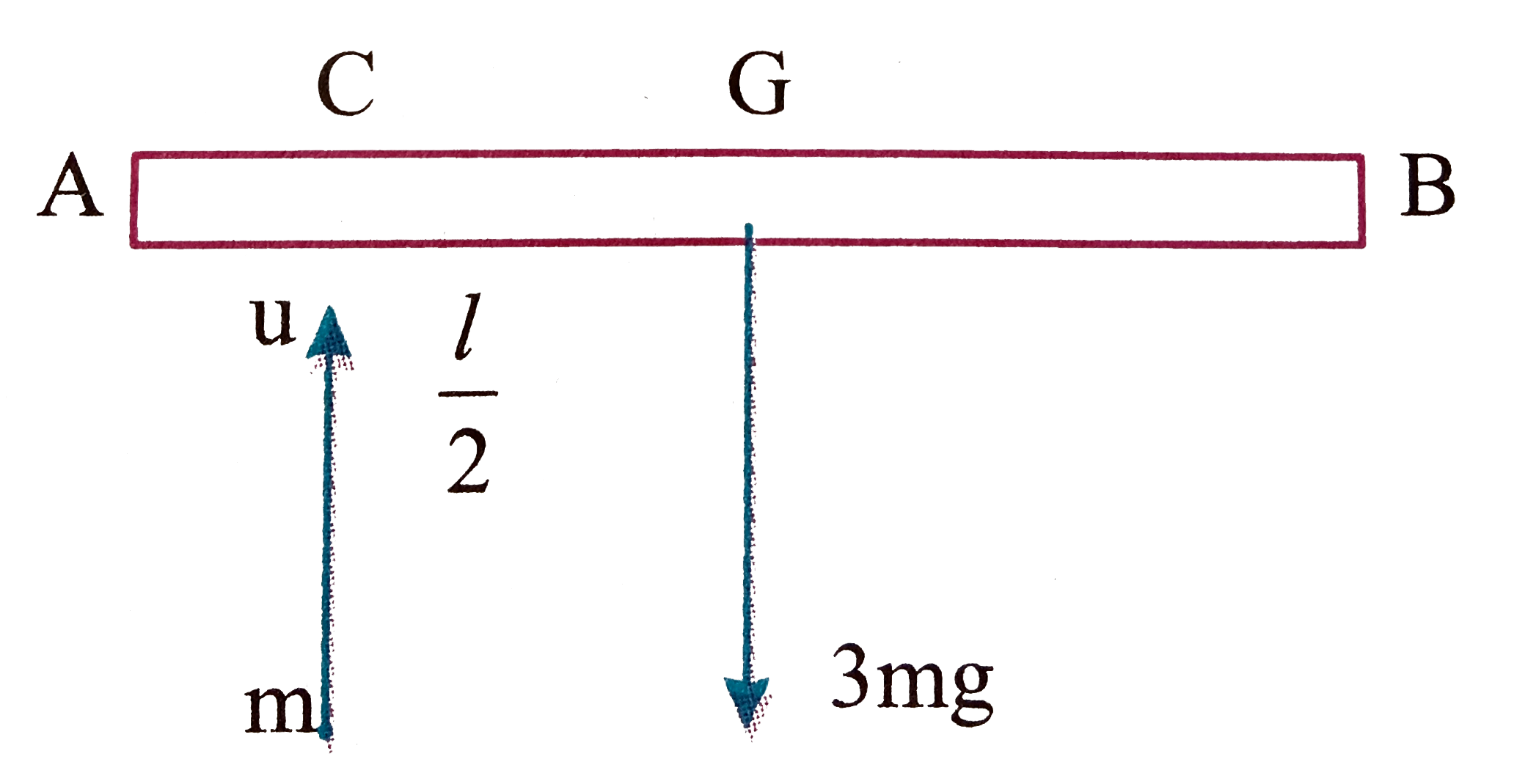
The velocity of the particle immediately after the impactA2919udownB1929udownC2919,uupD2719udownA rod AB of mass 3m and length 4a is falling freely in a horizontal position and c is a point distance a from A. When the speed of the rod is u, the point c collides with a particle of mass m which is moving vertically upwards with speed u. if the impact between the particle and the rod is perfectely elastic find
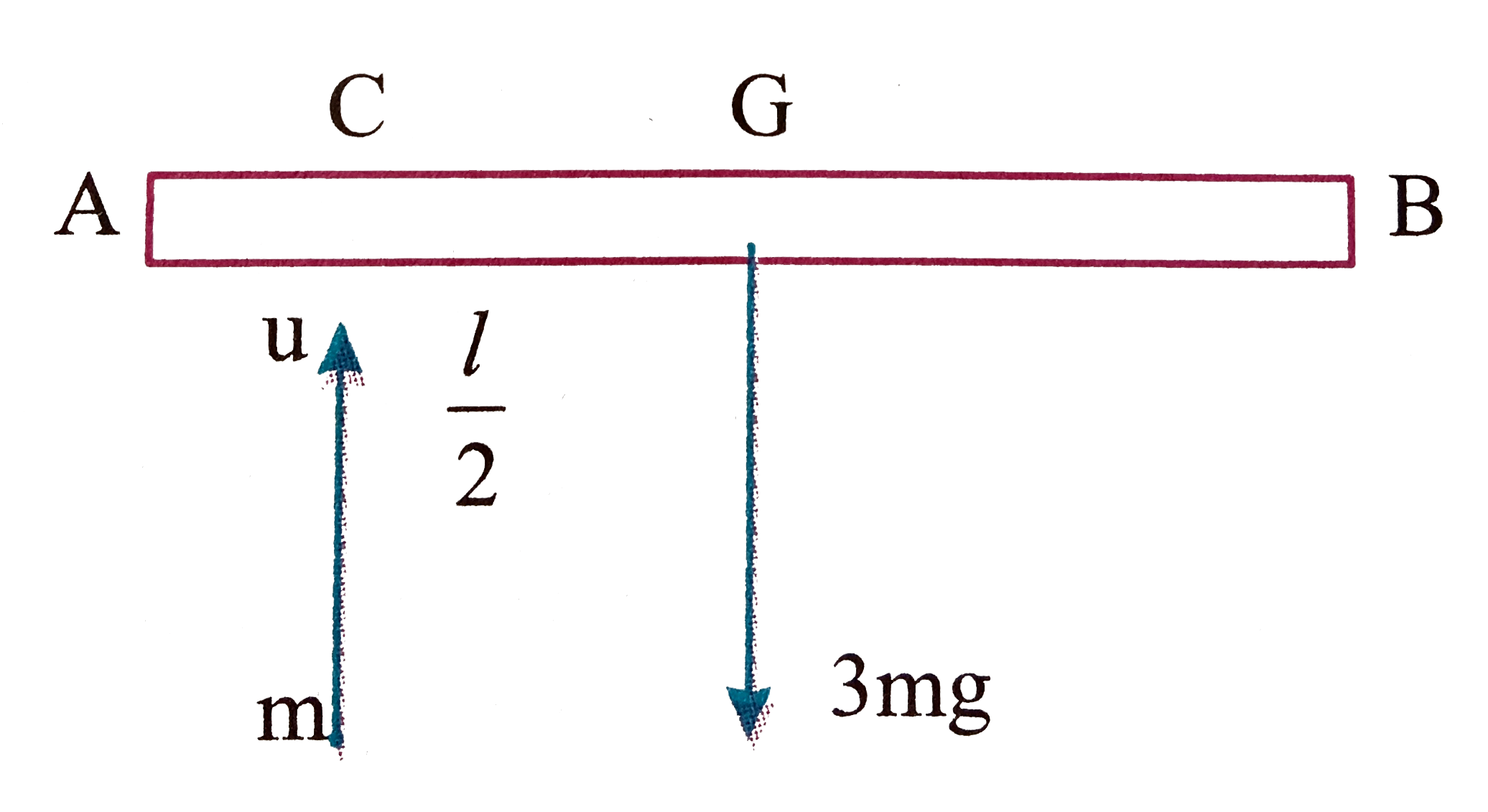
The speed of B immediately after the impact isA1927udownB1927uupC2719udownD2719uupA uniform rod AB of mass m and length 2a is falling freely without rotationb under gravity with AB horizontal. Suddenly the end A is fixed when the speed of the rod is v. The angular speed which the rod begains to rotate is
Av2aB4v3aCv3aD3v4a
A rod AB of mass m and length L is rotating in horizontal plane about one of its ends which is pivoted. The constant angular speed with which the rod is rotating is ω. The force applied on the rod by the pivot is
View SolutionA uniform rod AB of mass 3m and length 2l is lying at rest on a smooth horizontal table with a smooth vertical axis through the end A. A particle of mass 2m moves with speed 2u across the table and strikes the rod at its mid-point C if the impact is perfectly elastic. Find the speed of the particle after impact if
(a). It strikes rod normally,
(b). Its path before impact was inclinded at 60∘ to AC.
View SolutionA uniform rod AB of mass m length 2a is allowed to fall under gravity with AB in horizontal. When the speed of the rod is v suddenly the end A is fixed. Find the angular velocity with which it begins to rotate.
View SolutionA rod AB of mass M and length L is lying on a horizontal frictionless surface. A particle of mass m travelling along the surface hits the end A of the rod with a velocity v0 in a direction perpendicular to AB. The collision in elastic. After the collision the particle comes to rest
(a). Find the ratio m/M
(b). A point P on the rod is at rest immediately after collision find the distance AP.
(c). Fid the linear speed of the point P a time πL/3v0 after the collision.
View Solution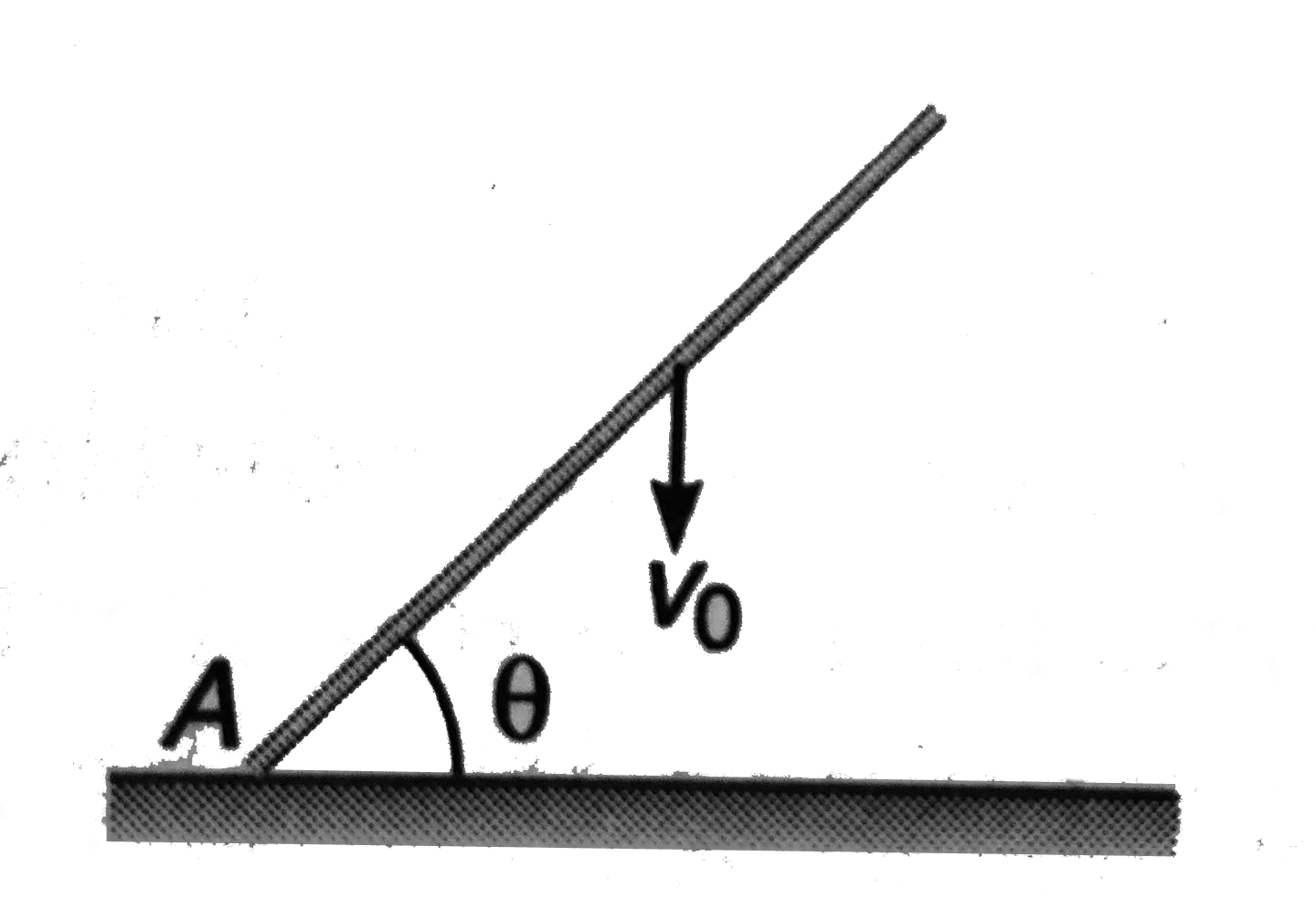
A rod of length l forming an angle θ with the horizontal strikes a frictionless floor at A with its centre of mass velocity v0 and no angular velocity. Assuming that the impact at A is perfectly elastic. Find the angular velocity of the rod immediately after the impact.
View Solution
