Question

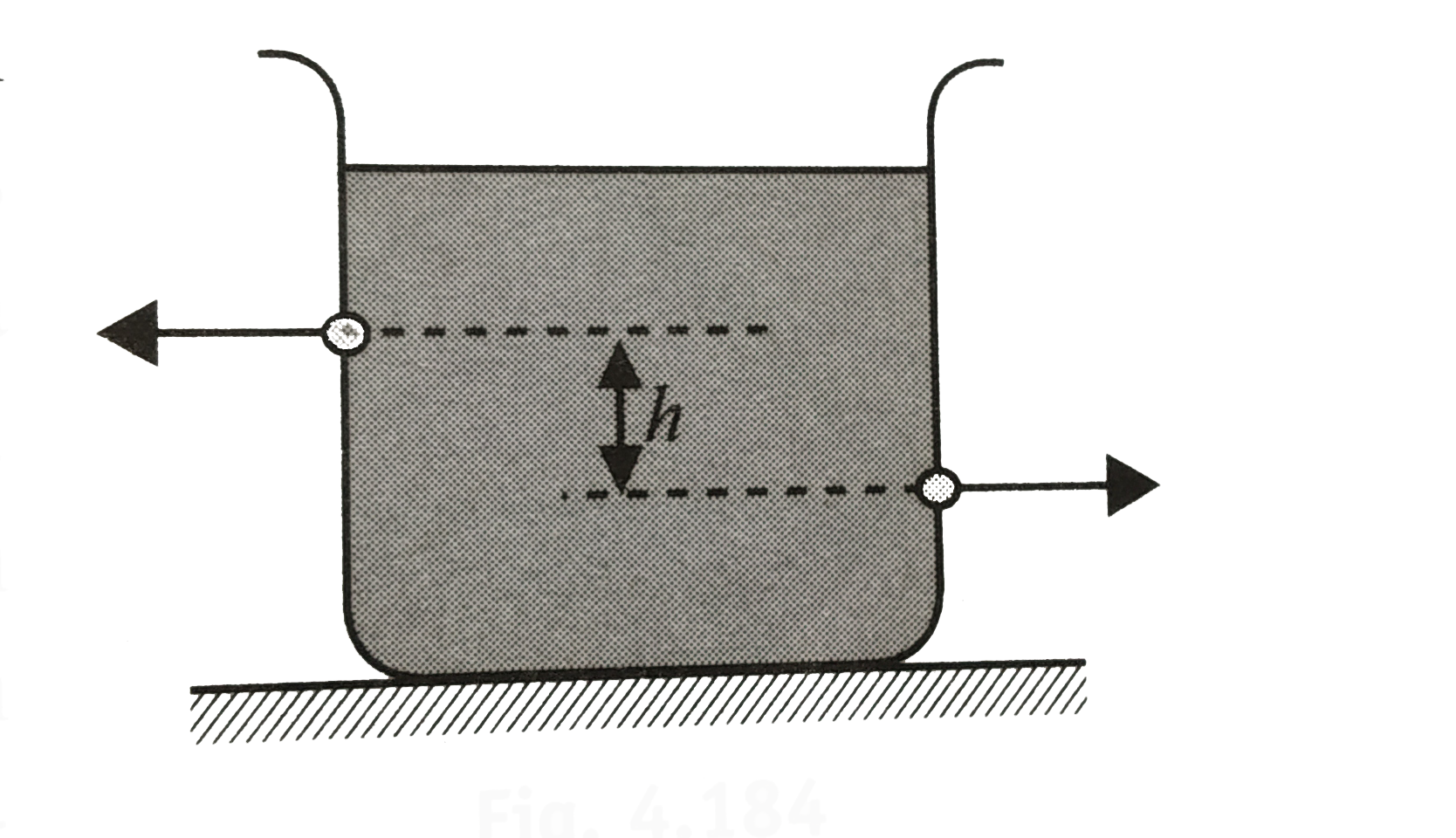
There are two holes one each along the opposite sides of a wide rectangular tak. The cross section of each hole is $0.01m^2$ and the vertical distance between the holes is one meter. The tak is filled with water. The net force on the tak in newton when water flows out of the holes is (density of water $1000kg/m^3)$

Options

A. 100
B. 200
C. 300
D. 400

Answer

B

Explanation

To solve the problem, we need to calculate the net force acting on the tank when water flows out of the two holes. We will follow these steps:

Step 1: Identify the velocities of efflux
The velocity of efflux from each hole can be calculated using Torricelli's theorem, which states that the velocity of fluid flowing out of an orifice under the influence of gravity is given by:

\( v = \sqrt{2gh} \)

For the hole at the top (let's call it Hole 1), the height \( h \) is 1 meter (the vertical distance to the water surface). Thus, the velocity \( v_1 \) is:

\( v_1 = \sqrt{2g \cdot 1} = \sqrt{2g} \)

For the hole at the bottom (let's call it Hole 2), the height \( h \) is 0 meters (as it is at the water level). Thus, the velocity \( v_2 \) is:

\( v_2 = \sqrt{2g \cdot 0} = 0 \)

Step 2: Calculate the flow rates
The flow rate \( Q \) through each hole can be calculated using the formula:

\( Q = A \cdot v \)

where \( A \) is the cross-sectional area of the hole.

For Hole 1:

\( Q_1 = A_1 \cdot v_1 = 0.01 \cdot \sqrt{2g} \)

For Hole 2:

\( Q_2 = A_2 \cdot v_2 = 0.01 \cdot 0 = 0 \)

Step 3: Calculate the forces due to the efflux
The force exerted by the water flowing out of each hole can be calculated using the rate of change of momentum:

\( F = \rho \cdot Q \cdot v \)

For Hole 1:

\( F_1 = \rho \cdot Q_1 \cdot v_1 = 1000 \cdot (0.01 \cdot \sqrt{2g}) \cdot \sqrt{2g} = 1000 \cdot 0.01 \cdot 2g = 20g \)

For Hole 2:

Since \( Q_2 = 0 \), the force \( F_2 \) is:

\( F_2 = 0 \)

Step 4: Calculate the net force on the tank
The net force \( F_{net} \) on the tank is the difference between the forces exerted by the water flowing out of the two holes:

\( F_{net} = F_2 - F_1 = 0 - 20g \)

Using \( g \approx 10 \, \text{m/s}^2 \):

\( F_{net} = -20 \cdot 10 = -200 \, \text{N} \)

The negative sign indicates that the force is acting in the upward direction.

Final Answer
The net force on the tank when water flows out of the holes is:

\( \text{Net Force} = 200 \, \text{N} \)