If C is the mid-point of AB and P is any point outside AB, then
A
PA + PB = PC
B
PA + PB + 2 PC = 0
C
PA + PB - 2PC = 0
D
PA + PB + PC = 0
Text Solution
The correct Answer is:C
By the properties of mid-point theorem,
PA + PB = 2 PC
|
Topper's Solved these Questions
- VECTORS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - VECTORS EXERCISE - (VECTORS ) Exercise 2 ( Topical problems )
88 Videos
- VECTORS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - VECTORS EXERCISE - ( MHT CET Corner)
66 Videos
- TRIGONOMETRIC FUNCTIONS OF COMPOUND ANGLESBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - TRIGONOMETRIC FUNCTIONS OF COMPOUND ANGLESEXERCISE - Exercise 2 (MISCELLANEOUS PROBLEMS)
20 Videos
Similar Questions
Knowledge Check
If C is the middle point of AB and P is any point outside AB, then
AP→A+P→B=P→CBP→A+P→B=2P→CCP→A+P→B+P→C=→0DP→A+P→B+2P→C=→0If C is the middle point of AB and P is any point outside AB, then
A→PA+→PB=→PCB→PA+→PB=2→PCC→PA+→PB+→PC=0D→PA+→PB+2→PC=0Which of the following statements are correct :- If M is the mid point of AB and O is any point, then
AOM=OA+MABOM=OA−MACOM=12(OA−OB)DOM=12(OB+OA)
If C is the middle point of AB and P is any point outside AB, then −−→PA+−−→PB=...
View SolutionIn Δ ABC, D is the mid-point of AB and P is any point on BC. If CQ∣∣PD meets AB and Q (shown in figure), then prove that
ar(ΔBPQ)=12ar(ΔABC).
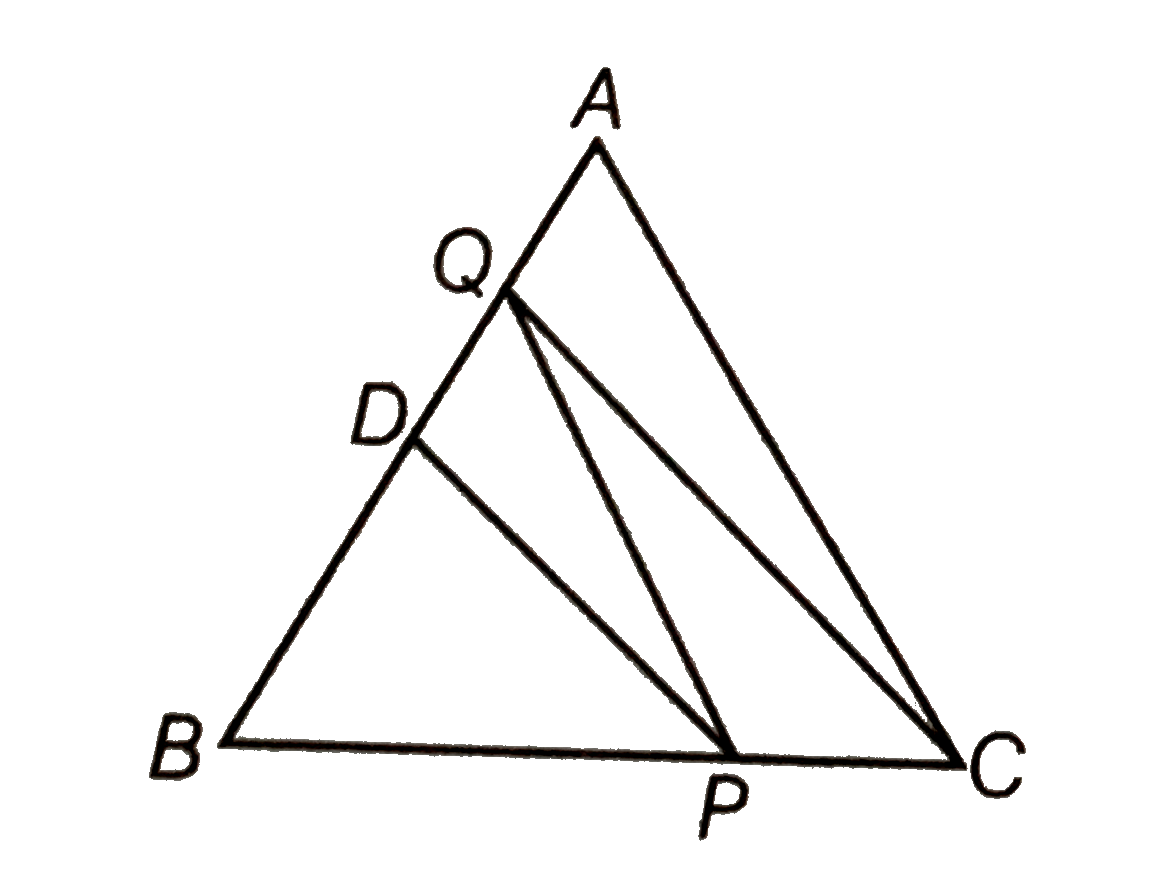
View SolutionIn Δ ABC, D is the mid-point of AB and P is any point on BC. If CQ∣∣PD meets AB and Q (shown in figure), then prove that
ar(ΔBPQ)=12ar(ΔABC).

View SolutionIn the given figure, in △ABC, D is the mid-point cf AB and P is any point on BC. If CQ∣∣PD meets AB in Q and area of △ABC=30cm2, then, area of △BPQ is
View SolutionIf →C is the midpoint of −−→ABand→P is any point outside −−→AB, then −−→PA+−−→PB=
View Solution
