Two simple harmonic motion y2Asinωt and y2=Acosωt are superimposed on a particle of mass m. The total mechanical energy of the particle is:
Phase difference between the two SHM is 90∘ Therefore, resultant amplitude is
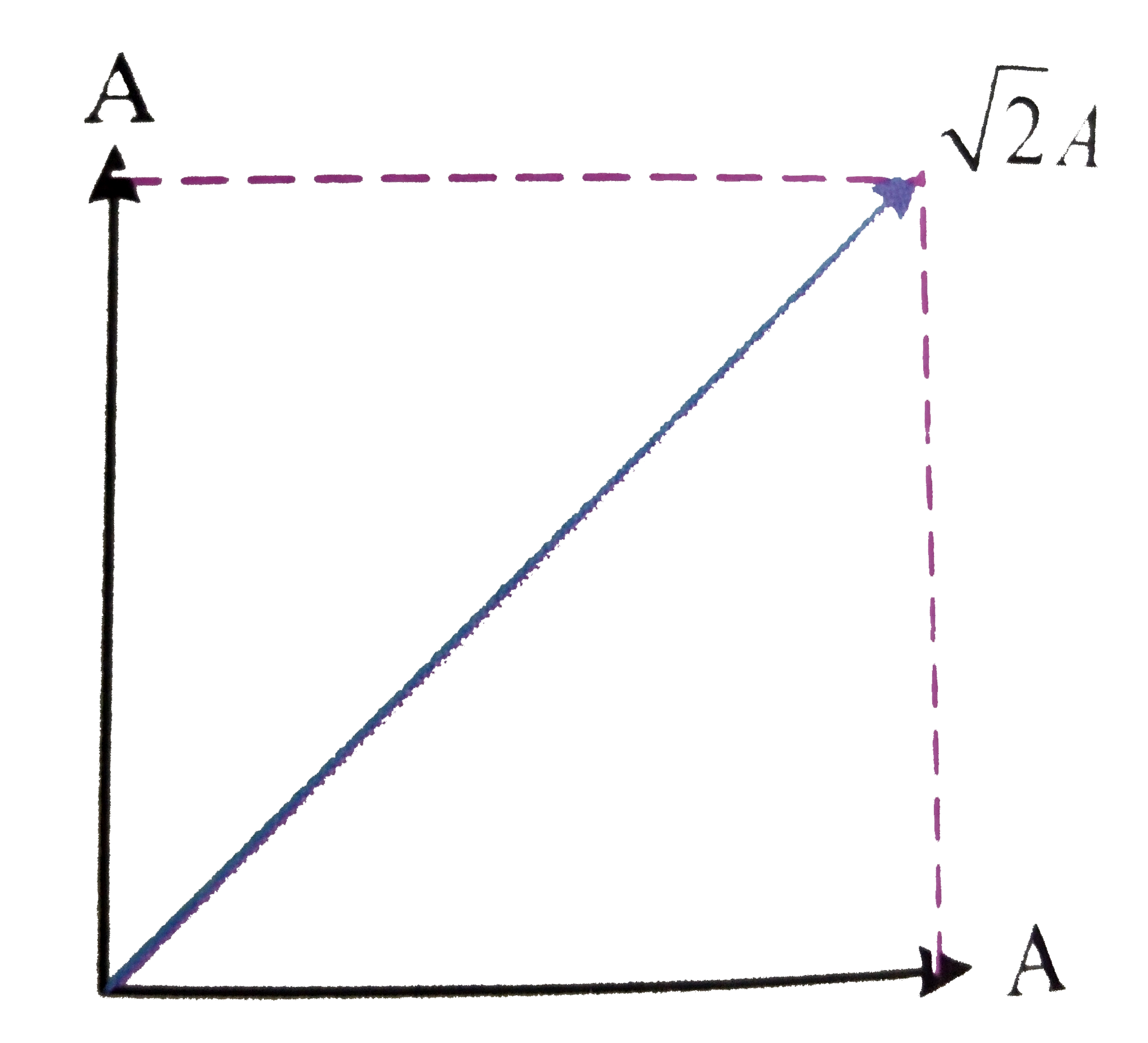
AR=√2A,TE=12m∙2=A2R
=12m∙2(√2A)2=m∙2A2
Topper's Solved these Questions
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - C.U.Q
78 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - LEVEL -I (C.W)
34 Videos
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - PASSAGE TYPE QUESTION
6 Videos
- PHYSICAL WORLDBOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q
10 Videos
Similar Questions
(a) A particle is subjected to two simple harmonic motions
x1=A1sinωt and x2=A2sin(ωt+π/3).
Find (i) the displacement at t=0, (ii) the maximum speed of the particle and (iii) the maximum acceleration of the particle.
(b) A particle is subjected to two simple harmonic motions, one along the x-axis and the other on a line making an angle of 45∘ with the x-axis. The two motions are given by
xy=x0sinωt and s=s0sinωt
Find the amplitude of the resultant motion.
View SolutionTwo waves of equation
y1=acos(ωt+kx)andy2=acos(ωt−kx) are superimposed upon each other. They will produce
View Solution
Knowledge Check
Two simple harmonic motions y1=Asinωt and y2 = Acosωt are superimposed on a particle of mass m. The total mechanical energy of the particle is
A12mω2A2Bmω2A2C14mω2A2DzeroTwo simple harmonic motions y1=Asinωt and y2=Acosωt are supre imposed on a particle of mass m.
A12mω2A2Bmω2A2C14mω2A2DzeroTwo waves y1=asin(ωt−kx) and y2=acos(ωt−kx) superimpose at a point :
AThe resultant amplitude is √2aBIf l is the intensity of each source then Imax=2lCThe resultant wave leads y1 by a phase angle of π4DData insufficient to arrive at options (B) and ("C")
Two simple harmonic motions are given by
y1=A1sinωtandy2=A2sin(ωt+ϕ) are acting on the particles in the same direction .The resultant motion is S.H.M., its amplitude is
View SolutionTwo waves y1=2sinωt and y2=4sin(ωt+δ) superimpose. The ratio of the maximum to the minimum intensity of the resultant wave is
View SolutionTwo waves y1=2sinωt and y2=4sin(ωt+δ) superimpose. The ratio of the maximum to the minimum intensity of the resultant wave is
View SolutionTwo waves
y1=Asin(ωt−kx)
and y2=Asin(ωt+kx)
superimpose to produce a stationary wave, then
View SolutionThe epoch of a simple harmonic motion represented by x=√3sinωt+cosωtmis
View Solution
