For a body in SHM the velocity is given by the relation V=√144−16x2ms−1. The maximum acceleration is
12m/s2
16m/s2
36m/s2
48m/s2
The correct Answer is:D
To find the maximum acceleration of a body in simple harmonic motion (SHM) given the velocity equation V=√144−16x2ms−1, we can follow these steps:
Step 1: Understand the relationship between velocity and displacement in SHM.
In SHM, the velocity V can be expressed in terms of displacement x as:
V=ω√A2−x2
where ω is the angular frequency and A is the amplitude.
Step 2: Compare the given equation with the standard form.
From the given equation:
V=√144−16x2
we can rewrite it as:
V=√122−(4x)2
This implies that:
- ω=12rad/s
- A=3m (since 4A=12⇒A=3)
Step 3: Use the formula for maximum acceleration.
The maximum acceleration Amax in SHM is given by:
Amax=ω2A
Step 4: Calculate Amax.
Substituting the values of ω and A:
Amax=(12)2×3=144×3=432m/s2
Conclusion:
The maximum acceleration is 432m/s2.
---
In SHM, the velocity V can be expressed in terms of displacement x as:
V=ω√A2−x2
where ω is the angular frequency and A is the amplitude.
From the given equation:
V=√144−16x2
we can rewrite it as:
V=√122−(4x)2
This implies that:
- ω=12rad/s
- A=3m (since 4A=12⇒A=3)
The maximum acceleration Amax in SHM is given by:
Amax=ω2A
Substituting the values of ω and A:
Amax=(12)2×3=144×3=432m/s2
The maximum acceleration is 432m/s2.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - LEVEL -II (C.W)36videos
OSCILLATIONS
- BOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - LEVEL -III51videos
OSCILLATIONS
- BOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - C.U.Q78videos
OSCILLATIONS
- BOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - PASSAGE TYPE QUESTION6videos
NEWTONS LAWS OF MOTION
- BOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q10videos
PHYSICAL WORLD
Similar Questions
The velocity of a particle is given by v=(2t2−3t+10)ms−1. Find the instantaneous acceleration at t = 5 s.
View SolutionA body executes SHM, such that its velocity at the mean position is 1ms−1 and acceleration at exterme position is 1.57ms−2. Calculate the amplitude and the time period of oscillation.
View Solution
Knowledge Check
- Question 1 - Select One
If the velocity of a particle is given by v=(180−16x)12ms, then its acceleration will be
AzeroB8ms2C−8ms2D4ms2 - Question 2 - Select One
For a particle in SHM the amplitude and maximum velocity are A and V respectively. Then its maximum acceleration is
AV22ABV2ACV2ADVA - Question 3 - Select One
The velocity of a particle varies with its displacement as v=(√9−x2)ms−1.
Find the magnitude of the maximum acceleration of the particle.A3ms−2B4ms−2C3.5ms−2D5ms−2
The maximum velocity of a particle executing SHM is 100cms−1 and the maximum acceleration is 157cms−2 determine the periodic time
View SolutionThe rod of length l=1m rotates with an angular velocity ω=2rads−1 an the point P moves with velocity v=1ms−1 and acceleration a=1ms−2. Find the velocity and acceleration of Q.
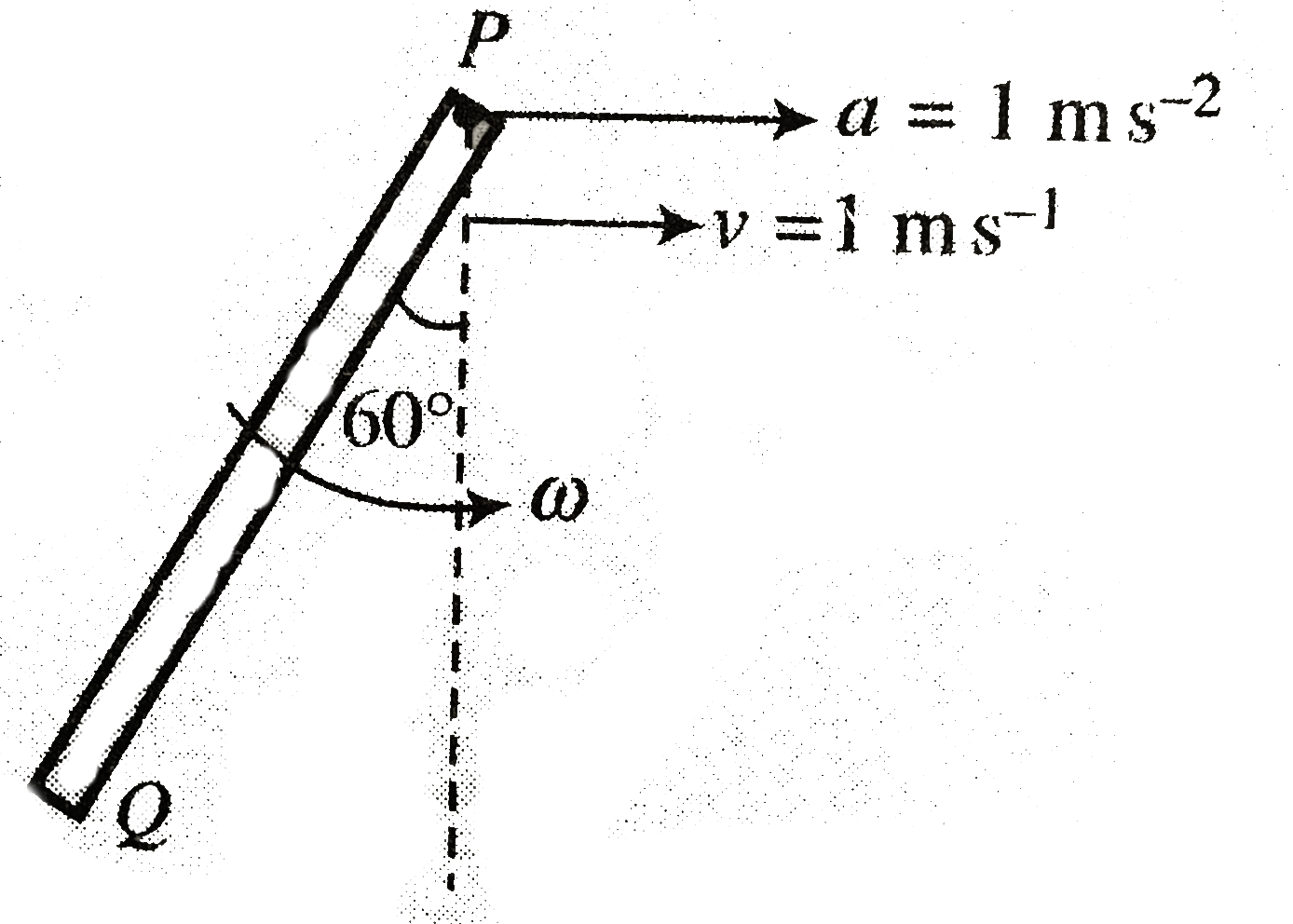 View Solution
View SolutionA particle is executing a linear S.H.M. Its velocity at a distance x from the mean position is given by v2=144−9x2. The maximum velocity of the particle is
View SolutionA body of mass 1/4kg is in SHM and its displacement is given by the relation x=0.05Sin(20t+π2)m. If is in seconds, the maximum force acting on the particle is
View SolutionA body is projected from the ground with a velocity v=(3ˆi+10ˆj)ms−1. The maximum height attained and the range of the body respectively are (given g=10ms−2)
View Solution

