A load of mass M is attached to the bottom of a spring of mass 'M/3' and spring constant 'K'. If the system is set into oscillation, the time period of oscillation is
2π3√10MK
4π√M3K
4π√MK
2π√M3K
The correct Answer is:A
To find the time period of oscillation for the given system, we need to consider both the mass of the load and the mass of the spring itself. Here’s a step-by-step solution:
Step 1: Identify the masses involved
- The mass of the load attached to the spring is M.
- The mass of the spring is M3.
Step 2: Calculate the effective mass
When a mass is attached to a spring, the oscillation is influenced by both the mass of the load and the mass of the spring. Therefore, we need to find the total effective mass Meff that contributes to the oscillation:
Meff=M+M3
Step 3: Simplify the effective mass
To simplify Meff:
Meff=M+M3=3M3+M3=4M3
Step 4: Use the formula for the time period of oscillation
The formula for the time period T of a mass-spring system is given by:
T=2π√MeffK
Substituting the effective mass into the formula:
T=2π√4M3K
Step 5: Simplify the time period expression
Now, simplify the expression for T:
T=2π√4M3K=2π⋅2√M√3K=4π√M√3K
Final Result
Thus, the time period of oscillation for the system is:
T=4π√M√3K
---
- The mass of the load attached to the spring is M.
- The mass of the spring is M3.
When a mass is attached to a spring, the oscillation is influenced by both the mass of the load and the mass of the spring. Therefore, we need to find the total effective mass Meff that contributes to the oscillation:
Meff=M+M3
To simplify Meff:
Meff=M+M3=3M3+M3=4M3
The formula for the time period T of a mass-spring system is given by:
T=2π√MeffK
Substituting the effective mass into the formula:
T=2π√4M3K
Now, simplify the expression for T:
T=2π√4M3K=2π⋅2√M√3K=4π√M√3K
Thus, the time period of oscillation for the system is:
T=4π√M√3K
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - SIMPLE PENDULUM14videos
OSCILLATIONS
- BOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - DAMPED AND FORCED OSCILLATIONS2videos
OSCILLATIONS
- BOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - ENERGY OF A PARTICLE EXECUTING SHM9videos
OSCILLATIONS
- BOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - PASSAGE TYPE QUESTION6videos
NEWTONS LAWS OF MOTION
- BOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q10videos
PHYSICAL WORLD
Similar Questions
Springs of spring constant k, 2k, 4k , 8k, …. Are connected in series. A block of mass 50 kg is attached to the lower end of the last spring and the system is set into oscillation. If k = 3.0 N/cm, what is the time period of oscillations ?
View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass m hangs from three springs having same spring constant k. If the mass is slightly displaced downwards, the time period of oscillation will be
 A2π√m3kB2π√3m2kC2π√2m3kD2π√3km
A2π√m3kB2π√3m2kC2π√2m3kD2π√3km - Question 2 - Select One
A particle of mass m is attached to three identical springs of spring constant k as shwon in figure. The time period of vertical oscillation of the particle is
 A2π√mkB2π√m2kC2π√m3kDπ√mk
A2π√mkB2π√m2kC2π√m3kDπ√mk - Question 3 - Select One
A uniform disc of mass m is attached to a spring of spring constant k as shown in figure and there is sufficient friction to prevent slipping of disc. Time period of small oscillations of disc is:
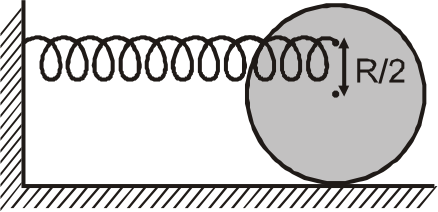 A2π√mkB2π√2m3kCπ√3m2kDπ√2m3k
A2π√mkB2π√2m3kCπ√3m2kDπ√2m3k
Springs of apring constants k,2k,4k,8k . . . Are connected in series. A mass m kg is attached to the lower end of the last spring and the system is allowed to vibrate. What is the time period of oscillations?
View SolutionSprings of spring constants k, 2k, 4k, 8k, …… are connected in series. A mass m kg is attached to the lower end of the last spring and the systemm is allowed to vibrate. What is the time period of oscillations? Given m=40gm,k=2.0Ncm−1.
View Solution
The period of oscillation of a mass M suspended from a spring of spring constant K is T. the time period of oscillation of combined blocks isView SolutionA particle of mass m is attached to three identical massless springs of spring constant k as shown in the m 135 figure. The time period of vertical oscillation of the particle is
 View Solution
View SolutionA body of mass m is suspended from three springs as shown in figure. If mass m is displaced slightly then time period of oscillation is
 View Solution
View Solution

