Springs of spring constant k, 2k, 4k , 8k, …. Are connected in series. A block of mass 50 kg is attached to the lower end of the last spring and the system is set into oscillation. If k = 3.0 N/cm, what is the time period of oscillations ?
To find the time period of oscillations for the given system of springs connected in series, we can follow these steps:
Step 1: Identify the Spring Constants
The spring constants of the springs in series are given as k,2k,4k,8k,…. This forms a geometric series where:
- First term a=k
- Common ratio r=2
Step 2: Write the Formula for Equivalent Spring Constant
For springs in series, the equivalent spring constant keq can be calculated using the formula:
1keq=1k1+1k2+1k3+…
In our case:
1keq=1k+12k+14k+18k+…
Step 3: Sum the Infinite Series
This series can be simplified as follows:
1keq=1k(1+12+14+18+…)
The series inside the parentheses is a geometric series with:
- First term a=1
- Common ratio r=12
The sum of an infinite geometric series is given by:
S=a1−r=11−12=2
Thus,
1keq=1k⋅2⟹keq=k2
Step 4: Substitute the Given Spring Constant
Given k=3N/cm, we need to convert this to SI units:
k=3N/cm=3×100N/m=300N/m
Now substituting into the equivalent spring constant:
keq=3002=150N/m
Step 5: Calculate the Time Period
The time period T of oscillation for a mass-spring system is given by:
T=2π√mkeq
Substituting the values:
- Mass m=50kg
- keq=150N/m
T=2π√50150=2π√13=2π⋅1√3≈2⋅3.14⋅0.577≈3.66s
Final Answer
The time period of oscillations is approximately T≈3.66s.
---
The spring constants of the springs in series are given as k,2k,4k,8k,…. This forms a geometric series where:
- First term a=k
- Common ratio r=2
For springs in series, the equivalent spring constant keq can be calculated using the formula:
1keq=1k1+1k2+1k3+…
In our case:
1keq=1k+12k+14k+18k+…
This series can be simplified as follows:
1keq=1k(1+12+14+18+…)
The series inside the parentheses is a geometric series with:
- First term a=1
- Common ratio r=12
S=a1−r=11−12=2
Thus,
1keq=1k⋅2⟹keq=k2
Given k=3N/cm, we need to convert this to SI units:
k=3N/cm=3×100N/m=300N/m
Now substituting into the equivalent spring constant:
keq=3002=150N/m
The time period T of oscillation for a mass-spring system is given by:
T=2π√mkeq
Substituting the values:
- Mass m=50kg
- keq=150N/m
The time period of oscillations is approximately T≈3.66s.
Topper's Solved these Questions
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Revision Exercises (Very Short Answer Questions)
37 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Revision Exercises (Additional Question )
3 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - NCERT Exemplar Problems Subjective Question ( short Answer Type Questions )
6 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST
16 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
Similar Questions
Springs of spring constants k, 2k, 4k, 8k, …… are connected in series. A mass m kg is attached to the lower end of the last spring and the systemm is allowed to vibrate. What is the time period of oscillations? Given m=40gm,k=2.0Ncm−1.
View SolutionSprings of apring constants k,2k,4k,8k . . . Are connected in series. A mass m kg is attached to the lower end of the last spring and the system is allowed to vibrate. What is the time period of oscillations?
View Solution
Knowledge Check
Springs of spring constant K,3K,9K,27K,….243K are connected in series. A mass M is attached to the last spring and the system is allowed to make oscillation. The time period will be
A2π√MKB2π√3M2KC4π9√91M3KD2π√4M3KA load of mass M is attached to the bottom of a spring of mass 'M/3' and spring constant 'K'. If the system is set into oscillation, the time period of oscillation is
A2π3√10MKB4π√M3KC4π√MKD2π√M3KSprings of constants k, 2k, 4k, 8k,…….,2048k are connected in series. A mass m is attached to one end and the system is allowed to oscillate. The time period is approximately
A2π√m2kB2π√2mkC2π√m4kD2π√4mk
Infinite spring with force constants k,2k,4k,8k,……..respectively are connected in series. Calculate the effetive force constant of the spring.
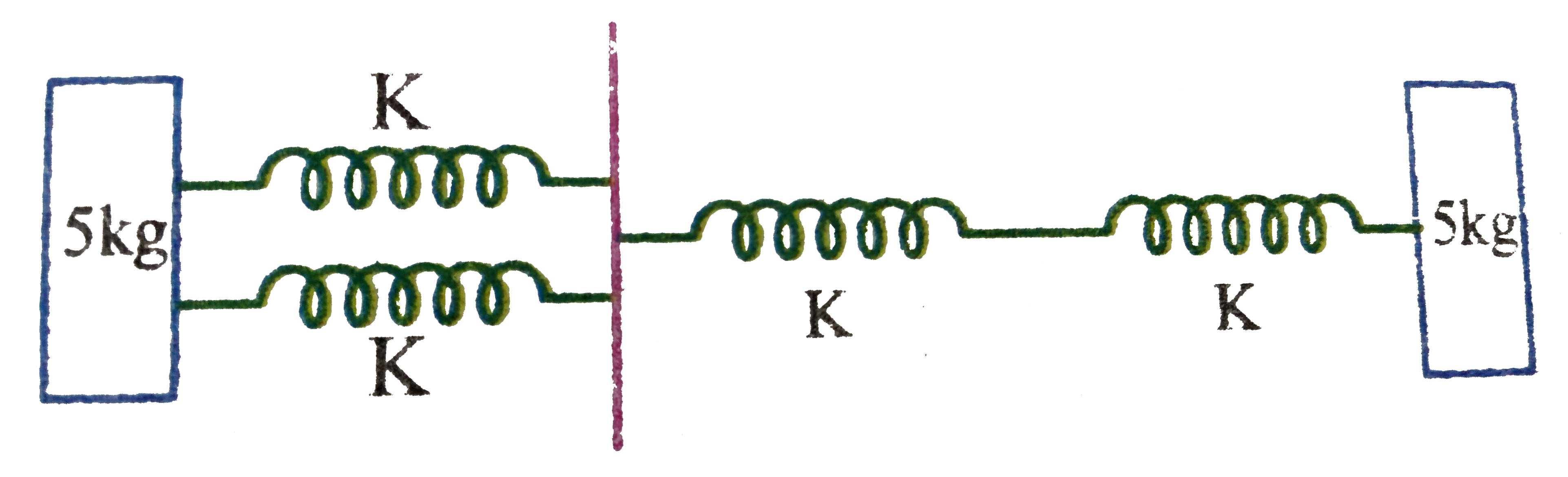
View SolutionIn the given spring block system if k=25π2Nm−1, find time period of oscillation.
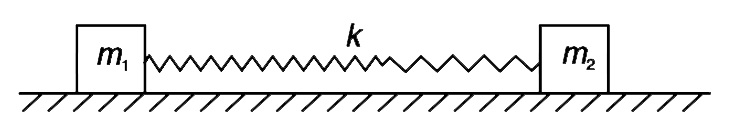
View SolutionTwo blocks rest on a smooth horizontal surface. They are connected by a spring of force constant k. If the system is set into oscillation find its time period.
View SolutionInfinite springs with force constants k,2k,4k,8k,….. respectively are connected in series to which mass 'm' is attched. The frequency of small oscillations is……..
View SolutionInfinite springs with force constantk , 2k, 4k and 8 k .... respectively are connected in series. The effective force constant of the spring will b
View Solution
