A block 'A' of mass 2m is placed on another block 'B' of mass 4m which in turn is placed on a fixed table. The two blocks have a same length 4d and they are placed as shown in fig The coefficient of friction (both static and kinetic) between the block 'B' and table is μ. There is no friction between the two blocks. A small object of mass m moving horizontally along a line passing through the centre of mass (cm.) of the block B and perpendicular to its face with a speed v collides elastically with the block B at a height d above the table.
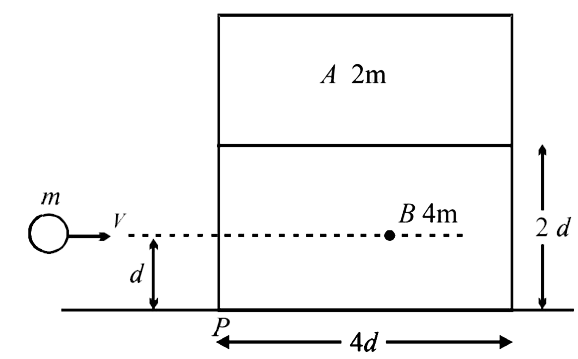
(a) What is the minimum value of v(call it v0) required to make the block A topple?
(b) If v=2v0,find the distance (from the point P in the figure ) at which the mass m falls on the table after collision. (Ignore the role of friction during the collision).
The correct Answer is:(a) 52√6μgd , (b) 6d sqrt(3 mu)
If v1 and v2 are the velocities of object of mass m and block of mass 4m, just after collision then by conservation of momentum,
mv=mv1+4mv2,
i.e., v=v1+4v2 …(1)
Further, as collision is elastic
12mv2=12mv21+124mv22, i.e.,
v2=v21+4v22 ...(2)
Soliving, these two equations we get either
v2=0 or v2=25v
Therefore, v2=25v , Substituting in Eq. (1)
v1=35v
When v2=0,v−(1)=v2, but it is physically unacceptable.
(a) Now, after collision the block B will start moving with velocity v2 to the right. Since, there is no friction between blocks A and B the upper block A will stay at its position and will topple if B moves a distance s such that s>2d ....(3)
However, the motion of B is retarded by frictional force f=m(4m+2m)g between table and iis lower surface. So, the distance moved by B till stops
0=v22>6gd or 25v>√6μgd[asv2=2v5]
i.e., v>52√6m or vmin=v0=56√μgd
(b) If v=2v0=5√6μgd, the object will rebound
with speed , v1=35v=3√6μgd
and as time taken by it to fall down ltbgt t=√2hg=√2dg[ash=d]
The horizontal distance moved by it to the left of P in this time x=v1t=6d√3μ
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - COLLISIONEXERCISE - Level-I (H.W)52videos
COLLISION
- BOOK - NARAYNACHAPTER - COLLISIONEXERCISE - Level-II (H.W)54videos
COLLISION
- BOOK - NARAYNACHAPTER - COLLISIONEXERCISE - Comprehension type35videos
COLLISION
- BOOK - NARAYNACHAPTER - CIRCULAR MOTIONEXERCISE - LEVEL II(H.W)51videos
CIRCULAR MOTION
- BOOK - NARAYNACHAPTER - FRICTIONEXERCISE - Passage type of questions I6videos
FRICTION
Similar Questions
A block on table shown in is just on the edge of slipping Find the coefficient of static friction between the blocks and table
 .View Solution
.View SolutionThe block of mass M and are arrenged as the situation in fig is shown.The coefficient of friction between two block is μand that between the bigger block and the ground is μ find the acceleration of the block
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass m=4kg is placed oner a rough inclined plane as shown in figure. The coefficient of friction between the block and the plane is μ=0.5 . A force F=10N is applied on the block at an angle of 30∘ .
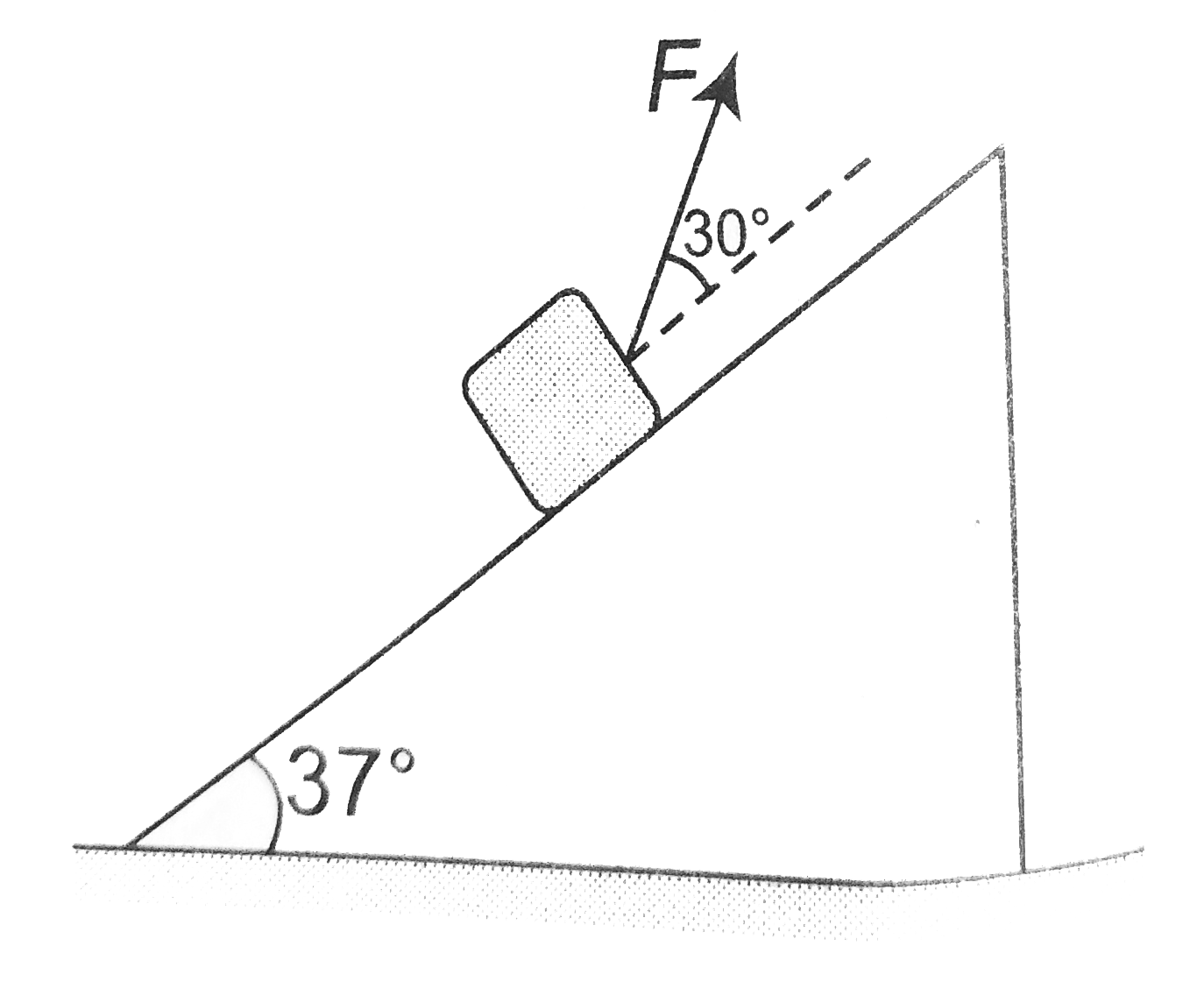 Astatic in nature in the direction up the plane and have the value 30.2NBstatic in nature in the direction down the plane and have the value 30.2NCkinetic in nature in the direction up the plane and have the value 30.2NDNone of these
Astatic in nature in the direction up the plane and have the value 30.2NBstatic in nature in the direction down the plane and have the value 30.2NCkinetic in nature in the direction up the plane and have the value 30.2NDNone of these - Question 2 - Select One
A block of massm is placed on another block of mass M which itself is lying on a horizontal surface .The coefficient of friction between two blocks is μ1and that between the block of mass M and horizontal surfece is μ2 What maximum horizontal force can be applied to the lower block move without separation?
 A(M+m)(μ2−μ1)gB(M−m)(μ2−μ1)gC(M−m)(μ2+μ1)gD(M+m)(μ2+μ1)g
A(M+m)(μ2−μ1)gB(M−m)(μ2−μ1)gC(M−m)(μ2+μ1)gD(M+m)(μ2+μ1)g - Question 3 - Select One
The coefficient of friction between the block A of mass m & block B of mass 2m is μ. There is no friction between A&B is released from rest & there is no slipping between A&B then:
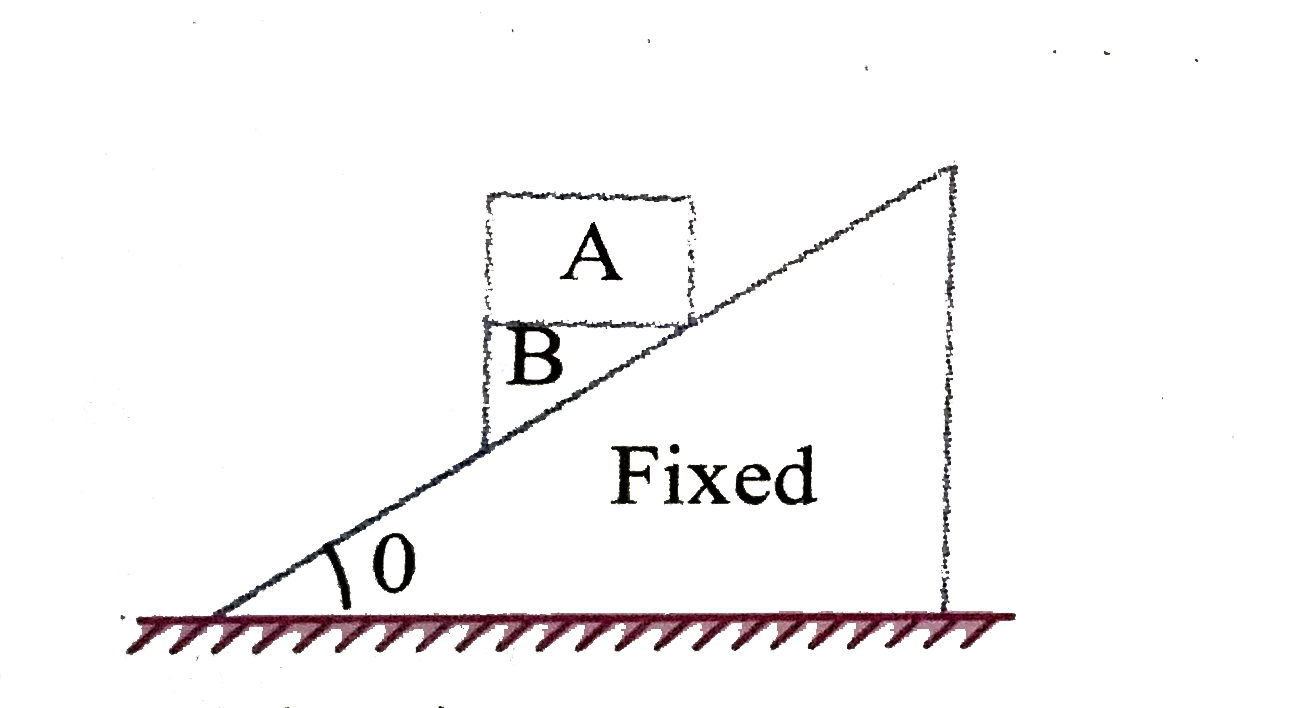 A2θ≤sin−1(2μ)Bθ≤tan−1(μ)C2θ≤cos−1(2μ)D2θ≤tan−1(μ/2)
A2θ≤sin−1(2μ)Bθ≤tan−1(μ)C2θ≤cos−1(2μ)D2θ≤tan−1(μ/2)
A block A slides over an another block B which is placed over a smooth inclined plane as shown in figure . The coefficient of friction between the two blocks A and B is μ . Mass of block B is two times the mass of block A . The acceleration of the centre of mass of two blocks is
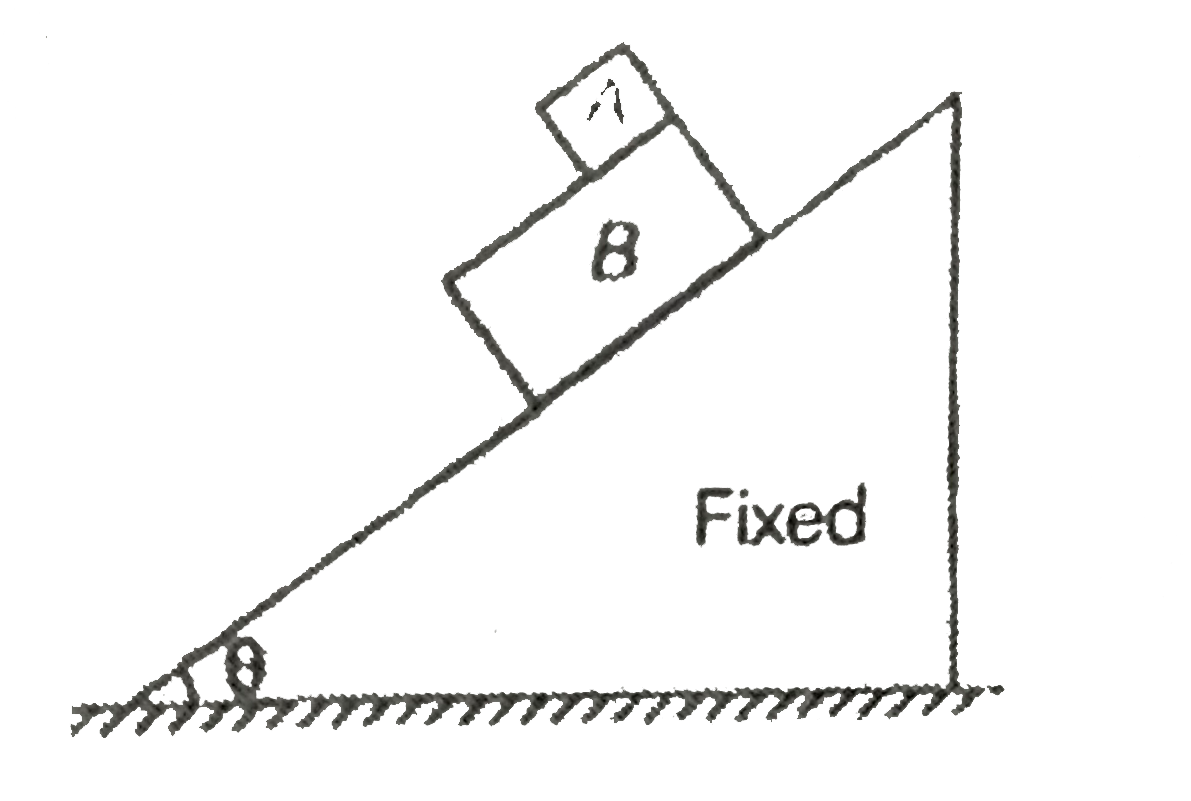 View Solution
View SolutionFind the acceleration of the block of mass M in the situation of figure. The coefficient of friction between the two blocks is μ1 and that between the bigger block and the ground is μ2.
View SolutionA cart of mass M has a block of mass m in contact with it as shown in the figure-2.133. The coefficient of friction between the block and the cart is μ. What is the minimum acceleration of the cart so that the block m does not fall ?
 View Solution
View SolutionA block of mass m is placed on the top of another block of mass M as shown in the figure. The coefficient of friction between them is μ.

The maximum acceleration with which the block M may move so that m also moves along with it isView SolutionTwo block of masses m1 and m2 are connected with a massless unstretched spring and placed over a plank moving with an acceleration 'a' as shown in figure. The coefficient of friction between the blocks and platform is μ
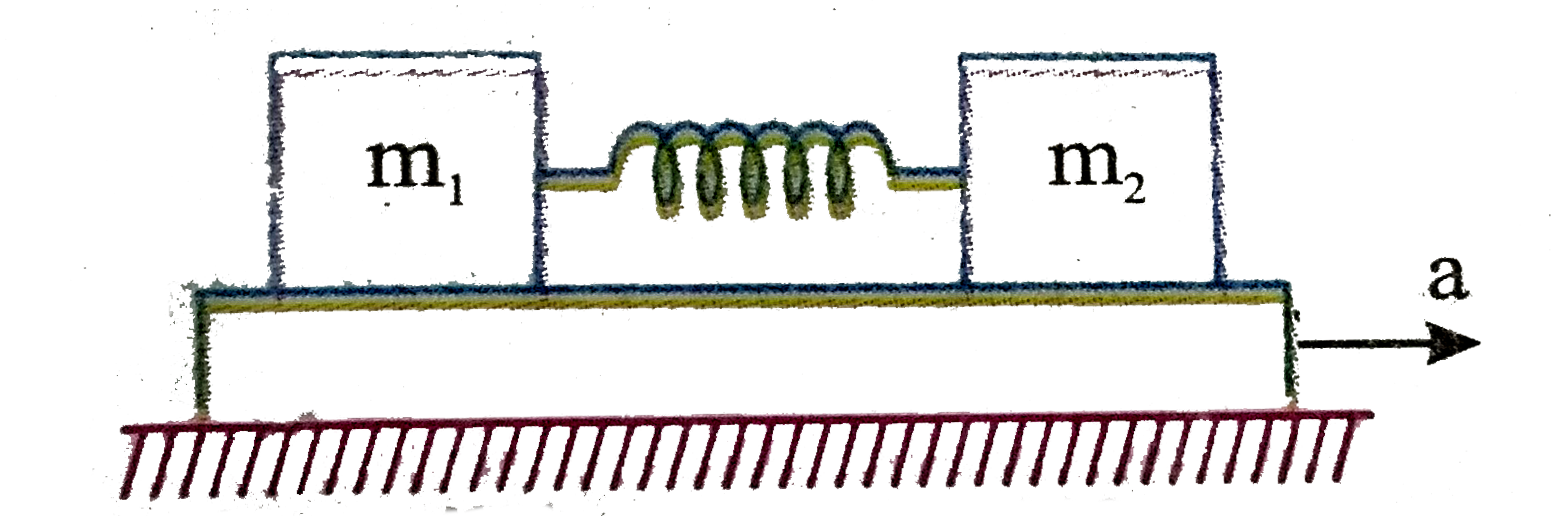 View Solution
View Solution

