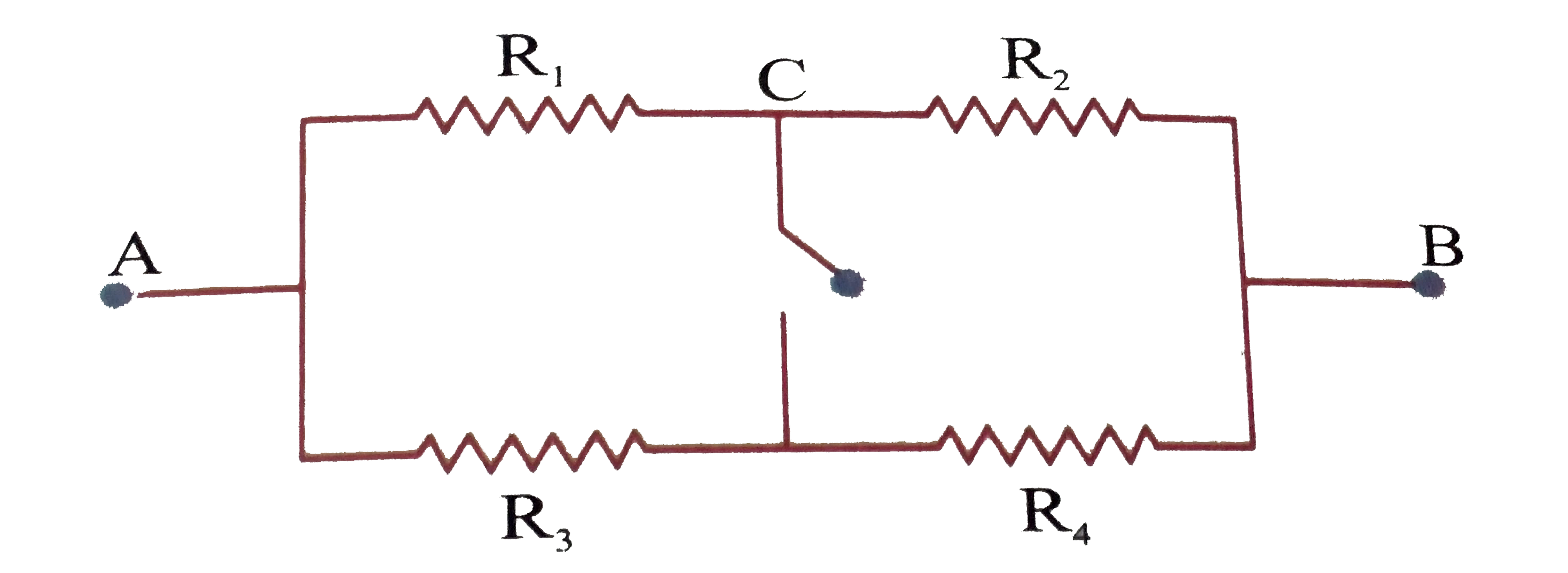
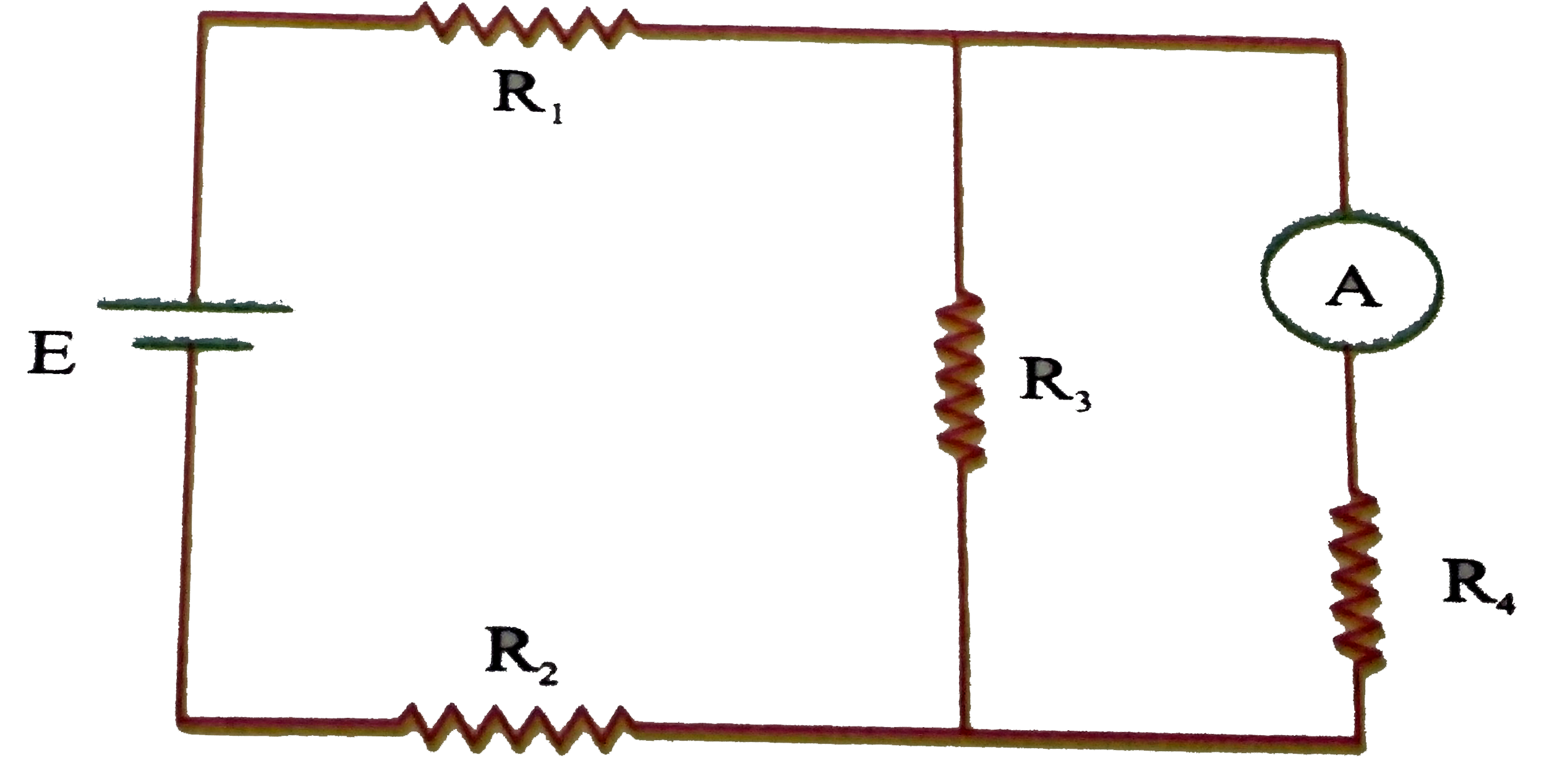
Four resistors R1,R2,R3 and R4 are connected between two terminals A and B iin a network as shown in the diagram. A key K can connect the two points (see diagram) C and D. A constant potential difference (VA−VB)=V is maintained between thhe points A and B
Q. If R1=R4=1Ω and R2=R3=2Ω, the effective resistance between the terminals A and B will change, on closing the key K, by
an increase of 0.5Ω
a decrease of 0.16Ω
an increase of 0.16Ω
a decrease of 0.5Ω
The correct Answer is:B
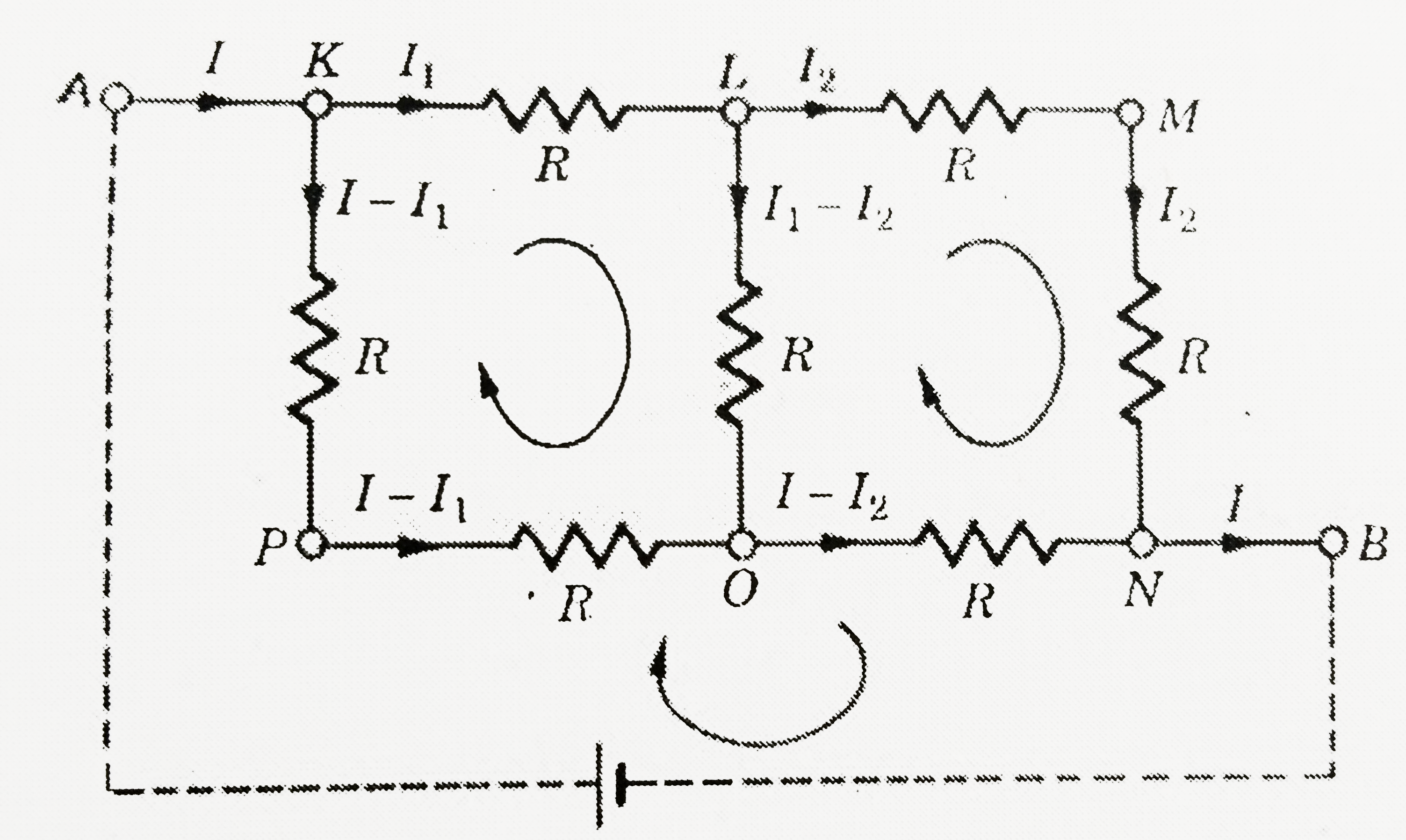
Let the resistance (small but zero) in the arm CD be x Ω. Assume currents i1,i2,i3,i4 and ix as shown in the diagram. Then applications of kirchhoff's junctions and loop laws give,
i1=i2+ix ..(i)
i3=i4−ix ..(ii)
V=i1R1+i1R2+i4R4 ..(iii)
and i1R1+Xix−i3R3=0 ..(iv)
Solving these equation for ix we get from (1) & (3)
V−i1(R1+R2)−ixR2 ..(v)
and from (2) and (3)
V−i3(R3+R4)+ixR4 ...(vi)
Sustituting for i1 from (5) and i3 from (vi) and (iv)
R1[V+ixR2R1+R2]+Xi2−R3[V−ixR4R3+R4]=0
Collecting thew terms in
ix,ix[x+R1R2R1+R2+R3R4R3+R4]
=V[R3R3+R4−R1R1R2]
⇒Ix=V[R3R3+R4−R1R1+R2]x+R1R2R1+R2+R3R4R3+R4
Proceeding to limit x→0 (x= m negligibly small)
ix=25[R3R3+r4−R1R1+R2][R1R2R1+R2+R3R4R3+R4] substituting values,
ix=25[37−13][23+127]=25×250=lamp
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - NARAYNACHAPTER - CURRENT ELECTRICITYEXERCISE - Level 5
42 Videos
- COMMUNICATION SYSTEMBOOK - NARAYNACHAPTER - COMMUNICATION SYSTEMEXERCISE - Level-II(H.W)
25 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - NARAYNACHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - ASSERTION AND REASON
15 Videos
Similar Questions
Knowledge Check
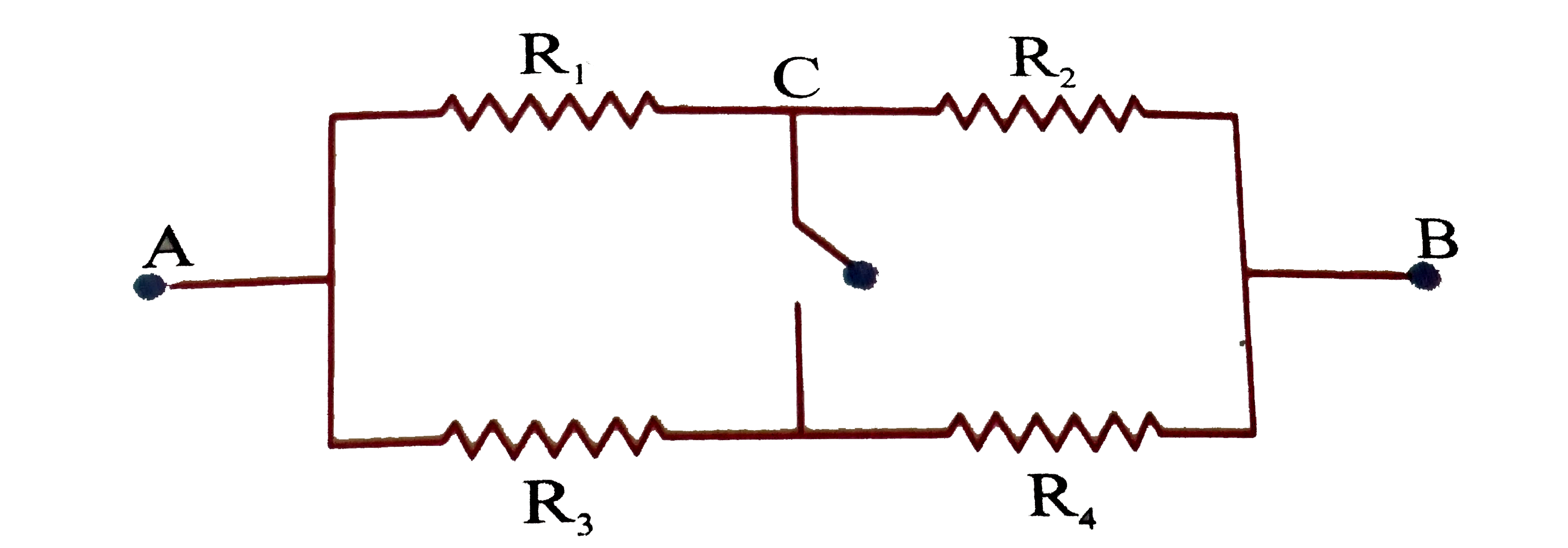
Four resistors R1,R2,R3 and R4 are connected between two terminals A and B iin a network as shown in the diagram. A key K can connect the two points (see diagram) C and D. A constant potential difference (VA−VB)=V is maintained between thhe points A and B
Q. If V=25,R1=1Ω,R2=2Ω,R3=3Ω and R4=4Ω the current that will flow from C to D on connecting key K isAzeroBinfinityC+1AD−1A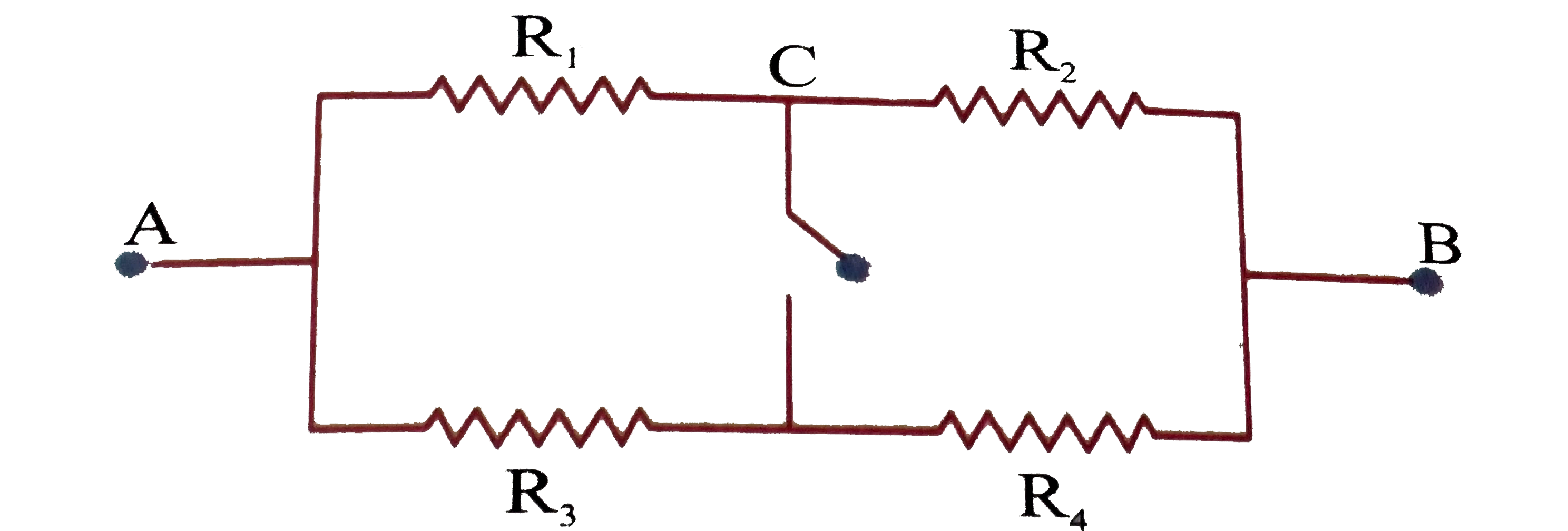
Four resistors R1,R2,R3 and R4 are connected between two terminals A and B iin a network as shown in the diagram. A key K can connect the two points (see diagram) C and D. A constant potential difference (VA−VB)=V is maintained between thhe points A and B
Q. In on connecting the key K, no current flows through the segment CD, whatever potential difference V exists between A and B which of the following cases will satisfy this condition?AR1=1Ω,R2=2Ω,R3=3Ω,R4=4ΩBR1=2Ω,R2=1Ω,R3=4Ω,R4=2ΩCR1=1Ω,R2=2Ω,R3=2Ω,R4=1ΩDR1=R2=2Ω,R3=2R4=4ΩAn infinite ladder network is arranged with resistances R and 2 R as shown. The effective resistance between terminals A and B is
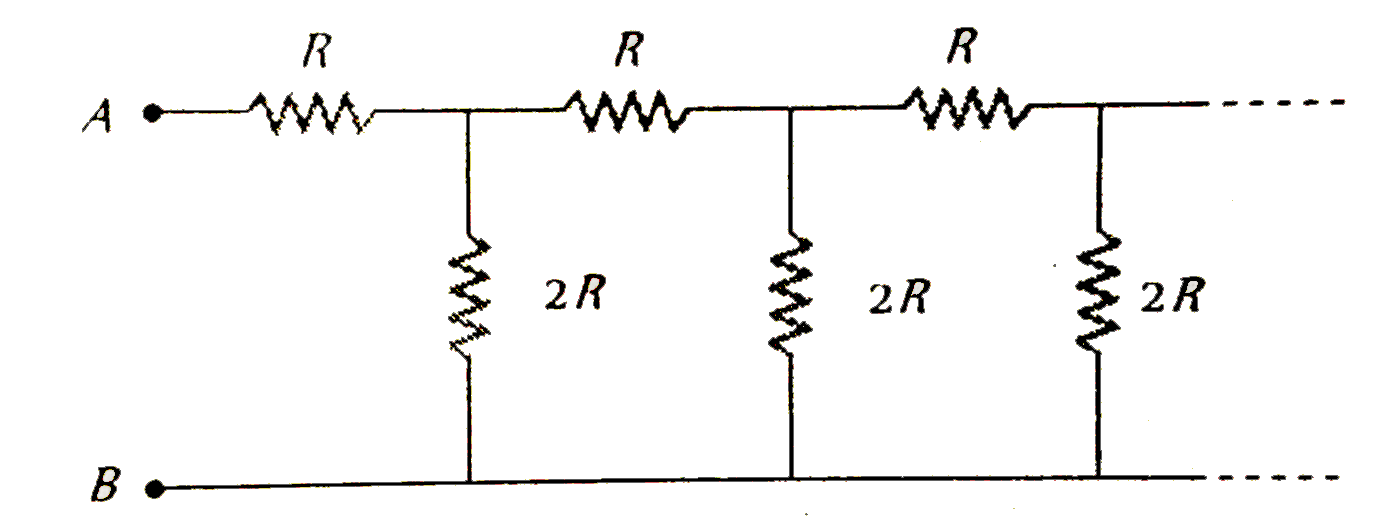 A∞BRC2RD3R
A∞BRC2RD3R
If each resistance R = 1 ohm then the equivalent resistance (in ohm) between the terminal points A and B in the network shown in figure is
View SolutionIn the circuit as shown in figure,
E1=2V,E2=2V,E3=1V,andR=r1=r2=r3Ω.
The potential difference between points A and B will be
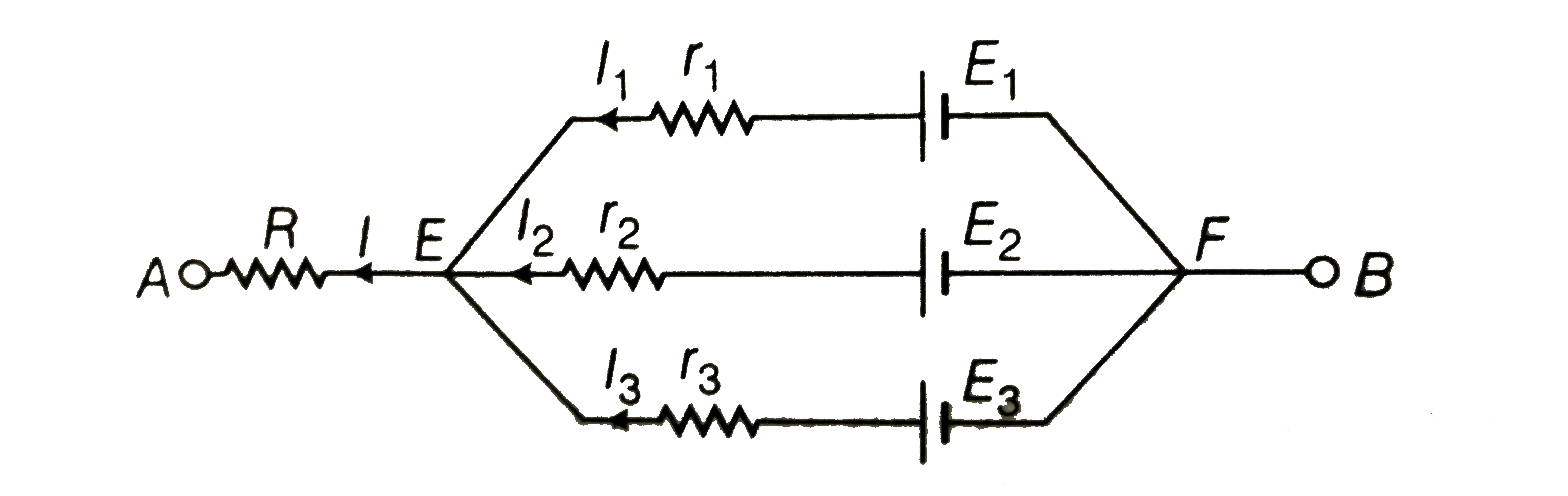
View Solution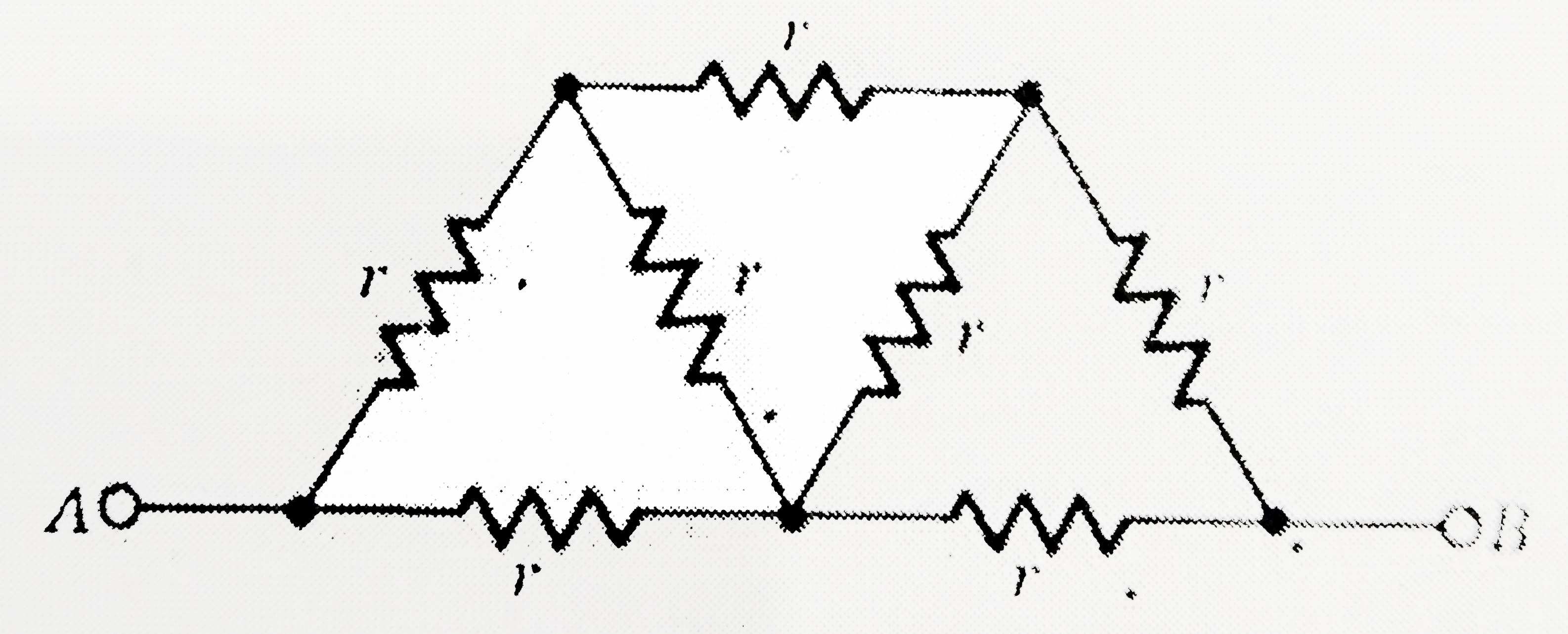
In the network as shown in figure. Each resistance r is of 2Ω find the effective resistance between points A and B.
View SolutionIn Fig. 27-9, what is the potential difference V.d−Vc between points d and c if ε1=4.0V.ε2−1.0V,R1=R2=10Ω,andR3=8.0Ω, and the battery is ideal?
View SolutionA network of four unequal resistor R1,R2,R3 and R4 are connected in a network (see diagram) that contains a source of e.m.f E and an ammeter A. The source has negligible internal resistance. For this network,
View Solution