A uniform chain of length of length πr lies inside a smooth semicircular tube (AB) of radius r. Assuming a slight disturbance to start the chain in motion, the velocity with which it will emerge from the end (B) of the tube will be

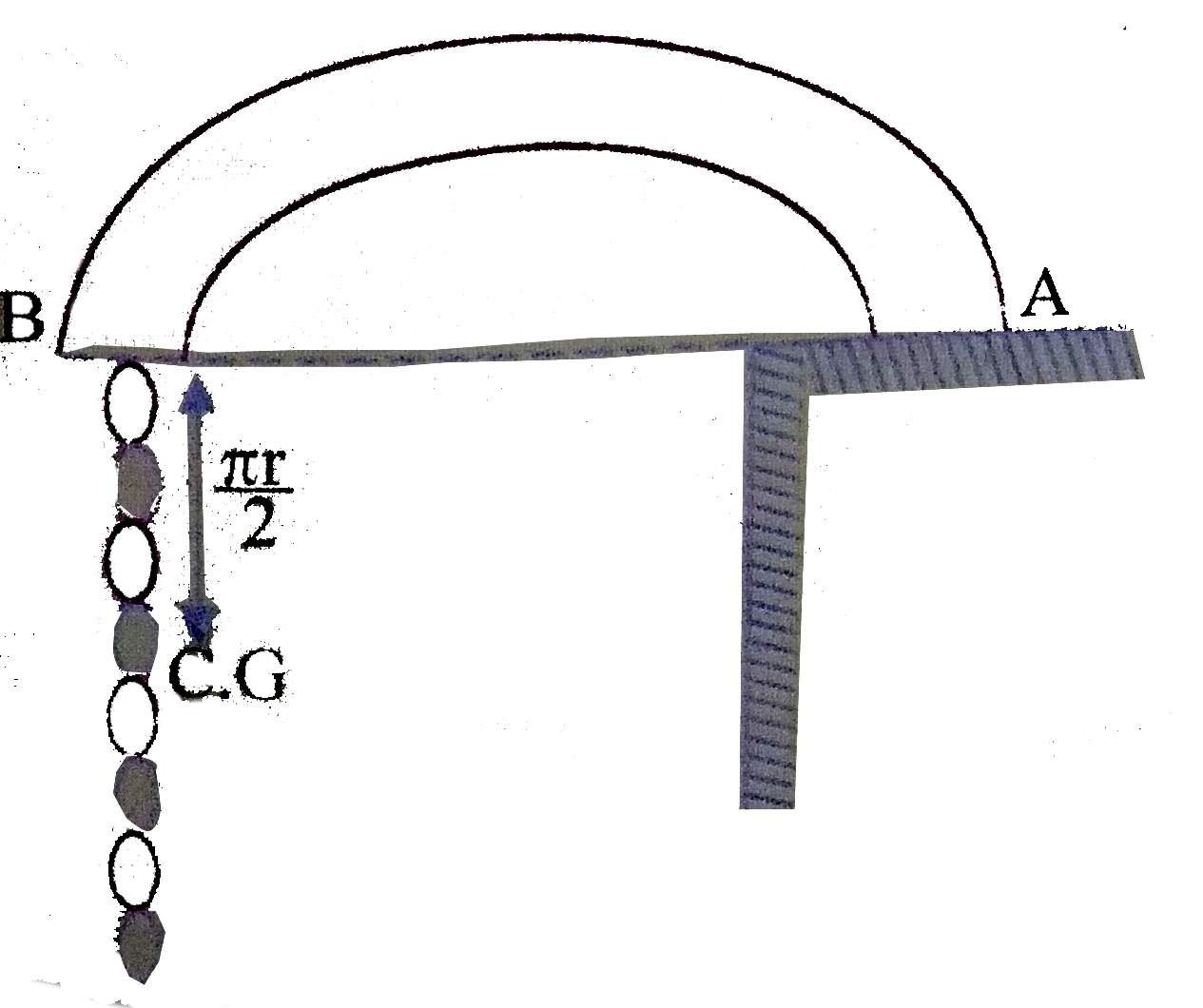
Centre of gravity if a semicircular arc is at a distance 2rπ from the centre.
Initial potential energy Ui=(λπr)g(2rπ)
Final potential energy Uf=(λπr)g(−πr2)
When the chain is completely slipped off the tube, all the links of the chain have the same velocity v.
kinetic energy of chain k=12mv2=12(λπr)v2
From conservation of energy,
λπrg(2rπ)=(λπr)g(−πr2)+12(λπr)v2
On solving we get, v=√2rg(2π+π2).
Topper's Solved these Questions
Similar Questions
A chain AB of length equal to the quarter of a circle of radius R is placed on a smooth hemisphere as shown in figure -3.66. When it is released, it starts falling. Find the velocity of the chain when it falls of the end B from the hemisphere.
 View Solution
View SolutionA uniform chain of length l and mass m lies on a smooth table. A very small part of this chain hangs from the table. It begins to fall under the weight of hanging part. Find the velocity of chain when the length of hanging part becomes y.
View Solution
Knowledge Check
- Question 1 - Select One
A uniform chain of length of length πr lies inside a smooth semicircular tube (AB) of radius f. Assuming a slight disturbance to start the chain in motion, the velocity it will emerge from the end (B) of the tube will be
 A√gr(1+29π)B√2gr(2π+π2π)C√gr(π+2)D√πgr
A√gr(1+29π)B√2gr(2π+π2π)C√gr(π+2)D√πgr - Question 2 - Select One
A heavy flexible uniform chain of length πr and mass λπr lies in a smooth semicircular tube AB of radius 'r'. Assuming a slight disturbane to start the chain in motion, find the velocity v with which it will emerge from the end of the tube?
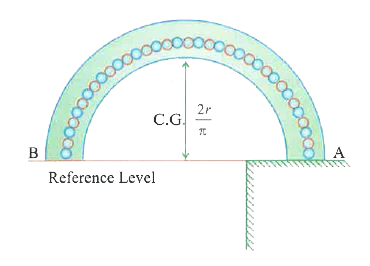 A√2π+π2B√2rg(2π+π2)C√2rg(1π+π)D√rf(π+2π)
A√2π+π2B√2rg(2π+π2)C√2rg(1π+π)D√rf(π+2π) - Question 3 - Select One
A smooth semicircular tube AB of radius r is fixed in a vertical plane and contains a heavy flexible chain of length πr and weight Wπr as shown. Assuming a slight disturbance to start the chain in motion, the velocity v with it will emerge from the open end B of the tube is:
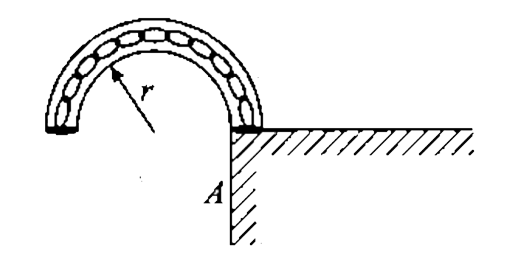 A4grπB2grπC√2gr(2π+π)D√2gr(2π+π2)
A4grπB2grπC√2gr(2π+π)D√2gr(2π+π2)
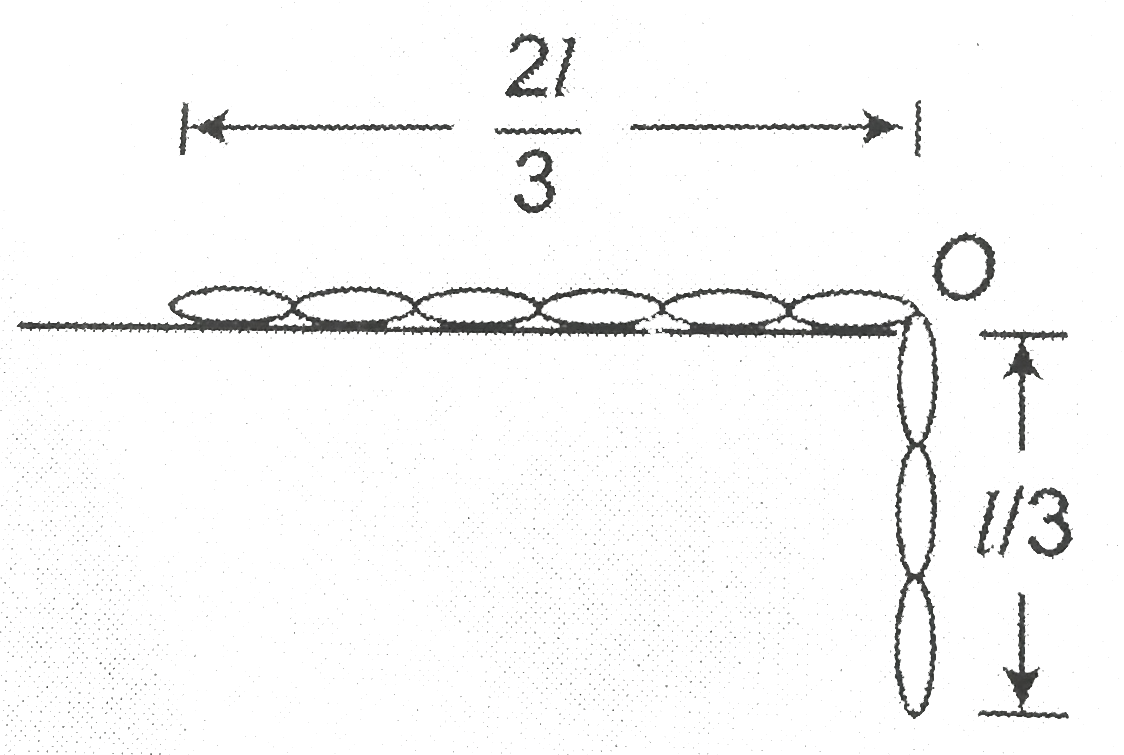
A uniform chain of mass m and length l is placed on a smooth table so that one−third length hanges freely as shown in the figure. Now the chain is released, with what velocity chain slips off the table?
 View Solution
View SolutionAS chain of length l and mass m lies o the surface of a smooth sphere of radius R>l with one end tied to the top of the sphere. a.Find the gravitational potential energy of the chain with reference level at the centre of the sphere. B. suppose the chin is released and slides down the sphere. Find the kinetic eneergy of the chain, when it has slid through an angle θ c. find the tangential acceleration dvdt of the chain when the chain starts sliding down.
View SolutionA uniform chain of length l is placed on a smooth horizontal table, such that half of its length hangs over one edge. It is releasedfrom rest, the velocity with which it leaves the table is
View SolutionA uniform chain of length L lies on a smooth horizontal table with its length perpendicular to the edge of the table and a small portion of the chain is hanging over the edge. The chain starts sliding due to the weight of the hanging part
View SolutionA chain AB of length l is located in a smooth horizontal tube so that its fraction of length h hangs freely and touches the surface of the table with its end B(figure). At a certain moment the end A of the chain is set free. With what velocity will this end of the chain slip out of the tube?
 View Solution
View Solution

