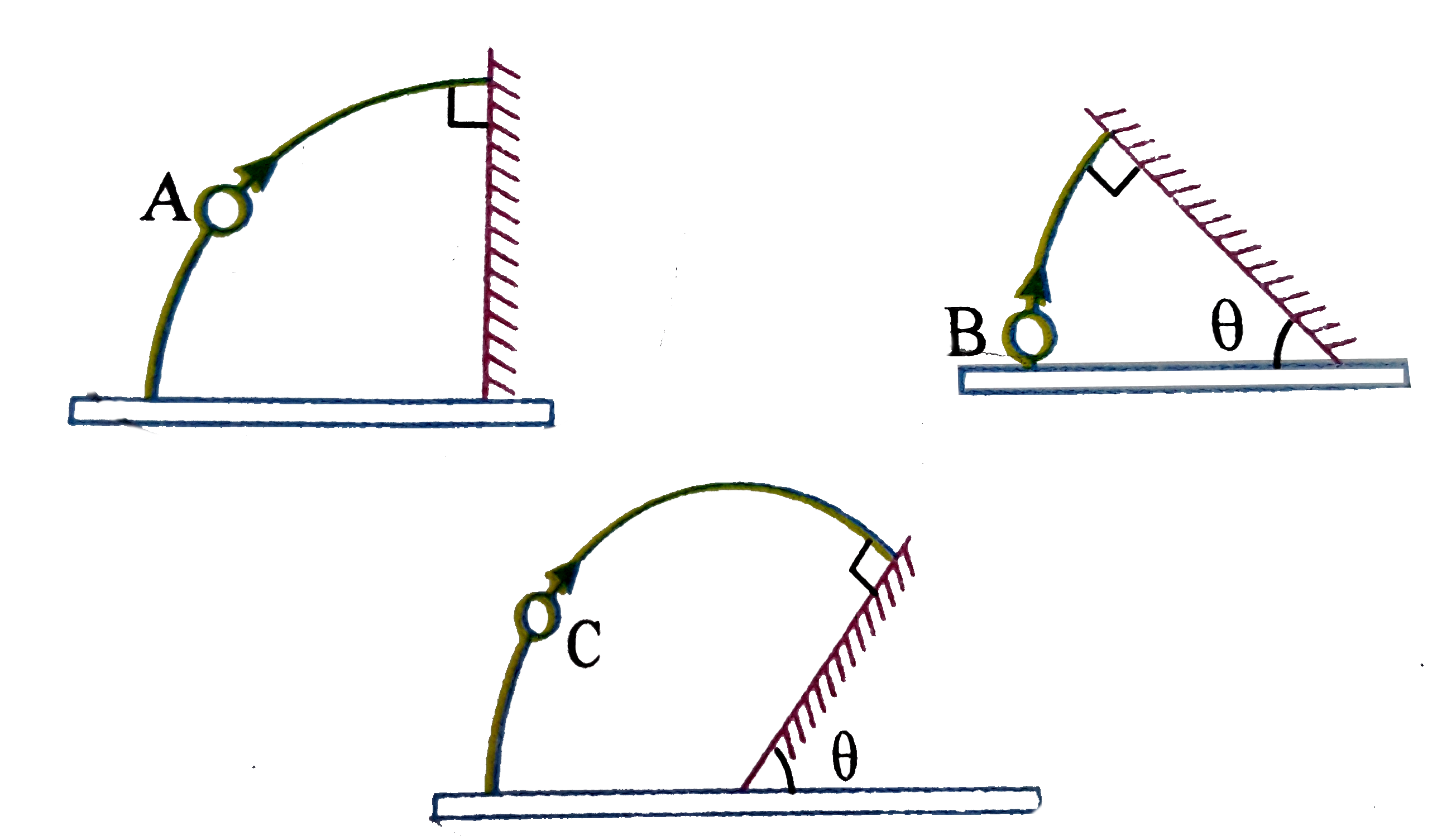
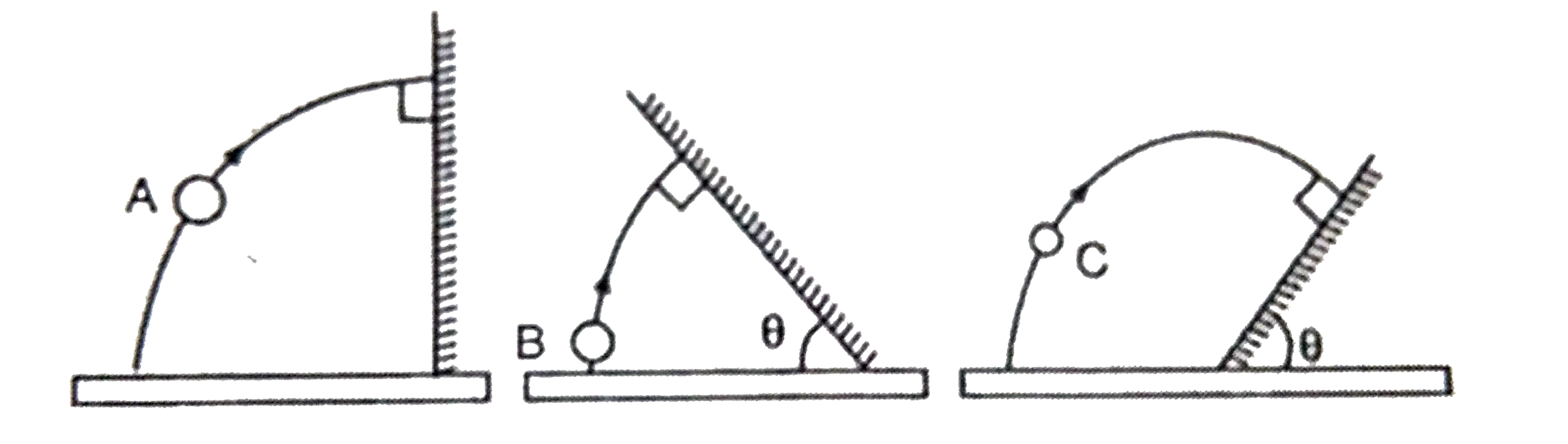
Three balls and are projected from ground with same speed at same angle with the horizontal.The balls and collide with the wall during a flight in air and all three collide perpendicularly and elastically with the wall as shown in figure.If the time taken by the ball and fall back on ground is seconds and that by ball is Then the time taken by the ball to reach the ground after projection will be
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-Vi single answer
30 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-Vi multiple answer
16 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level -V Passage
15 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)
24 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W
29 Videos
Similar Questions
Three balls A,B and C are projected from ground with same speed at same angle with the horizontal. The balls A,B and C collie with the wall during a flight in air and all three collide perpendicu lary and eleastically with the wall as shown in Fig. 5.119 it the time taken by the ball A to fall back on ground is 4 seconds and that by ball B is 2 seconds. Then the time taken by the ball C to reach the ground after projection will be:
View SolutionA ball is projected from ground with a speed of 20ms−1 at an angle of 45∘ with horizontal.There is a wall of 25m height at a distance of 10m from the projection point. The ball will hit the wall at a height of
View Solution
Knowledge Check
We know how by neglecting the air resistance, the problems of projectile motion can be easily solved and analysed. Now we consider the case of the collision of a ball with a wall. In this case the problem of collision can be simplified by considering the case of elastic collision only. when a ball collides with a wass we divide its velocity into two components, one perpendiclar to the wall and other parallel to the wall. If the collision is elastic then the perpendicular component of velocity of the ball gets reversed with the same magnitude.

The other parallel component of velocity will remain constant if wall is given smooth.
Now, let us take a problem. Three balls A,B, and C are projected from ground with same speed at same angle with the horizontal. The ball A,B and C collide with the wall during their flilght in air and all three collide perpendicularly with the wall as shown in figure.

If the total time taken by the ball A to fall back on ground is 4 seconds and that by ball B is 2 secons. Then, the maximum height attained by ball B from ground will beA15mB5mC3mD25mWe know how by neglecting the air resistance, the problems of projectile motion can be easily solved and analysed. Now we consider the case of the collision of a ball with a wall. In this case the problem of collision can be simplified by considering the case of elastic collision only. when a ball collides with a wass we divide its velocity into two components, one perpendiclar to the wall and other parallel to the wall. If the collision is elastic then the perpendicular component of velocity of the ball gets reversed with the same magnitude.

The other parallel component of velocity will remain constant if wall is given smooth.
Now, let us take a problem. Three balls A,B, and C are projected from ground with same speed at same angle with the horizontal. The ball A,B and C collide with the wall during their flight in air and all three collide perpendicularly with the wall as shown in figure.

The vertical component of velocity of balls wiht which they are projected isA20m/sB10m/sC10√3m/sDUndeterminableA ball is thrown upwards with a speed u from a height h above the ground.The time taken by the ball to hit the ground is
A√2h/gB√8h/gC√u2+2ghgDug+√2hg
A ball projected from the ground with speed 10m/s at an angle of 45∘ with horizontal. It collides with a wall at a distance 2 m from the point of projection and returns to its original position. If the coefficient of restitution between the ball and wall is 1/x, find x.
View SolutionA ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at a distance a from the point of projection and returns to its original position. Find the coefficient of restitution between the ball and the wall.
View SolutionA ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at some distance from the point of projection and returns on the same level. Coefficient of restitution between the ball and the wall is e, then time taken in path AB and maximum height attained by the ball is

View SolutionIf four balls A,B,C,D are projected with same speed at angles of 15∘,30∘,45∘and60∘ with the horizontal respectively, the two balls which will fall at the same place will be-
View SolutionA ball of mass 'm' collides with a wall with speed 'V' and rebounds on the same line with the same speed. If the mass of the wall is taken as infinite, then the work done by the ball on the wall is
View Solution
