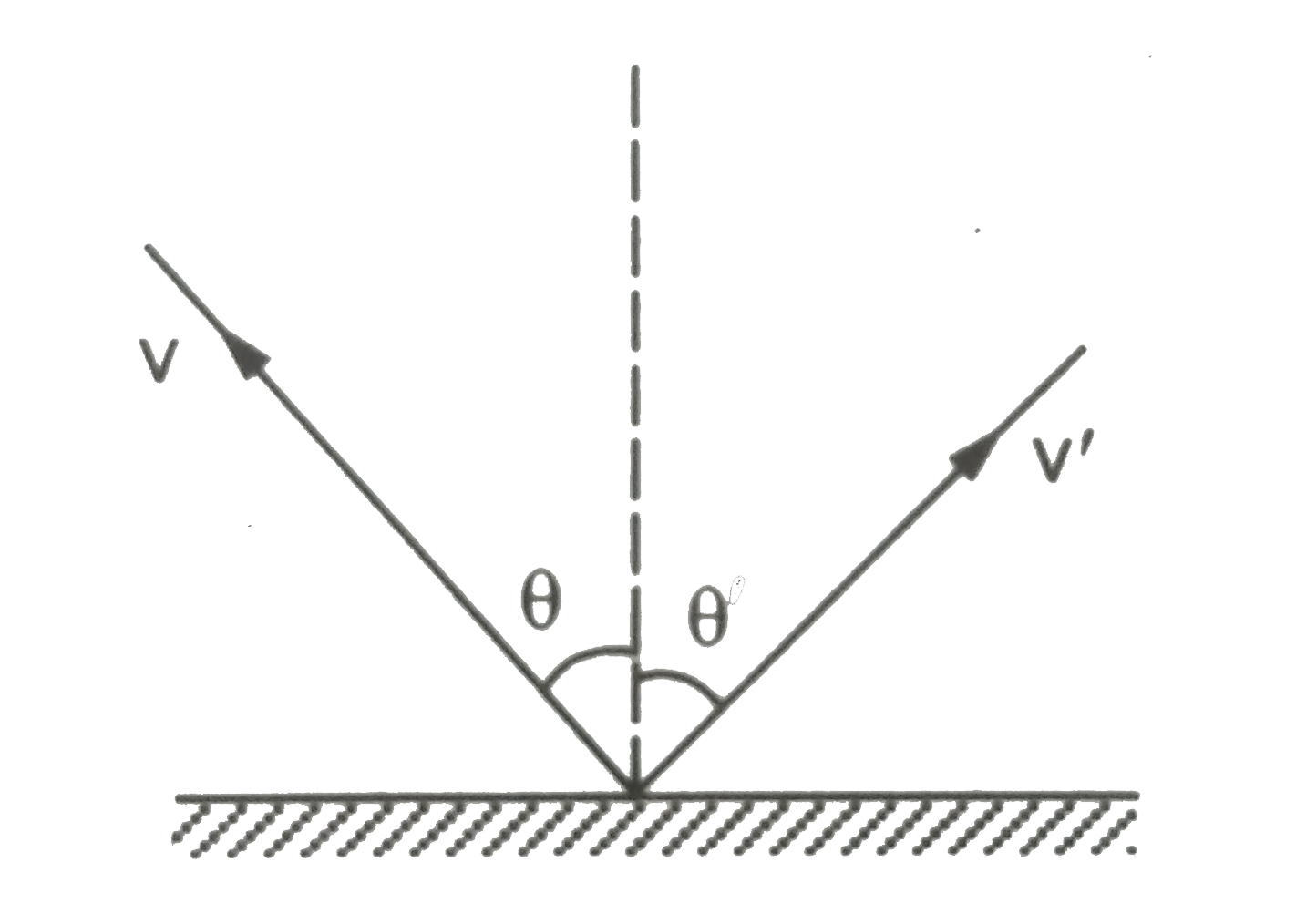
A particle of mass $m$ strikes a smooth floor with speed $u$ at angle of incidence $θ$ with the normal. The coefficient of resultant is $e$ . Find the magnitude and direction of velocity with which the particle rebounds. Also, find the impulse and loss in $K . E .$

Text Solution

Verified by Experts

Before the collision:
After the collision:
Since there is no force along the surface , hence the velocity component remains same.
$v sin α = u sin θ$ (i)
Due to the normal force between the particle and the floor , the velocity component becomes $e \times$ in opposite direction
$v cos α = e u cos θ$ (ii)
$v = \sqrt{{(v sin α)}^{2} + {(v cos α)}^{2}} = u \sqrt{{sin}^{2} θ + e^{2} {cos}^{2} θ}$
$tan α = \frac{v sin α}{v cos α} = \frac{1}{e} tan θ$
If the collision is elastic , $e = 1, v = u, α = θ$
$J_{x} = 0$
$J_{y} = m (v cos α + u cos θ)$
$= m (e u cos θ + u cos θ) = μ cos θ (1 + e)$
$J = μ cos θ (1 + e)$
$Δ K = \frac{1}{2} μ^{2} - \frac{1}{2} m u^{2} = \frac{1}{2} μ^{2} {cos}^{2} θ (1 - e^{2})$
$Δ$ K: loss in $K . E .$

Updated on:21/07/2023

A particle of mass $m$ strikes on ground with angle of incidence $45^{\circ}$ . If coefficient of restitution, $e = \frac{1}{\sqrt{2}}$ , find the velociy after impact and angle of reflection.
A ${tan}^{- 1} (2)$
B ${tan}^{- 1} (\frac{1}{\sqrt{2}})$
C ${tan}^{- 1} (\sqrt{2})$
D ${tan}^{- 1} (\frac{1}{2})$
A particle of mass, strikes on ground with angle of incidence $45^{\circ}$ , what will be the velocity after reflection if coefficient of restitution $e = \frac{1}{\sqrt{2}}$
A $\frac{\sqrt{2} v}{3}$
B $\frac{v}{\sqrt{3}}$
C $\frac{v}{2}$
D $\frac{\sqrt{3} v}{2}$