Question

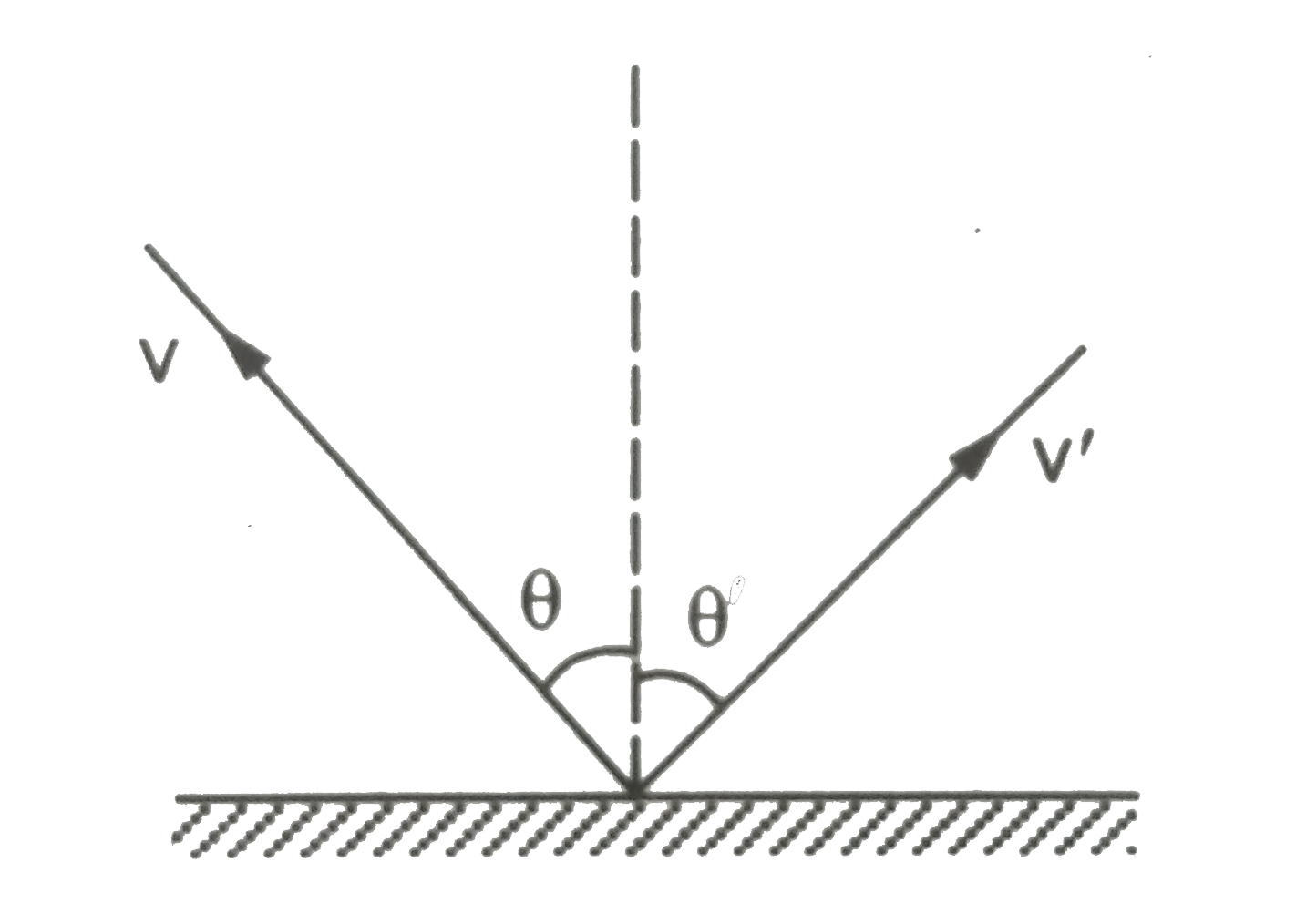
A ball of mass m hits a floor with a speed $v_0$ making an angle of incidence $\alpha$ with the normal. The coefficient of restitution is $e$. Find the speed of the reflected ball and the angle of reflection of the ball.

Answer

A::B

Explanation

To solve the problem, we will break down the motion of the ball into its components and apply the principles of collision and the coefficient of restitution.

1. Identify the Components of the Initial Velocity:
The ball hits the floor with an initial speed \( v_0 \) at an angle \( \alpha \) with the normal. We can break this velocity into two components:
- Normal Component (perpendicular to the floor):
\( v_{n} = v_0 \cos \alpha \)
- Tangential Component (parallel to the floor):
\( v_{t} = v_0 \sin \alpha \)

2. Apply the Coefficient of Restitution:
The coefficient of restitution \( e \) relates the velocities before and after the collision in the normal direction. The velocity of separation after the collision in the normal direction \( v_{n}' \) is given by:
\( e = \frac{v_{n}'}{v_{n}} \)
Rearranging gives:
\( v_{n}' = e v_{n} = e (v_0 \cos \alpha) \)

3. Determine the Tangential Component After Collision:
The tangential component of the velocity remains unchanged during the collision (assuming no friction). Therefore:
\( v_{t}' = v_{t} = v_0 \sin \alpha \)

4. Calculate the Magnitude of the Final Velocity:
The final velocity \( v \) of the ball after the collision can be found using the Pythagorean theorem:
\( v = \sqrt{(v_{n}')^2 + (v_{t}')^2} \)
Substituting the values we have:
\( v = \sqrt{(e v_0 \cos \alpha)^2 + (v_0 \sin \alpha)^2} \)
Simplifying this gives:
\( v = \sqrt{e^2 v_0^2 \cos^2 \alpha + v_0^2 \sin^2 \alpha} \)
\( v = v_0 \sqrt{e^2 \cos^2 \alpha + \sin^2 \alpha} \)

5. Determine the Angle of Reflection:
Let \( \beta \) be the angle of reflection with respect to the normal. The relationship between the tangential and normal components after the collision gives us:
\( \tan \beta = \frac{v_{t}'}{v_{n}'} = \frac{v_0 \sin \alpha}{e v_0 \cos \alpha} \)
This simplifies to:
\( \tan \beta = \frac{\sin \alpha}{e \cos \alpha} \)
Therefore, the angle of reflection \( \beta \) can be expressed as:
\( \beta = \tan^{-1}\left(\frac{\tan \alpha}{e}\right) \)

Final Results:
- The speed of the reflected ball is:
\( v = v_0 \sqrt{e^2 \cos^2 \alpha + \sin^2 \alpha} \)
- The angle of reflection is:
\( \beta = \tan^{-1}\left(\frac{\tan \alpha}{e}\right) \)