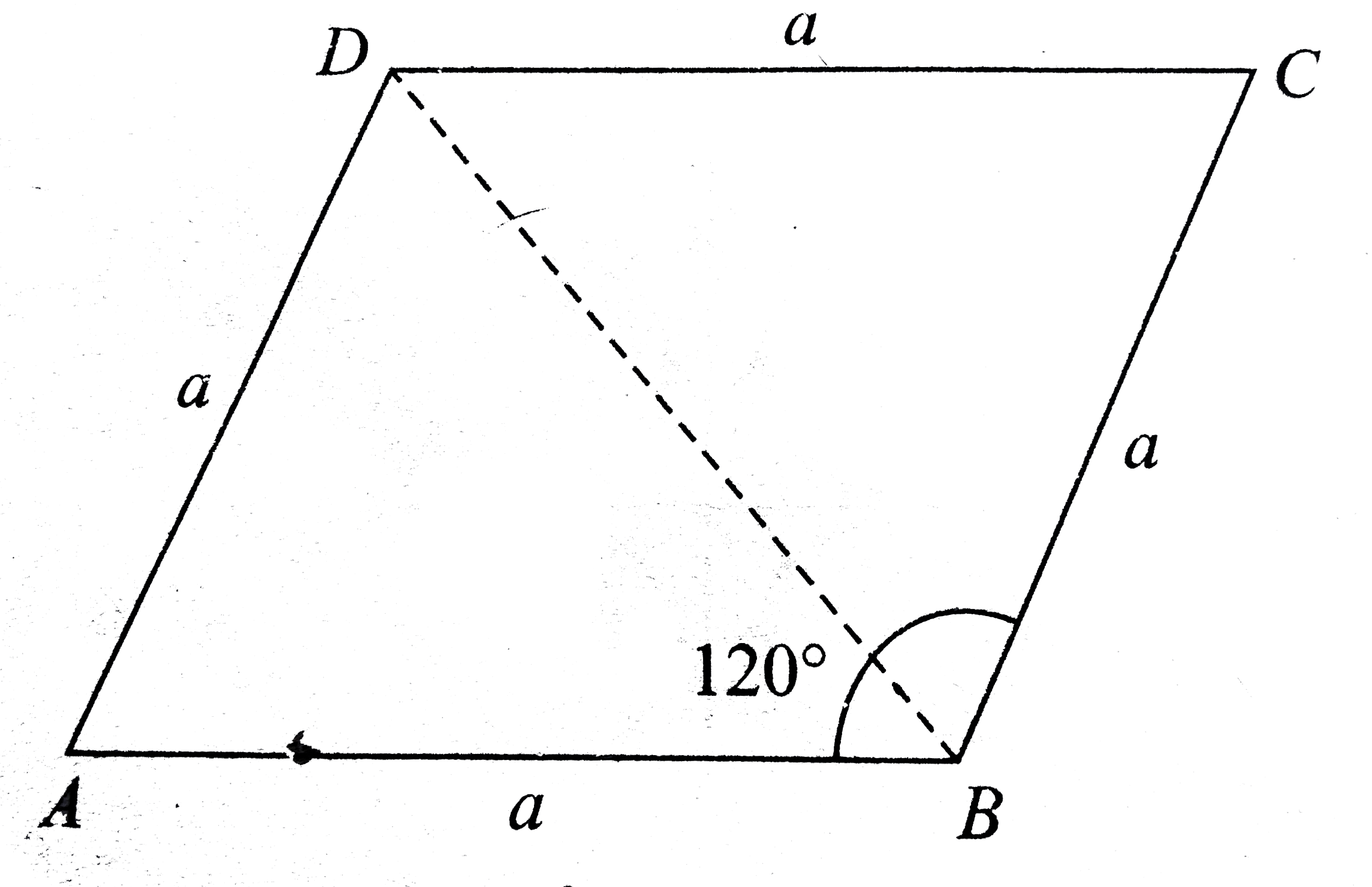
The longer side of a rectangle is twice the length of its shorter side

A cube of side x has a charge q at each of its vertices. Determine the potential due to this charge array at the center of the cube.
A $\frac{4 q}{3 π ε_{0} x}$
B $\frac{4 q}{\sqrt{3} π ε_{0} x}$
C $\frac{3 q}{4 π ε_{0} x}$
D $\frac{2 q}{\sqrt{3} π ε_{0} x}$
If the base of a triangle and the ratio of the lengths of the other two unequal sides are given, then the vertex lies on
A. straight line
Bcircle
Cellipse
Dparabola

The longer side of a rectangle is twice the length of its shorter side .A charge $q$ is kept at one vertex.The maximum electric potential due to that charge at any other vertex is $V$ , then the minimum electric potential at any other vertex will be