A cube of side b has a charge q at each of its vertices. Determine the potential and electric field due to this charge array at the center of the cube.
To solve the problem of determining the electric potential and electric field at the center of a cube with a charge q at each vertex, we can follow these steps:
Step 1: Determine the distance from the center to a vertex
The center of the cube is equidistant from all vertices. The distance from the center of the cube to any vertex can be calculated using the formula for the diagonal of a cube. The diagonal length d of the cube can be given by:
d=√3b
where b is the side length of the cube. The distance from the center to a vertex (let's denote this distance as r) is half of the diagonal:
r=d2=√3b2
Step 2: Calculate the potential at the center due to one charge
The electric potential V due to a point charge q at a distance r is given by the formula:
V=kqr
where k is the electrostatic constant. Substituting the value of r:
V=kq√3b2=2kq√3b
Step 3: Calculate the total potential at the center due to all charges
Since there are 8 charges (one at each vertex of the cube), the total potential Vtotal at the center of the cube is the sum of the potentials due to each charge:
Vtotal=8×V=8×2kq√3b=16kq√3b
Step 4: Determine the electric field at the center
To find the electric field E at the center of the cube, we need to consider the contributions from all the charges. The electric field due to a point charge q at a distance r is given by:
E=kqr2
However, due to the symmetry of the charge distribution (charges are placed symmetrically at the vertices), the electric fields due to opposite charges will cancel each other out. Therefore, the net electric field at the center of the cube is:
Etotal=0
Final Results
- The electric potential at the center of the cube is:
Vtotal=16kq√3b
- The electric field at the center of the cube is:
Etotal=0
The center of the cube is equidistant from all vertices. The distance from the center of the cube to any vertex can be calculated using the formula for the diagonal of a cube. The diagonal length d of the cube can be given by:
The electric potential V due to a point charge q at a distance r is given by the formula:
Since there are 8 charges (one at each vertex of the cube), the total potential Vtotal at the center of the cube is the sum of the potentials due to each charge:
To find the electric field E at the center of the cube, we need to consider the contributions from all the charges. The electric field due to a point charge q at a distance r is given by:
- The electric potential at the center of the cube is:
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - NCERT ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Exercise
37 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise
15 Videos
- MAGNETISM AND MATTERBOOK - NCERT ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - Exercise
25 Videos
Similar Questions
Knowledge Check
A cube of side x has a charge q at each of its vertices. Determine the potential due to this charge array at the center of the cube.
A4q3πε0xB4q√3πε0xC3q4πε0xD2q√3πε0xA hexagon of side 8 cm has a charge 4μC at each of its vertices. The potential at the centre of the hexagon is
A2.7×106VB7.2×1011VC2.5×1012VD3.4×104V
A point charge q is placed at the origin . How does the electric field due to the charge very with distance r from the origin ?
View SolutionGiven a cube with point charges q on each of its vertices. Calculate the force exerted on any of the charges due to rest of the 7 charges.
View SolutionEight equal point charges are placed at corners of a cube side length a. The potential and electric field magnitude at the centre of cube will be
View SolutionThe length of each side of a cubical closed surface is l If charge q is situated on one of the vertices of the cube , then find the electric flux passing through shaded face of the cube.
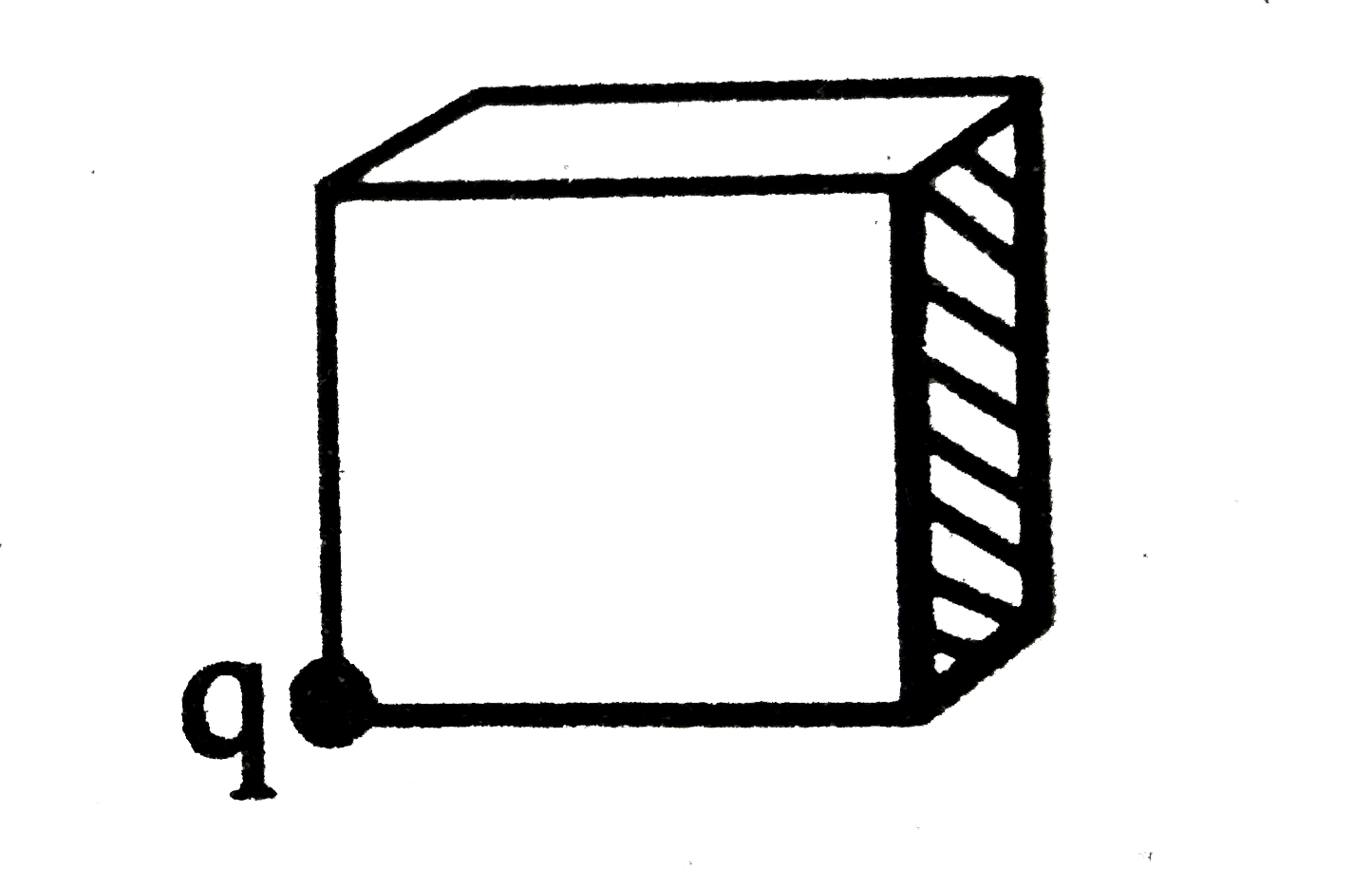 .
.
View SolutionA regular hexagon of side a has a charge Q at each vertex. Potential at the centres of hexagon is (k= (14πε0))
View SolutionA right isosceles triangle of side a has charges q, 3q and - q arranged on its vertices. What is the electric potential at P midway between the line connecting the + q and – q charges ?
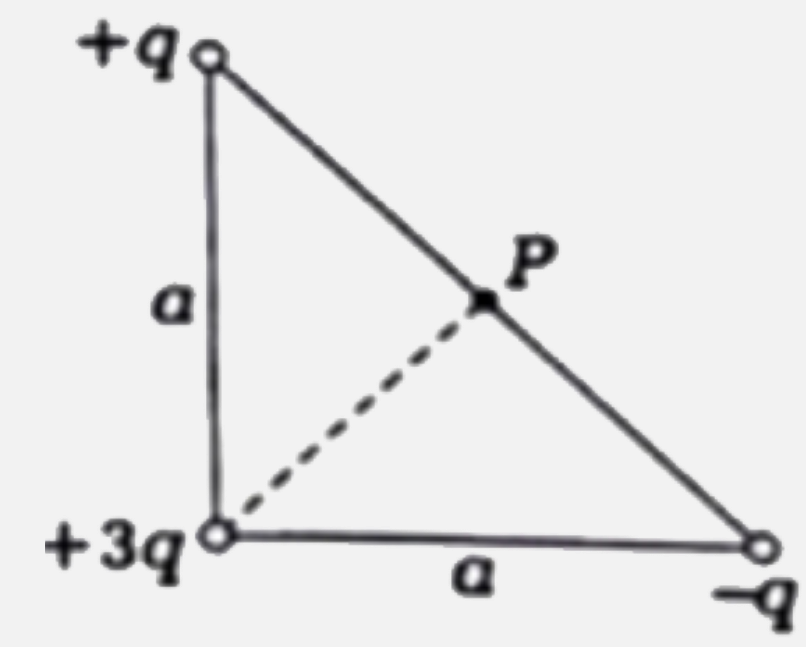
View Solution
