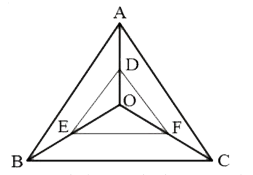
Any point $X$ inside $D E F$ is joined to its vertices. From a point $P$ in $D X, P Q$ is drawn parallel to $D E$ meeting $X E$ at $Q$ and $Q R$ is drawn parallel to $E F$ meeting $X F$ in $R$ . Prove that $P R D F$ .

Video Solution

Answer

Step by step video & image solution for Any point X inside D E F is joined to its vertices. From a point P in D X ,\ P Q is drawn parallel to D E meeting X E at Q and Q R is drawn parallel to E F meeting X F in R . Prove that P R D F . by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

SURFACE AREAS AND VOLUMESBOOK - RD SHARMACHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - All Questions
417 Videos
TRIGONOMETRIC IDENTITIESBOOK - RD SHARMACHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - All Questions
254 Videos

Knowledge Check

In $Δ P Q R$ , QR=10, RP=11 and PQ=12. D is the midpoint of PR, DE is drawn parallel to PQ meeting QR in E. EF is drawn parallel to RP meeting PQ in F. What is the length of DF?
A $\frac{11}{2}$
B6
C $\frac{33}{4}$
D5

Two triangle ABC and $D B C$ lie on the same side of the base $B C$ . From a point $P$ on $B C, P Q A B$ AND $P R B D$ ARE DRAWN. They meet $A C$ in $Q$ and $D C$ in $R$ respectively. Prove that $Q R A D .$
View Solution
$P$ is the mid-point of side $A B$ of a parallelogram $A B C D$ . A line through $B$ parallel to $P D$ meets $D C$ at $Q a n d A D$ produced at $R .$ Prove that: $A R = 2 B C$ (ii) $B R = 2 B Q$
View Solution
In Figure $A B C D and A E F D$ are two parallelograms. Prove that: $P E = F Q$
View Solution
$P Q R S$ is a parallelogram. $P X and Q Y$ are respectively, the perpendiculars from $P and Q$ to $S R and R S$ produced. Prove that $P X = Q Y$
View Solution
The side $A B$ of a parallelogram $A B C D$ is produced to any point $P .$ A line through $A$ parallel to $C P$ meets $C B$ produced in $Q$ and the parallelogram $P B Q R$ completed. Show that $a r (^(g m) A B C D) = a r (^(g m) B P R Q) .$ CONSTRUCTION : Join $A C$ and PQ. TO PROVE : $a r (^(g m) A B C D) = a r (^(g m) B P R Q)$
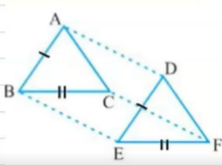
View Solution
If $A B C a n d D E F$ are two triangles such that $A B, B C$ are respectively equal and parallel to $D E, E F,$ then show that Quadrilateral $A B E D$ is a parallelogram Quadrilateral $B C F E$ is a parallelogram $A C = D F$ (iv) $A B C ≅ D E F$

View Solution
In a $A B C, E and F$ are the mid-points of $A C and A B$ respectively. The altitude $A P$ to $B C$ intersects $F E$ at $Q$ Prove that $A Q = Q P$
View Solution

RD SHARMA-TRIANGLES-All Questions

In Fig. if E F|| D C|| A Bdot Prove that (A E)/(E D)=(B F)/(F C) . (...
06:42
In Fig. if P Q|| B C and P R|| C D . Prove that (i) (A R)/(A D)=(A...
07:05
Any point X inside D E F is joined to its vertices. From a point P...
03:21
In Fig. 4.31, if (A D)/(D C)=(B E)/(E C) and /C D E=/C E D , prove ...
03:02
In Fig. 4.32, D E A Q and D F A R . Prove that E F Q R . (FIG...
02:22
In Fig. 4.33, (P S)/(S Q)=(P T)/(T R) and /P S T=/P R Q . Prove ...
02:46
In Fig. 4.34, A ,\ B and C are points on O P ,\ O Q and O R resp...
02:19
In a A B C , D and E are points on the sides A B and A C respectiv...
04:13
In a A B C , D and E are points on the sides A B and A C respectiv...
03:42
In a A B C , D and E are points on the sides A B and A C respectiv...
03:33
In a A B C , D and E are points on the sides A B and A C respectiv...
06:22
In a A B C ,\ \ D\ a n d\ E are points on the sides A B and A C re...
03:57
In a A B C ,\ \ D\ a n d\ E are points on the sides A B and A C re...
04:01
In a A B C , P and Q are point on the sides A B and A C respective...
05:39
In a A B C , D and E are points on A B and A C respectively such ...
07:25
In Fig. 4.35, state if P Q E F . (FIGURE)
01:46
M and N are points on the sides P Q and P R respectively of a P...
03:31
If D and E are points on sides A B and A C respectively of a A ...
01:53
In Fig. 4.41, A D is the bisector of /A . If B D=4c m , D C=3c m...
01:10
In Fig. 4.42, A D is the bisector of /B A C . If A B=10 c m . A ...
01:59

Home
Ask Doubt
Profile

Any point X inside DEF is joined to its vertices. From a point P in DX, PQ is drawn parallel to DE meeting XE at Q and QR is drawn parallel to EF meeting XF in R . Prove that PRDF .

Topper's Solved these Questions

Similar Questions

Knowledge Check

In ΔPQR, QR=10, RP=11 and PQ=12. D is the midpoint of PR, DE is drawn parallel to PQ meeting QR in E. EF is drawn parallel to RP meeting PQ in F. What is the length of DF?

RD SHARMA-TRIANGLES-All Questions

Any point $X$ inside $D E F$ is joined to its vertices. From a point $P$ in $D X, P Q$ is drawn parallel to $D E$ meeting $X E$ at $Q$ and $Q R$ is drawn parallel to $E F$ meeting $X F$ in $R$ . Prove that $P R D F$ .

In $Δ P Q R$ , QR=10, RP=11 and PQ=12. D is the midpoint of PR, DE is drawn parallel to PQ meeting QR in E. EF is drawn parallel to RP meeting PQ in F. What is the length of DF?