Suppose line segments AB and CD intersect at O in such a way that AO=OD and OB=OC. Prove that AC=BD but AC may not be parallel to BD
In △AOC and △ODB
OA=OD (Given)
OB=OC (Given)
∠AOC=∠DOB(Vertically Opposite Angle)
∴△AOC≅△BOD (SAS)
AC=BD (CPCT)
Also, ∠OAC=∠ODB (CPCT)
and ∠OCA=∠OBD(CPCT)
Thus, ∠OAC may not be equal to ∠OBD
∴AC may not be parallel to BD
However, if OA=OC then ∠OAC=∠OCA
Angles opposite to equal sides of △OAC but ∠OAC=∠ODB
∴∠OCA=∠ODB
But these angles form a pair of equal alternate angles.
∴AC parallel to BD
Hence proved.
Topper's Solved these Questions
Similar Questions
Diagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD)=ar ( BOC). Prove that ABCD is a trapezium.
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar(AOD)=ar(BOC) . Prove that ABCD is a trapezium.
View SolutionLine segments AB and CD intersect at O such that AC∣∣DB . If ∠CAB=350 and ∠CDB=550 find the ∠BOD.
View SolutionIn Figure, it is given that AB=BC and AD=EC. Prove that abe ≅CBD (ii) BD=BE
 View Solution
View SolutionLine segments AB and CD intersect at O such that AC∣∣DB. If ∠CAB=450and ∠CDB=550, then ∠BOD= 1000 (b) 800 (c) 900 (d) 1350
View SolutionThe diagonals of a parallelogram ABCD intersect at OA line through O intersects AB at XandDC at Y Prove that OX=OY
View SolutionABCD is a parallelogram whose AC and BD intersect at O. A line through O intersects AB at P and DC at Q. Prove that ar ( POA)= ar ( QOC).
View SolutionABCD is a trapezium with AB∣∣DC . A line parallel to AC intersects AB at X and BC at Y. Prove that ar(ADX)=ar(ACY).
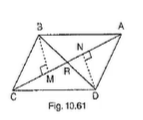
View SolutionIn Figure, BM and DN are both perpendiculars to the segments AC and BM=DN . Prove that AC bisects BD
 View Solution
View SolutionABC is a triangle. D is a point on AB such that D=14AB and E is a point on AC such that AE=14AC. Prove that DE=14BC
View Solution

