Prove that ABC is isosceles if any one of the following holds: (i) Altitude AD bisects BC (ii) Median AD is perpendicular to the base BC
To prove that triangle ABC is isosceles under the given conditions, we will consider each case separately.
Case 1: Altitude AD bisects BC
1. Given: AD is the altitude from vertex A to base BC, and it bisects BC.
- This means that BD = DC, where D is the foot of the altitude.
2. Triangles to Consider: We will consider triangles ABD and ACD.
- In triangle ABD:
- AD is the altitude, so angle ADB = 90°.
- In triangle ACD:
- AD is also the altitude, so angle ADC = 90°.
3. Common Sides: The side AD is common to both triangles ABD and ACD.
- Therefore, we have:
- AD = AD (common side)
4. Equal Segments: From the given, we know:
- BD = DC (since D bisects BC)
5. Angles: We have established:
- Angle ADB = Angle ADC = 90°
6. Applying SAS Criterion: We can now apply the Side-Angle-Side (SAS) criterion for triangle congruence:
- Triangle ABD ≅ Triangle ACD (by SAS)
7. Conclusion for Case 1: Since the triangles ABD and ACD are congruent, by the Corresponding Parts of Congruent Triangles are Equal (CPCTC), we have:
- AB = AC
- Thus, triangle ABC is isosceles.
Case 2: Median AD is perpendicular to base BC
1. Given: AD is the median from vertex A to base BC, and it is perpendicular to BC.
- This means that D is the midpoint of BC, so BD = DC.
2. Triangles to Consider: Again, we will consider triangles ABD and ACD.
- In triangle ABD:
- AD is the median, so BD = DC.
- In triangle ACD:
- AD is also the median, so BD = DC.
3. Common Sides: The side AD is common to both triangles ABD and ACD.
- Therefore, we have:
- AD = AD (common side)
4. Angles: Since AD is perpendicular to BC:
- Angle ADB = Angle ADC = 90°
5. Applying SAS Criterion: We can now apply the Side-Angle-Side (SAS) criterion for triangle congruence:
- Triangle ABD ≅ Triangle ACD (by SAS)
6. Conclusion for Case 2: Since the triangles ABD and ACD are congruent, by CPCTC, we have:
- AB = AC
- Thus, triangle ABC is isosceles.
Final Conclusion:
In both cases, we have shown that triangle ABC is isosceles if either condition is satisfied.
---
- This means that BD = DC, where D is the foot of the altitude.
- In triangle ABD:
- AD is the altitude, so angle ADB = 90°.
- In triangle ACD:
- AD is also the altitude, so angle ADC = 90°.
- Therefore, we have:
- AD = AD (common side)
- BD = DC (since D bisects BC)
- Angle ADB = Angle ADC = 90°
- Triangle ABD ≅ Triangle ACD (by SAS)
- AB = AC
- Thus, triangle ABC is isosceles.
- This means that D is the midpoint of BC, so BD = DC.
- In triangle ABD:
- AD is the median, so BD = DC.
- In triangle ACD:
- AD is also the median, so BD = DC.
- Therefore, we have:
- AD = AD (common side)
- Angle ADB = Angle ADC = 90°
- Triangle ABD ≅ Triangle ACD (by SAS)
- AB = AC
- Thus, triangle ABC is isosceles.
In both cases, we have shown that triangle ABC is isosceles if either condition is satisfied.
Topper's Solved these Questions
Similar Questions
Prove that ABC is isosceles if any one of the following holds: (i) Altitude AD bisects BC (ii) Median AD is perpendicular to the base BC
View SolutionA triangle ABC is an isosceles triangle if any one of the following conditions hold: Altitude AD bisects ∠BAC. Bisector of ∠BAC is perpendicular to the base BC.
View SolutionIf ABC is an isosceles triangle such that AB=AC and AD is an altitude from A on BC . Prove that (i) ∠B=∠C (ii) AD bisects BC (iii) AD bisects ∠A
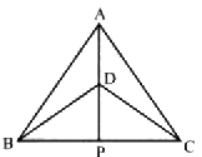
View SolutionABC and DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that ABD≅ACD (ii) ABP≅ACP AP bisects ∠A as well as ∠D AP is perpendicular bisector of BC.
 View Solution
View SolutionABC and DBC are two isosceles triangles on the same bas BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that AP bisects \ ∠A as well as ∠D AP is the perpendicular bisector of BC
View SolutionProve that A∪B=A∩B if and only if A=B If A={a,e,i,o,u},B={b,c,d} , then find (A−B).
View SolutionΔABCand ΔDBCare two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig. 7.39). If AD is extended to intersect BC at P, show that (i) ΔABD≅ΔACD
(ii) ΔABP≅ΔACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC
 View Solution
View SolutionProve that any two sides of a triangle are together greater than twice the median drawn to the third side. GIVEN : ABC in which AD is a median. PROVE : AB+AC>2AD CONSTRUCTION : Produce AD to E such that AD=DE. Join EC.
View SolutionIf the altitude from one vertex of a triangle bisects the opposite side, then the triangle is isosceles. GIVEN : A ABC such that the altitude AD from A on the opposite side BC bisects BC i.e., BD=DC. TO PROVE : AB=AC i.e. the triangle ABC is isosceles.
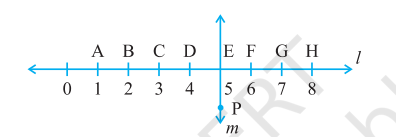
View SolutionStudy the diagram. The line l is perpendicular to line m (a) Is CE=EG (b) Does PE bisect CG = (c) Identify any two line segments for which PE is the perpendicular bisector. (d) Are these true? (i) AC>EG (ii) CD=GH (iii) BC<EH
 View Solution
View Solution

