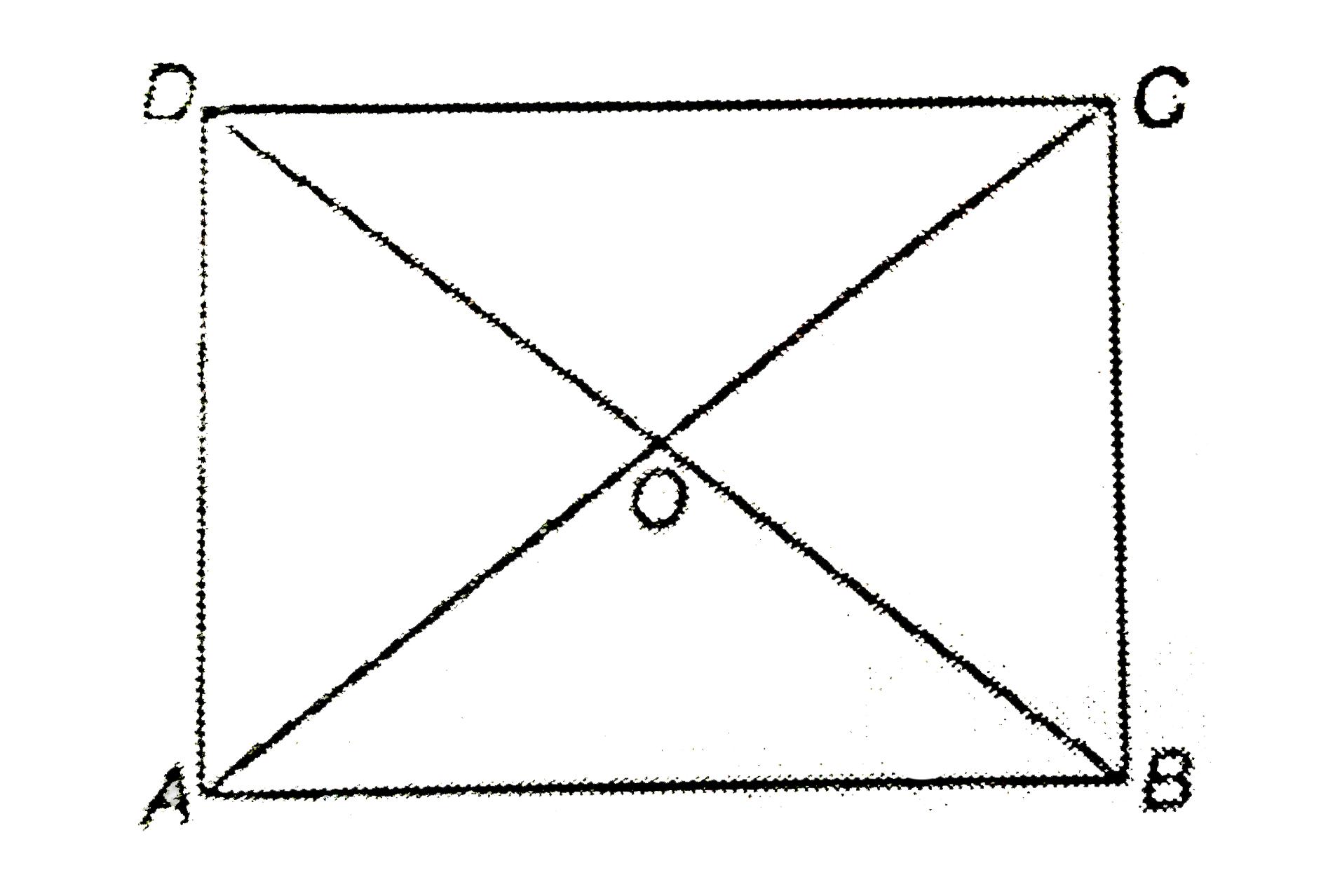
ABCD is a rectangle in which diagonal AC bisects ∠Aas well as ∠C. Show that:(i) ABCD is a square (ii) diagonal BD bisects ∠Bas well as ∠D..
Video Solution
Text Solution
(i)ABCD is a rectangle , in which diagonal AC bisect ∠A aswellas ∠C
Therefore,∠DAC=∠CAB(1)
∠DCA=∠BCA(2)
A square is a rectangle when all sides are equal.
Now,
AD∥BCandAC is transversal.
∠DAC=∠BCA (Alternate angles)
From (1),∠CAB=∠BCA(3)
In △ABC,∠CAB=∠BCA
BC=AB (4)(sides opposite to equal angles)
But BC=ADandAB=DC(5) (Opposite sides of rectangle)
Therefore from (4)& (5),AB=BC=CD=AD
Hence, ABCD is a square.
(ii)ABCD is a square and we know that diagonals of a square bisect its
angles.
Hence, BD bisects ∠Baswellas ∠D.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
If in a rectangle ABCD, diagonal AC bisect ∠A as well as ∠C then ABCD is a
AParallelogramBsquareCRhombusDTrapeziumThree statements are given below:
I. In a rectangle ABCD, the diagonal AC bisects ∠A as well as ∠C.
II. In a square ABCD, the diagonal AC bisects ∠A as well as ∠C.
III. In a rhombus ABCD, the diagonal AC bisects ∠A as well as ∠C.
Which is true?AI onlyBII and IIICI and IIIDI and IIABCD is a quadrlateral in which BD and AC are diagonals then
AAB+BC+CD+AD<AC+BDBAB+BC+CD+DA>AC+BDCAB+BC+CD+DA=AC+BDDAB+BC+CD+DA>2(AC+BD)
ABCD is a rhombus. Show that diagonal AC bisects ∠Aas well as ∠Cand diagonal BD bisects ∠B as well as ∠D.
View SolutionIf ABCD is a quadrilateral whose diagonals AC and BD intersect at O, then

View SolutionABC is an isoceless triangle in which AB= AC. AD bisects exterior ∠PAC and CD||AB. Show that (i) ∠DAC=∠BCA (ii) ABCD is a plarallelogram.
View SolutionABCD is a quadrilateral and BD is one of its diagonals as shown in Figure. Show that ABCD is a parallelogram and find its area.
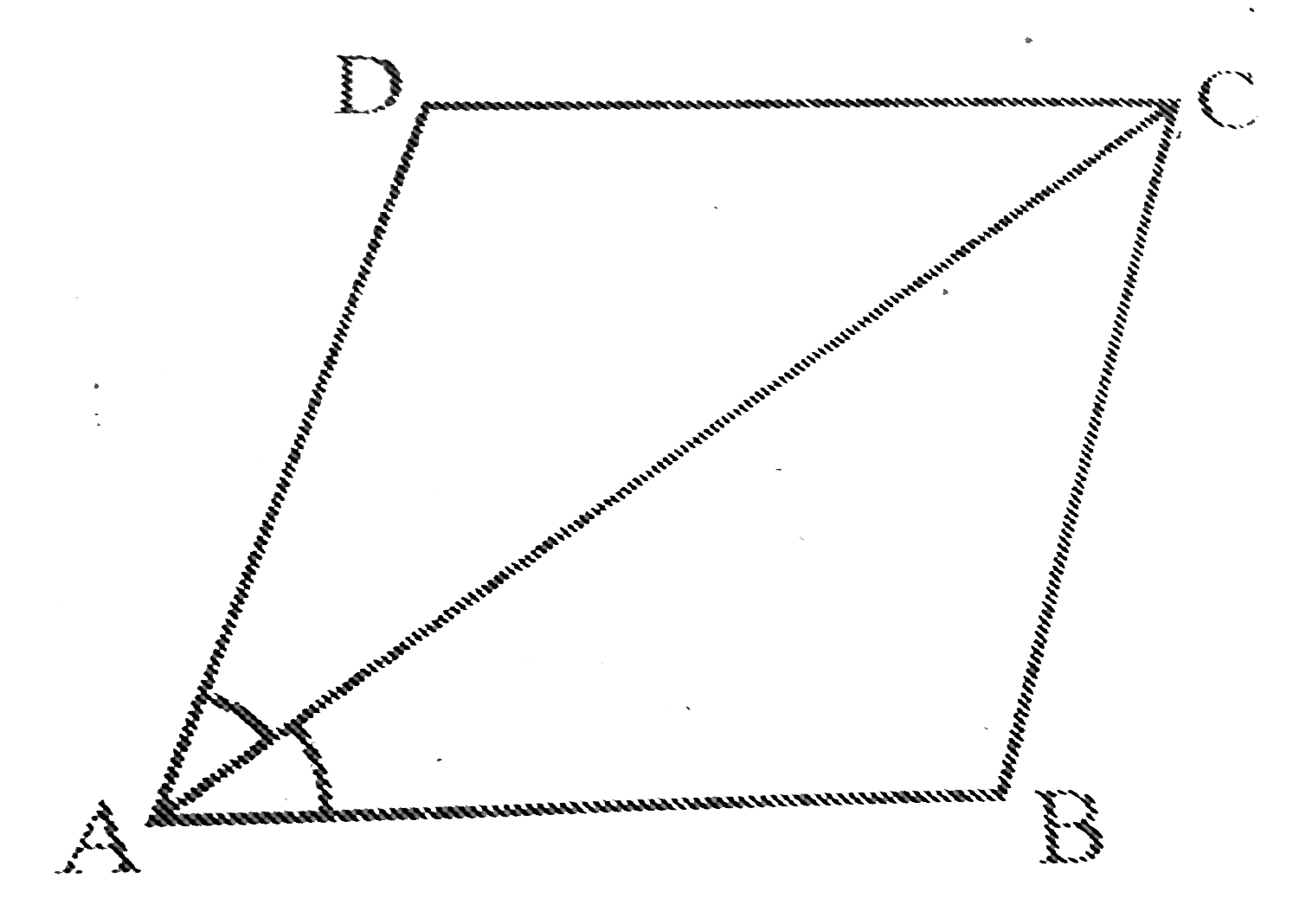
View SolutionDiagonal AC of a parallelogram ABCD bisects ∠A. Show that
(i) it bisects ∠C also,
(ii) ABCD is a rhombus.
View Solution