In hydrogen and hydrogen-like atom , the ratio of E4n−E2nandE2n−En varies with atomic nimber z and principal quantum numbern as
z2n2
z4n4
zn
none of these
The correct Answer is:D
E4n−E2nE2n−En=E116n2−E14n2E14n2−E1n2=14=constant
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS747videos
TEST PAPERS
- BOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)20videos
SIMPLE HARMONIC MOTION
- BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)5videos
WORK, ENERGY AND POWER
Similar Questions
Knowledge Check
- Question 1 - Select One
In hydrogen and hydrogen like atoms, the ratio of difference of energies E4n−E2n and E2n−En varies with its atomic number Z and n as
AZ2n2BZ4n4CZnDZ0n0 - Question 2 - Select One
The ratio of E2−E1 toE4−E3 for the hydrogen atom is approximately equal to -
A10B15C17D12 - Question 3 - Select One
According to the Bohr theory of Hydrogen atom, the speed of the electron, its energy and the radius of its orbit varies with the principal quantum number n, respectively, as
A1n,1n2,n2B1n,n2,1n2Cn2,1n2,n2Dn,1n2,1n2
Hydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
What is the principal quantum number, n' of the orbit of Be3 that has the same Bohr radius as that of ground state hydrogen atom ?
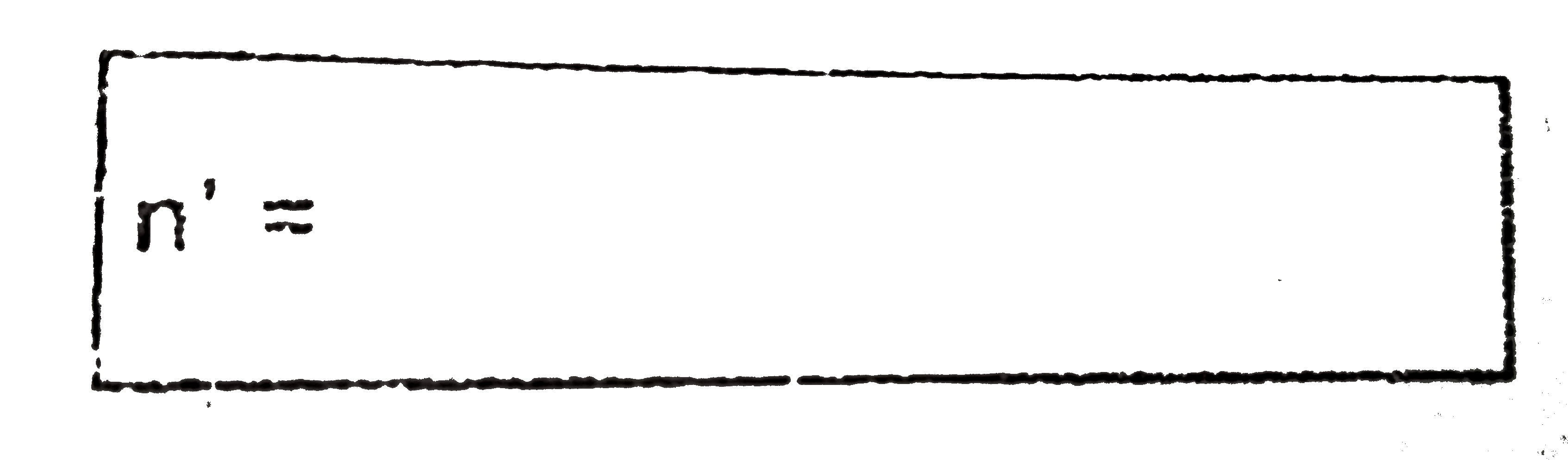 View Solution
View SolutionThe ratio of (E2−E1)to(E4−E3) for the hydrogen atom is approximately equal to.
View SolutionAccording to Bohr's theory, the time averaged magnetic field at the centre (i.e. nucleus) of a hydrogen atom due to the motion of electrons in the nth orbit is proportional to :
(n = principal quantum number)View SolutionAccording to Bohr's theory of the hydrogen atom , the speed vn of the electron in a stationary orbit is related to the principal quantum number nas (C is a constant):
View SolutionConsider the following statements
I Energies of the orbitals in hydrogen or hydrogen like species depend only on the quantum number 'n'
II Energies of the orbitals in multi-electron atoms depend on quantum numbers 'n' and 't'View Solution

