Consider an object of mass m moving in free space with velocity given by . v_(0) and omega are constants and t represents time. calculate force acting on object and angle between force and momentum.
(1) Let us calculate the force acting on it.
As we have
(2) Let us calculate the angle between force and momrntum .
As
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - TRY YOURSELF
67 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - ASSIGNMENT ( SECTION -A)
48 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTECHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTECHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
Consider a particle moving in the x−y plane according to r=r(cosωtˆi+sinωtˆj), where r and ω are constants. Find the trajectory, the velocity, and the acceleration.

View SolutionThe position vector of a particle is given by →r=kcosωˆi+ksinωˆj=xˆi+yˆj, where k and ω are constants and t time. Find the angle between the position vector and the velocity vector. Also determine the trajectory of the particle.
View Solution
Knowledge Check
A particle moves so that its position vector is given by →r=cosωtˆx+sinωtˆy, where ω is a constant which of the following is true ?
AVelocity is perpendicular to →r and acceleration is directed away from the origin.BVelocity and acceleration both are perpendicular to →r.CVelocity and acceleration both are parallel to →r.DVelocity is perpendicular to →r and acceleration is directed towards the origin.Position vector of a particle moving in x−y plane at time t is r=a(1−cosωt)ˆi+asinωtˆj. The path of the particle is
Aa circle of radius a and centre and (a,0)Ba circle of radius a and centre at (0,0)Can ellipseDNeither a circle nor an ellipseA particle is moving in a plane with velocity given by →u=u0ˆi+(aωcosωt)ˆj, where ˆi and ˆj are unit vectors along x and y axes respectively. If particle is at the origin at t=0. Calculate the trajectory of the particle :-
Ay=asin(u0ωx)By=asin(ωxu0)Cy=1a.sin(u0ωx)Dy=1a.sin(ωxu0)
A particle moves so that its position vector varies with time as →r=Acosωtˆi+Asinωtˆj. If d→rdt gives instantaneous velocity. Find the initial velocity of particle.
View SolutionA particle is moving in a plane with velocity →v=u0ˆi+kωcosωtˆj. If the particle is at origin at t=0, (a) determine the trajectory of the particle. (b) Find its distance from the origin at t=3π/2ω.
View SolutionA particle of mass m is moving with constant velocity v0 along the line y=b. At time t=0 it was at the point (0,b). At time t=bv0, the
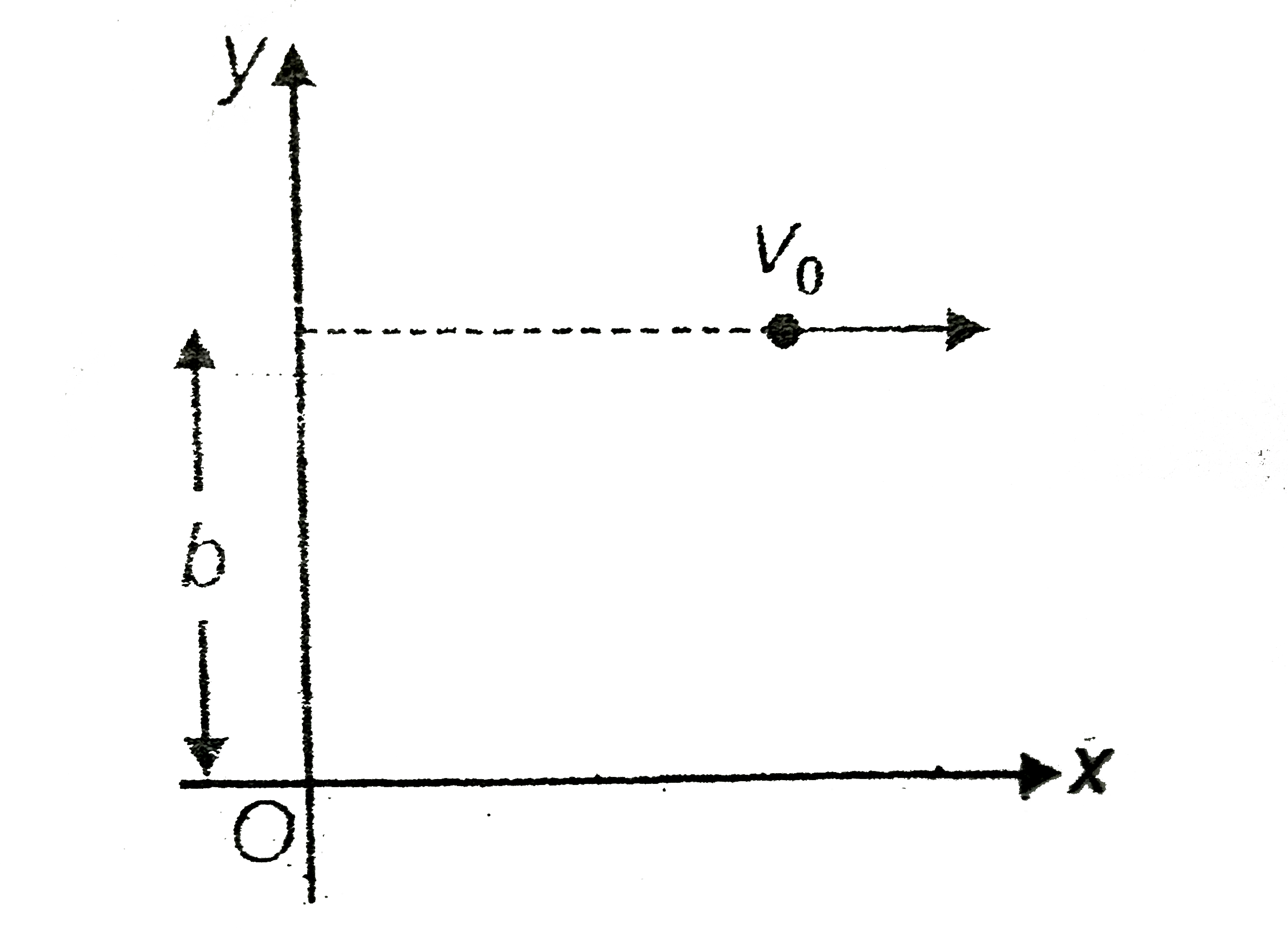
View SolutionThe velocity of a particle is given by v=v0sinωt, where v0 is constant and ω=2π/T. Find the average velocity in time interval t=0to t=T/2.
View SolutionA portion is fired from origin with velocity →v=v0ˆj+v0ˆk in a uniform magnetic field →B=B0ˆj. In the subsequent motion of the proton
View Solution
