Magnetic field in a space is given as B=−BoxRˆk. A circular ring of radius R having current I lies in the space with centre at origin as shown in the figure. Find the magnitude of net magnetic force acting on the circular ring due to the magnetic field.
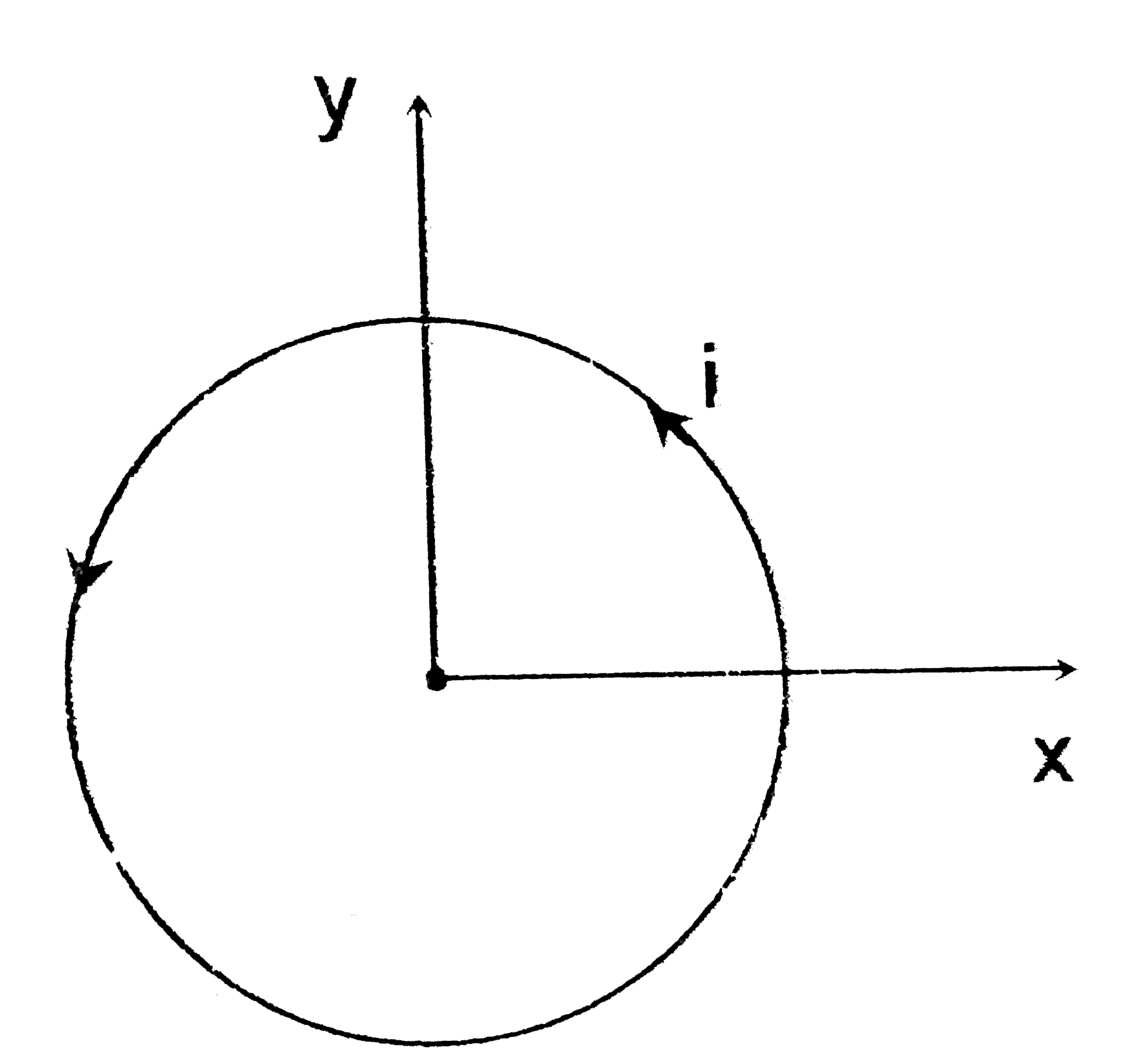
2iB0R
πiB0R
2πiB0R
xero
The correct Answer is:B
Fnet=∫force on idy
=4(idy)(B0R)(x)
=4iB0RR∫0√R2−y2dy
=πiB0R
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS747videos
TEST PAPERS
- BOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)20videos
SIMPLE HARMONIC MOTION
- BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)5videos
WORK, ENERGY AND POWER
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the magnitude of magnetic force on the frame, as shown. The magnetic field acting as shown:
 AZeroB2IB[a√2+(a2+(L−a)2}1/2]ILBC3 ILBDILB
AZeroB2IB[a√2+(a2+(L−a)2}1/2]ILBC3 ILBDILB - Question 2 - Select One
A part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is
 Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1)
Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1) - Question 3 - Select One
A semicircular wire of radius r, carrying a current I, is placed in a magnetic field of magnitude B. The force acting on it
Acan never be zeroBcan have maximum magnitude 2BIrCcan have the maximum magnitude BIπrDcan have the maximum magnitude BIr
Find the magnetic field at the centre of the circular loop shown in figure.
 View Solution
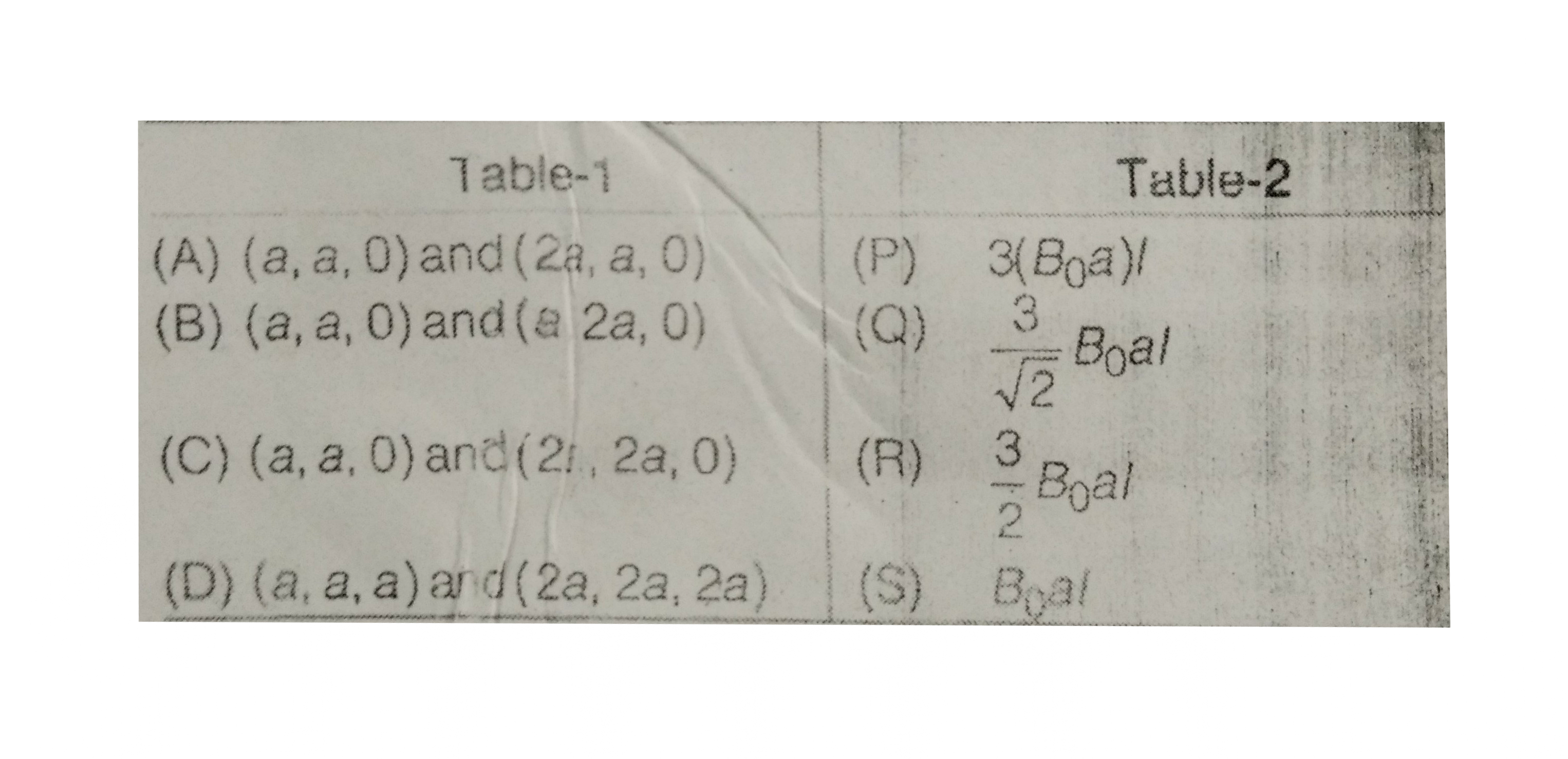
View SolutionMagnetic field exists in a space and given as B=−B0ayˆK, where B0 and a are constants. A wire PQ, having current I lies inside the magnetic field. If co-ordinates of P and Q are as given, then find the force acting on the wire as given in Table-2. Match the two Table.
 View Solution
View Solution(a) Draw the pattern of magnetic field lines for a circular coil carrying current.
(b) Two identical planes such that they have a common centre at P as shown in the figure. Find the magnitude and direction of the net magnetic field at the point P due to the loopsView SolutionA uniform but time-varying magnetic field B(t) exists in a circular region of radius a and is directed into the plane of the paper, as shown in the figure. The magnitude of the induced electric field at point P at a distance r from the centre of the circular region
 View Solution
View SolutionA circular coil of radius R carries a current i. The magnetic field at its centre is B. The distance from the centre on the axis of the coil where the magnetic field will be B/8 is
View Solution


