Question

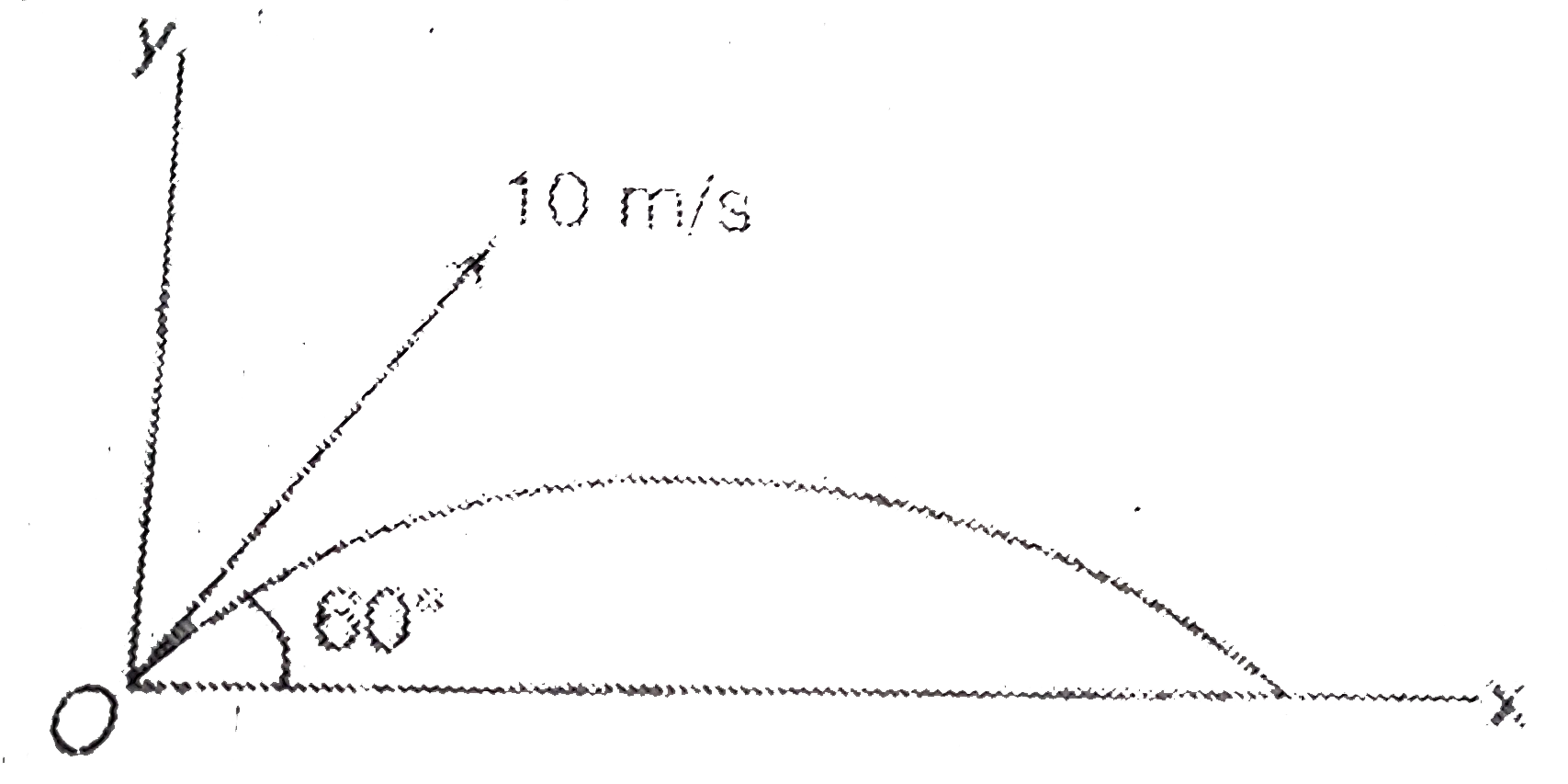
A heavy particle is projected from a point on the horizontal at an angle ${60}^{\circ }$ with the horizontal with a speed of $10m/s$. Then the radius of the curvature of its path at the instant of crossing the same horizontal is $\dots \dots \dots \dots \dots \dots \dots \dots ..$.

Answer

`20m`

Explanation

To find the radius of curvature of the path of a heavy particle projected at an angle of $${60}^{\circ }$$ with a speed of $$10\text{m/s}$$ at the instant it crosses the same horizontal, we can follow these steps:

Step 1: Understand the Motion
The particle is projected at an angle of \(60^\circ\) with the horizontal. The motion can be analyzed using projectile motion principles, where the horizontal and vertical components of the velocity can be calculated.

Step 2: Calculate the Initial Velocity Components
The initial velocity \(u\) is given as \(10 \, \text{m/s}\). We can find the horizontal (\(u_x\)) and vertical (\(u_y\)) components of the velocity:
- \(u_x = u \cdot \cos(60^\circ) = 10 \cdot \frac{1}{2} = 5 \, \text{m/s}\)
- \(u_y = u \cdot \sin(60^\circ) = 10 \cdot \frac{\sqrt{3}}{2} = 5\sqrt{3} \, \text{m/s}\)

Step 3: Determine the Velocity at the Horizontal Crossing
When the particle crosses the same horizontal level from which it was projected, its vertical component of velocity will be equal in magnitude but opposite in direction to its initial vertical component due to conservation of energy (ignoring air resistance). Therefore, the vertical component of velocity at this point is:
- \(v_y = -u_y = -5\sqrt{3} \, \text{m/s}\)

The horizontal component remains unchanged:
- \(v_x = u_x = 5 \, \text{m/s}\)

Step 4: Calculate the Magnitude of the Velocity
The total velocity \(v\) at the instant of crossing the horizontal can be calculated using the Pythagorean theorem:
\( v = \sqrt{v_x^2 + v_y^2} = \sqrt{(5)^2 + (-5\sqrt{3})^2} = \sqrt{25 + 75} = \sqrt{100} = 10 \, \text{m/s} \)

Step 5: Calculate the Normal Acceleration
The normal acceleration \(a_n\) at this point can be calculated using the gravitational acceleration \(g\) and the angle of projection:
\( a_n = g \cdot \cos(60^\circ) = 10 \cdot \frac{1}{2} = 5 \, \text{m/s}^2 \)

Step 6: Use the Formula for Radius of Curvature
The radius of curvature \(R\) can be calculated using the formula:
\( R = \frac{v^2}{a_n} \)
Substituting the values we have:
\( R = \frac{(10)^2}{5} = \frac{100}{5} = 20 \, \text{m} \)

Final Answer
The radius of curvature of the particle's path at the instant of crossing the same horizontal is \(20 \, \text{m}\).

---