A radio nuclide with half life $T = 69.31$ second emits $β$ -particles of average kinetic energy $E = 11.25 e V$ . At an instant concentration of $β$ -particles at distance, $r = 2 m$ from nuclide is $n = 3 \times 10^{13}$ per $m^{3}$ .
(i) Calculate number of nuclei in the nuclide at that instant.
(ii) If a small circular plate is placed at distance $r$ from nuclide such that $β$ -particles strike the plate normally and come to rest, calculate pressure experienced by the plate due to collision of $β$ -particle. (Mass of $β$ -particle $= 9 \times 10^{- 31} k g$ ) $({log}_{e} 2 = 0.693)$

Text Solution

Verified by Experts

The correct Answer is:(i) $9.6 π \times 10^{22}$
(ii) $1.08 \times 10^{- 4} N m^{- 2}$

Let activity (rate of decay) of the nuclide be $A$ nuclei per second. It means $A β$ -particles are emitted per second. If a spherical surface of radius $r$ with centre at position of nuclide be considered then $A β$ -particle cross this surface per second. It means during an elemental time interval $d t$ a number $(A . d t)$ of $b$ -particle cross this surface. If velocity of $β$ -particles be $v$ then above calculated $(A . d t) β$ -particle are in a space having shape of a spherical shell of radius $r$ and radial thickness $(v d t)$ as shown in figure.

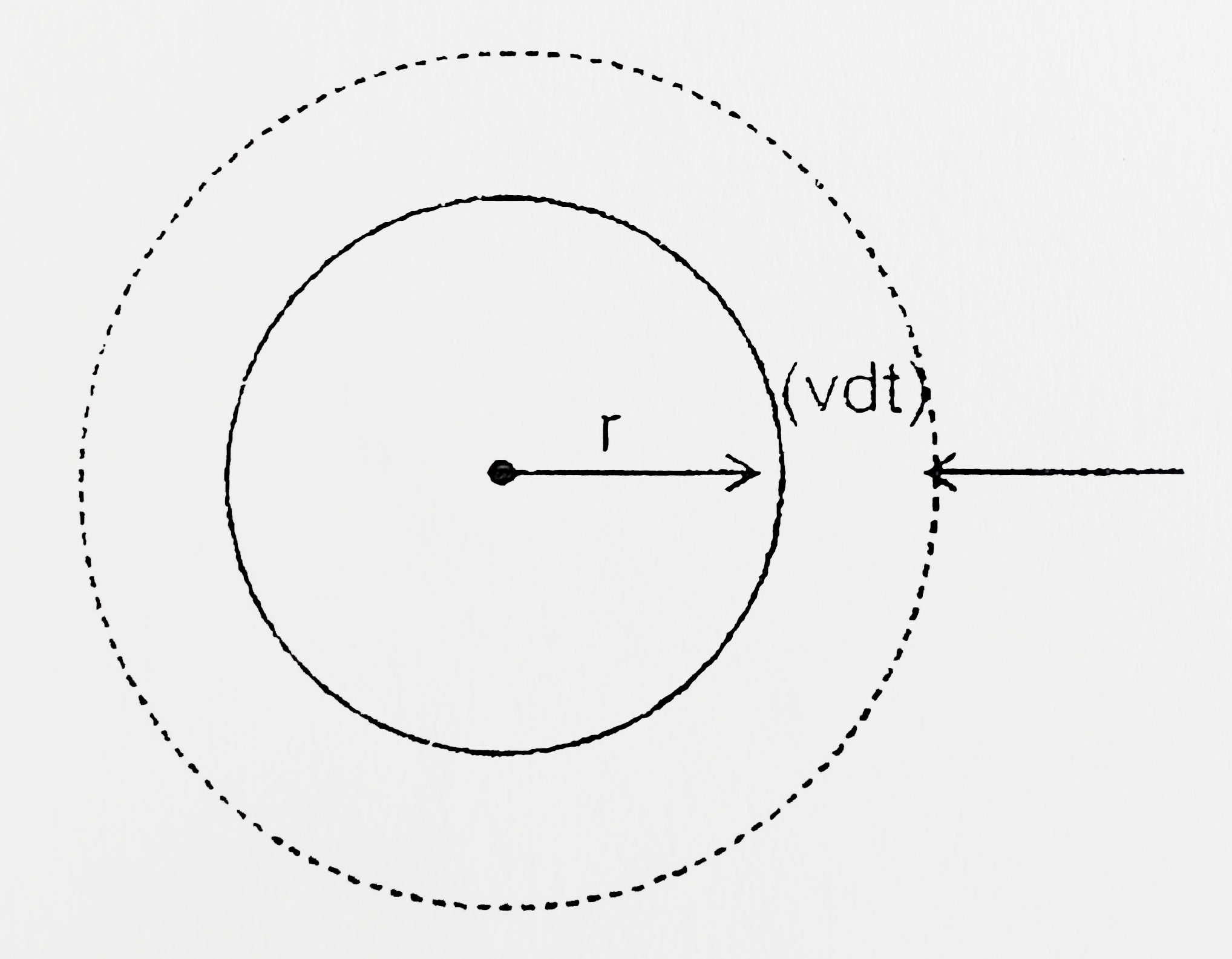
Volume of this space $= 4 π r^{2} (v d t)$
$∴$ Concentration of $b$ -particles at distance $r$ from nuclide is
$n = \frac{A d t}{4 π^{2} (v d t)}$
or activity of the nuclide, $A = 4 π r^{2} v n$
But activity, $A = λ N$ where $N$ is number of nuclei
Hence, $N = \frac{4 π r^{2} v n}{λ}$ but decay constant $λ = \frac{log 2}{T}$
$N = \frac{4 π r^{2} v n T}{0.6931}$ ........(1)
Kinetic energy of $β$ -particle $E = \frac{1}{2} m v^{2}$
$v = \sqrt{\frac{2 E}{m}}$
substituting this value in equation (1) , $N = \frac{4 π r^{2} n T}{0.6931} \sqrt{\frac{2 E}{m}} = 9.6 π \times 10^{22}$
(ii) At distance $r$ from the nuclide $(A / 4 π r^{2}) β$ -particle cross unit area per second. Let area of small circular plate be $S$ , then
number of $β$ -particle striking the plate per second $= \frac{A}{4 π r^{2}} S = \frac{λ N}{4 π r^{2}} S = \frac{0.6931 N S}{4 π r^{2} T}$
Momentum of each particle just before collision is $m v$ and after collision particles come to rest or momentum becomes Zero.
$∴$ Momentum transferred to plate due to collision is
$Δ p = m v - 0 = m v$
Due to transfer of momentum, the plate experiences a force which is equal to rate of transfer of momentum.
$∴$ Force, $F = Δ p \times no .$ of particles striking per second
or $F = m v \times \frac{0.6931 N S}{4 π r^{2} T}$
Pressure, P= Force per unit area
$∴ P = \frac{F}{S} = \frac{0.6931 N}{4 π r^{2} T} m v$ but $v = \frac{\sqrt{2 E}}{m}$
$∴ P = \frac{0.6931 N}{4 π r^{2} T} \sqrt{2 m E} = 1.08 \times 10^{- 4} N m^{- 2}$

Updated on:21/07/2023

|

Class 12 PHYSICS NUCLEI

Topper's Solved these Questions

Similar Questions

Knowledge Check

Nuclides
AHave specific atomic number
BHave same number of protons
CHave specific atomic number and mass numbers
DAre isotopes
n a sample of radioactive nuclide
Aa nucleus emits, $α, β$ radiations simultaneously.
Bonly $α, β$ can be emitted simultaneously by a nucleus.
C $α, β, γ$ may be obtained simultaneously from the sample
Dall the three $α, β, γ$ one after other will obtained from a nucleus
A nuclide at rest emits an alpha-particle. In this process:
Aalpha-particle moves with large velocity and the nuclesus remains at rest
Bboth alpha-particle and nucleus move with equal speed in opposite direction
Cboth move in opposite direction but nucleus with greater speed
Dboth move in opposite direction but alpha-particle with greater speed.

RESONANCE-NUCLEAR PHYSICS-Advanced level solutions

Home
Ask Doubt
Profile