Figure shows a concave mirror of focal length 20 cm. A point object P is oscillating along te principle axis of the mirror. Position co-ordinates of image at any time t is given by:
xi=40+20sin(2t+5π6)
Here x is in cm and t is in positive.
Speed of the object at t=0 is 40√3cm/s
speed of the object at t=0 is 80√39cm/s
if we start observing at t=0 minimum time t at which object & image will cross each other is π12 s
if we start observing at t=0, minimum time t at which object & imega will cross each other is π6s
The correct Answer is:B, C
At t=0 image distance v=−50cm
velocity of image VI=−20√3cm/s
VI=−m2.v0
20√3=(f−vf)2V0
20√3=−(−20+50−20)2V0
20√3=−94V0
V0=80√39cm/s
Object & image will cross each other at centre of curvature i.e., when xi=40
2t+5π6=π
2t+π6⇒t=π42s
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PT-01
30 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - Pt-02
30 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
107 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCECHAPTER - TEST SERIESEXERCISE - PHYSICS
127 Videos
Similar Questions
A 0.2 cm high object is placed 15 cm from a concave mirror of focal length 5 cm. Find position and size of the image.
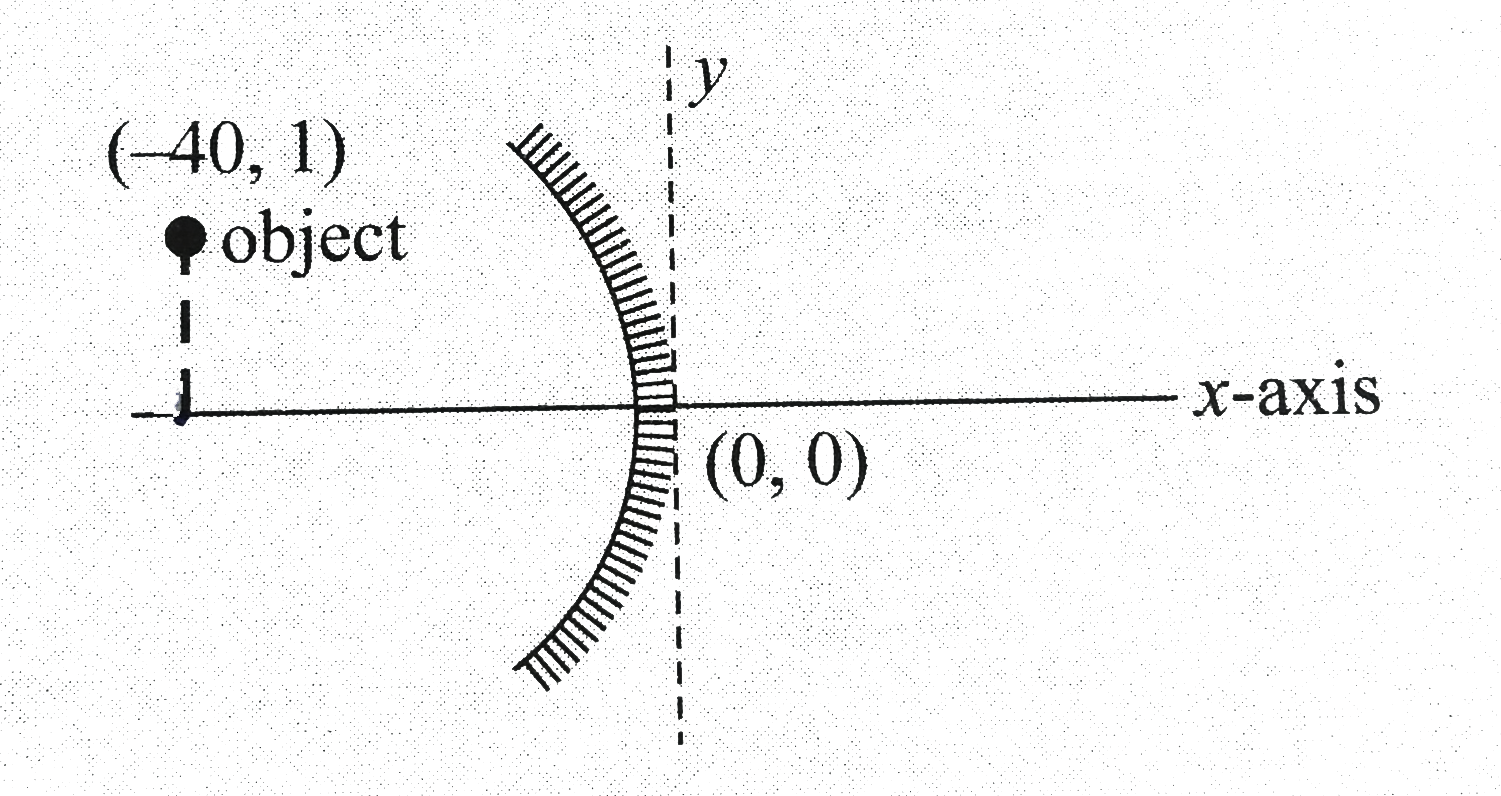
View SolutionFigure shows a shperical concave mirror of focal length f=5cm with its pole at (0,0) and principal axis along x-axis. There is a point object at (-40cm, 1cm), find the postion of image.
View Solution
Knowledge Check
A concave mirror has a focal length 20 cm. The distance between the two positions of the object for which the image size is double of the object size is
A20 cmB40 cmC30 cmD60 cmAn object is 20 cm away from a concave mirror with focal length 15 cm. If the object moves with a speed of 5 m/s along the axis, then the speed of the image will be
A45 m/sB27 m/sC9 m/sD10 m/sA point object at 15 cm from a concave mirror of radius of curvature 20 cm is made to oscillate along the principal axis with amplitude 2 mm. The amplitude of its image will be
A2mmB4mmC8mmDNone of these
A body of length 6 cm is placed 10cm from a concave mirror of focal length 20cm. Find the position, size, and nature of the image.
View SolutionA point object on the principal axis of a concave mirror of focal length 20 cm, is moving at a speed of 5 cm/s at an single 45∘ to the principal axis as shown of 25 cm from the pole of mirror.Find the velocity components of image along and normal to principal axis at this instant
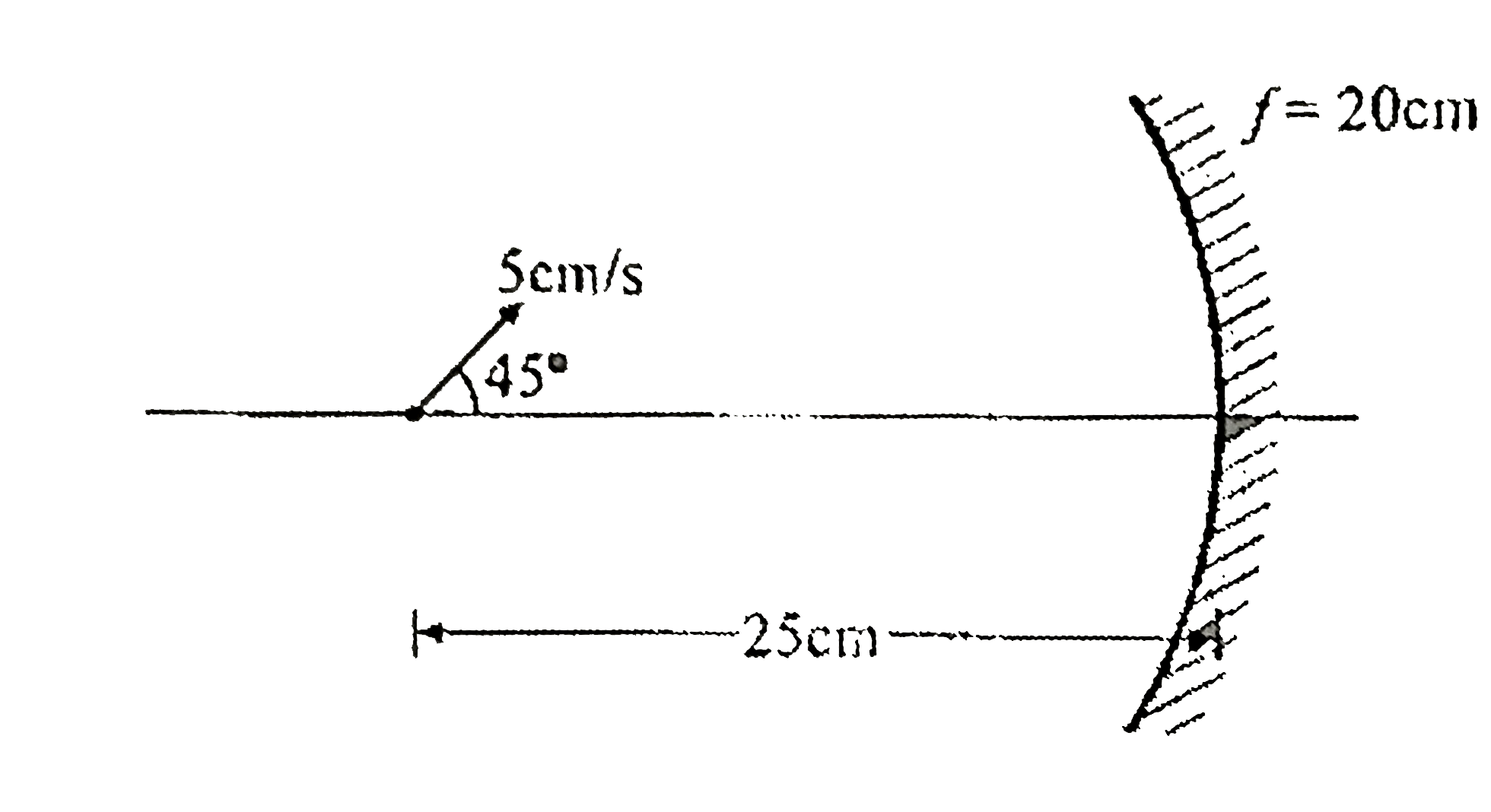
View SolutionAn object is kept 40 cm in front of a concave mirror of focal length of 20 cm. Find the position and nature of the image. Draw a ray diagram to show the formation of image in this case.
View SolutionA 0.5 cm high object is placed at 30 cm from a convex mirror whose focal length is 20 cm. Find the position and size of the image.
View SolutionA concave mirror has a focal length of 20 cm. Find the position or positions of an object for which the image-size is double of the object-size.
View Solution
