A uniform tube of volume V contains an ideal monatomic gas at a uniform pressure P the temperature of the gas varies linearly along te length of the tube ad T and 2 T are the temperature at the ends.
The number of moles of the gas in the tube is PVRTln2
The number of moles of the gas in the tube is PVRTln4
The internal energy of the gas in the tube is (3/2)PV
The internal energy of the gas in the tube is (3/2)nRT.
The correct Answer is:A, C

T′=T+TlξmpliesdT′=Tldx
n=∫dn=∫PadxRT′=∫2TTPVRTdT′T′
n=PVRTln2
U=f2PV
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PT-0130videos
TEST PAPERS
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - Pt-0230videos
TEST PAPERS
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS107videos
TEST PAPERS
- BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems13videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCECHAPTER - TEST SERIESEXERCISE - PHYSICS127videos
TEST SERIES
Similar Questions
An ideal gas has pressure P, volume V and temperature T. Select the correct option.
View SolutionA cylindrical tube of cross sectional area A and length L is filled with an ideal gas. Temperature of the gas varies linearly from T0 at one end to2T0 at the other end. Calculate the number of mole of the gas in the tube assuming pressure to be uniform throughout equal to P.
View Solution
Knowledge Check
- Question 1 - Select One
An insulated container of gas has two chambers separated by an insulating partition. One of the chmabers has volume V1 and contains ideal gas at pressure P1 and temperature T1.The other chamber has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partition is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
A(T1T2P1V1+P2V2P1V1T2+P2V2T1BP1V1T1+P2V2T2P1V1+P2V2CP1V1T2+P2V2T1P1V1+P2V2DT1T2(P1V1+P2V2)P1V1T1+P2V2T2 - Question 2 - Select One
If 2 mol of an ideal monatomic gas at temperature T0 are mixed with 4 mol of another ideal monoatomic gas at temperature 2T0 then the temperature of the mixture is
A53T0B32T0C43T0D54T0 - Question 3 - Select One
A vessel of volume V contains an ideal gas at absolute temperature T and pressure P. The gas is allowed to leak till its pressure falls to P'. Assuming that the temperature remains constant during leakage, the number of moles of the gas that have leaked is
AVRT(P+P')BV2RT(P+P')CVRT(P−P')DV2RT(P−P')
Two closed bulbs of equal volume (V) containing an ideal gas initially at pressure Pi and temperature T1 are connected through a narrow tube of negligible volume as shown in the figure below. The temperature of one of the bulbs is then raised to T2. The final pressure Pf is :
 View Solution
View SolutionAn insulated container of gas has two chambers separated by an insulating partition. One of the chambers has volume V1 and contains ideal gas at pressure P1 and temperature T1. The other chambers has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partion is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
View SolutionTwo adiabatic containers have volumes V1 and V2 respectively. The first container has monoatomic gas at pressure p1 and temperature T1. The second container has another monoatomic gas at pressure p2 and temperature T2. When the two containers are connected by a narrow tube, the final temperature and pressure of the gases in the containers are P and T respectively. Then
View SolutionThe specific heat of an ideal gas varies with temperature T as
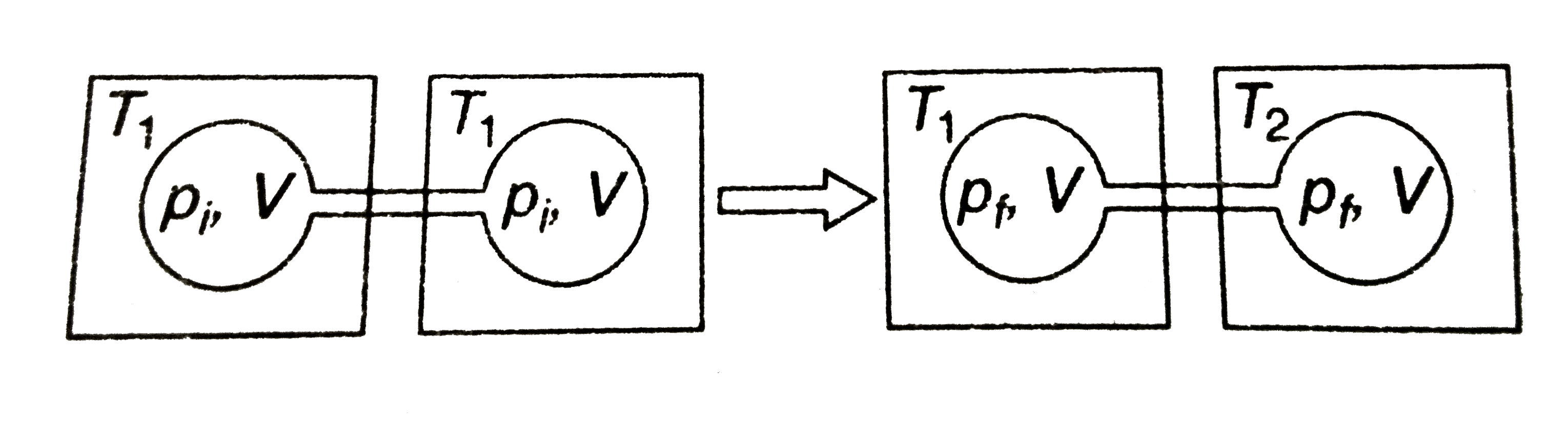
View SolutionTwo closed bulb of euqal volume (V) containing an ideal gas initially at pressure pi and Temperature T1 are connected through a narrow tube of negligible volume as shown in the figure below. The temperature of one of the bulbs is then released to T2. The final pressure pt is :
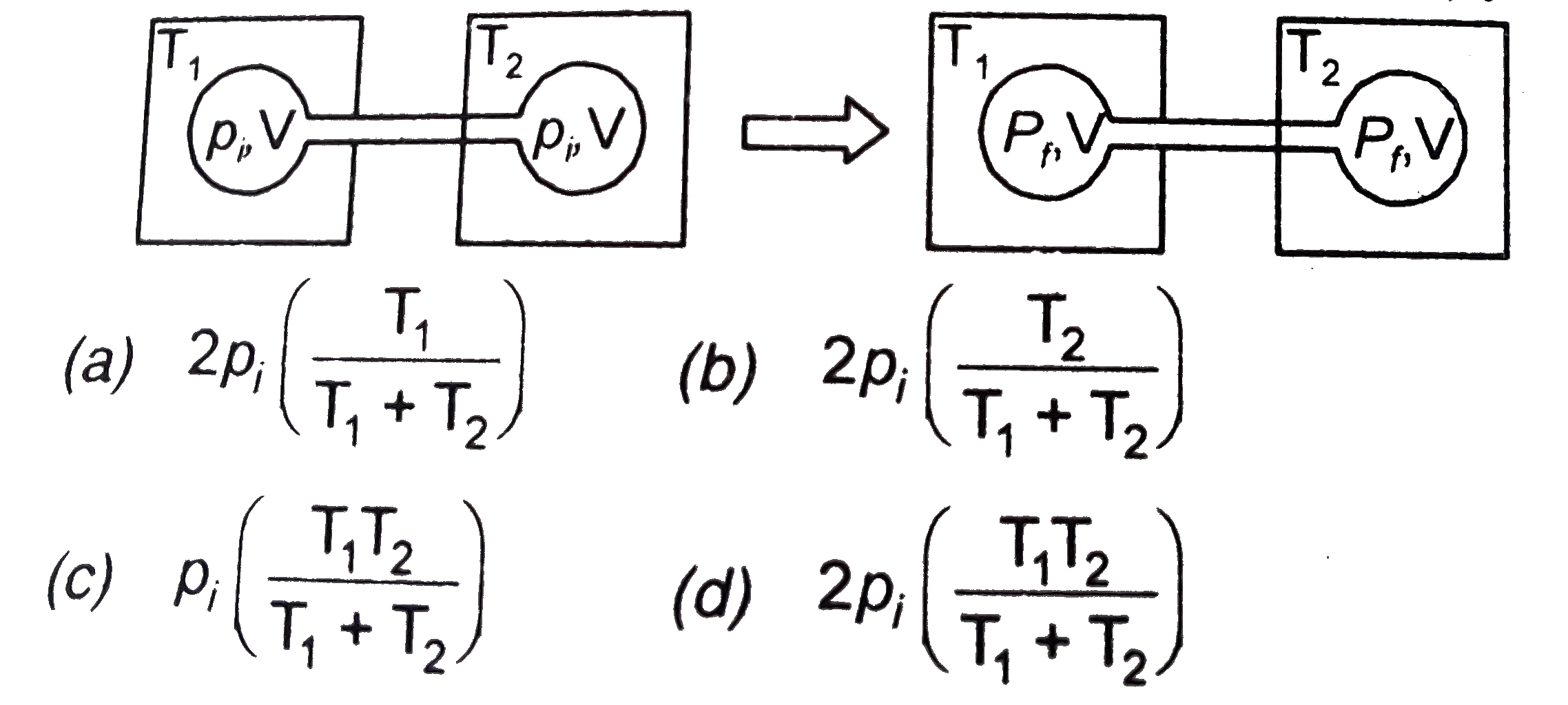 View Solution
View Solution

