If a=3√t and b=t2, then ba5=
t−13
t13
t56
t65\
The correct Answer is:B
To solve the problem, we need to find the value of ba5 given that a=√(3)t and b=t2.
1. Identify the values of a and b:
- We have a=√(3)t and b=t2.
2. Substitute the values of a and b into the expression ba5:
- Substitute b with t2 and a with √(3)t:
ba5=t2(√(3)t)5
3. Simplify a5:
- Recall that √(3)t=t1/3, thus:
a5=(t1/3)5=t5/3
4. Rewrite the expression:
- Now we can rewrite the expression:
ba5=t2t5/3
5. Apply the laws of exponents:
- When dividing like bases, we subtract the exponents:
t2t5/3=t2−5/3
6. Convert 2 to a fraction with a common denominator:
- Convert 2 to a fraction:
2=63
- Now substitute:
t2−5/3=t63−53=t13
7. Final Result:
- Therefore, the final result is:
ba5=t13
- We have a=√(3)t and b=t2.
- Substitute b with t2 and a with √(3)t:
ba5=t2(√(3)t)5
- Recall that √(3)t=t1/3, thus:
a5=(t1/3)5=t5/3
- Now we can rewrite the expression:
ba5=t2t5/3
- When dividing like bases, we subtract the exponents:
t2t5/3=t2−5/3
- Convert 2 to a fraction:
2=63
- Now substitute:
t2−5/3=t63−53=t13
- Therefore, the final result is:
ba5=t13
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If x=t2andy=t3 , then d2ydx2 is equal to: a) 32 b) 32t c) 32t d) 34t
A32B32tC32tD34t
If A is a nonsingular matrix such that AAT=ATA and B=A−1AT, then matrix B is
View SolutionIf u=x2andx=s+3t,y=2s−t, then d2uds2 is (a) 52t (b) 20t8 (c) 516t6 (d) non of these
View SolutionIf x=1+tt3,y=32t2+2t, then x(dydx)3−dydx is equal to (a)0 (b) −1 (c)1(d)2
View SolutionIf x=at2, y=2 at , then d2ydx2= −1t2 (b) 12 at3 (c) −1t3 (d) −12 at3
View SolutionIf tension in string A and string B are TA and TB then find out TATB (assume that stringe are light and g=10m/s2) :
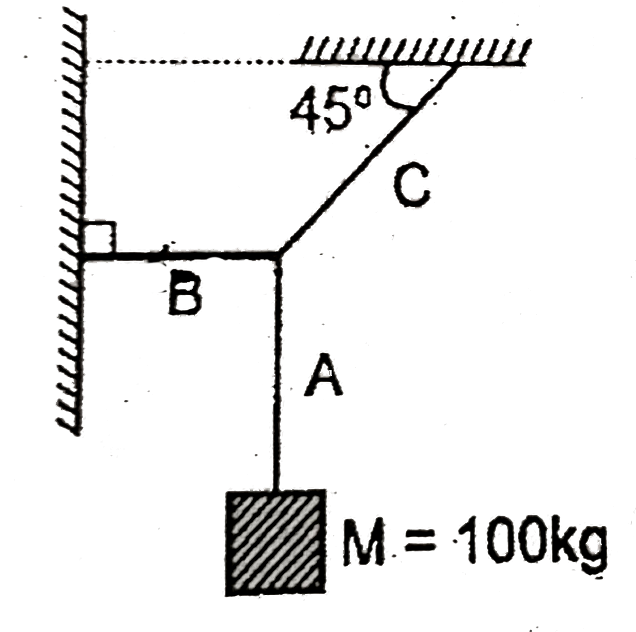 View Solution
View SolutionIf 3√b5⋅4√b3=3√bx for all b≥0, what is the value of x?
View SolutionLet C be incircle of ABC. If the tangents of lengths t1,t2andt3 are drawn inside the given triangle parallel to sidese a,bandc , respectively, the t1a+t2b+t3c is equal to 0 (b) 1 (c) 2 (d) 3
View Solution

