A circle is tangent to the lines with the equations x = 5 and y = 7. Which of the following could be the coordinates of the center of the circle ?
(3, 7)
(8, 4)
(10, 8)
(10, 14)
The correct Answer is:B
To find the coordinates of the center of a circle that is tangent to the lines x=5 and y=7, we need to determine the distances from the center of the circle to these lines. The distances must be equal because the circle is tangent to both lines.
1. Understanding the Tangent Lines:
- The line x=5 is a vertical line.
- The line y=7 is a horizontal line.
- The center of the circle will be at some point (h,k).
2. Distance from the Center to the Line x=5:
- The distance from a point (h,k) to the line x=5 is given by:
d1=|h−5|
3. Distance from the Center to the Line y=7:
- The distance from a point (h,k) to the line y=7 is given by:
d2=|k−7|
4. Setting the Distances Equal:
- Since the circle is tangent to both lines, we set the distances equal:
|h−5|=|k−7|
5. Analyzing Possible Options:
- We will check the given options to see which one satisfies the equation |h−5|=|k−7|.
6. Checking the First Option (3,7):
- For (3,7):
d1=|3−5|=2
d2=|7−7|=0
- Since d1≠d2, this option is not valid.
7. Checking the Second Option (8,4):
- For (8,4):
d1=|8−5|=3
d2=|4−7|=3
- Since d1=d2, this option is valid.
8. Checking Other Options (if any):
- If there are other options provided, repeat the distance calculations for each until you find the one that satisfies |h−5|=|k−7|.
Conclusion:
The coordinates of the center of the circle that is tangent to the lines x=5 and y=7 could be (8,4).
- The line x=5 is a vertical line.
- The line y=7 is a horizontal line.
- The center of the circle will be at some point (h,k).
- The distance from a point (h,k) to the line x=5 is given by:
d1=|h−5|
- The distance from a point (h,k) to the line y=7 is given by:
d2=|k−7|
- Since the circle is tangent to both lines, we set the distances equal:
|h−5|=|k−7|
- We will check the given options to see which one satisfies the equation |h−5|=|k−7|.
- For (3,7):
d1=|3−5|=2
d2=|7−7|=0
- Since d1≠d2, this option is not valid.
- For (8,4):
d1=|8−5|=3
d2=|4−7|=3
- Since d1=d2, this option is valid.
- If there are other options provided, repeat the distance calculations for each until you find the one that satisfies |h−5|=|k−7|.
The coordinates of the center of the circle that is tangent to the lines x=5 and y=7 could be (8,4).
Topper's Solved these Questions
Similar Questions
x2+6x+y2−8y=171
The equation of a circle in the xy-plane is shown above. What is the positive difference between the x-and y-coordinates of the center of the circle ?
View SolutionA circle touches both the coordinate axes and the line x−y=√2a,a>0, the coordinates of the centre of the circle cannot be
View Solution
Knowledge Check
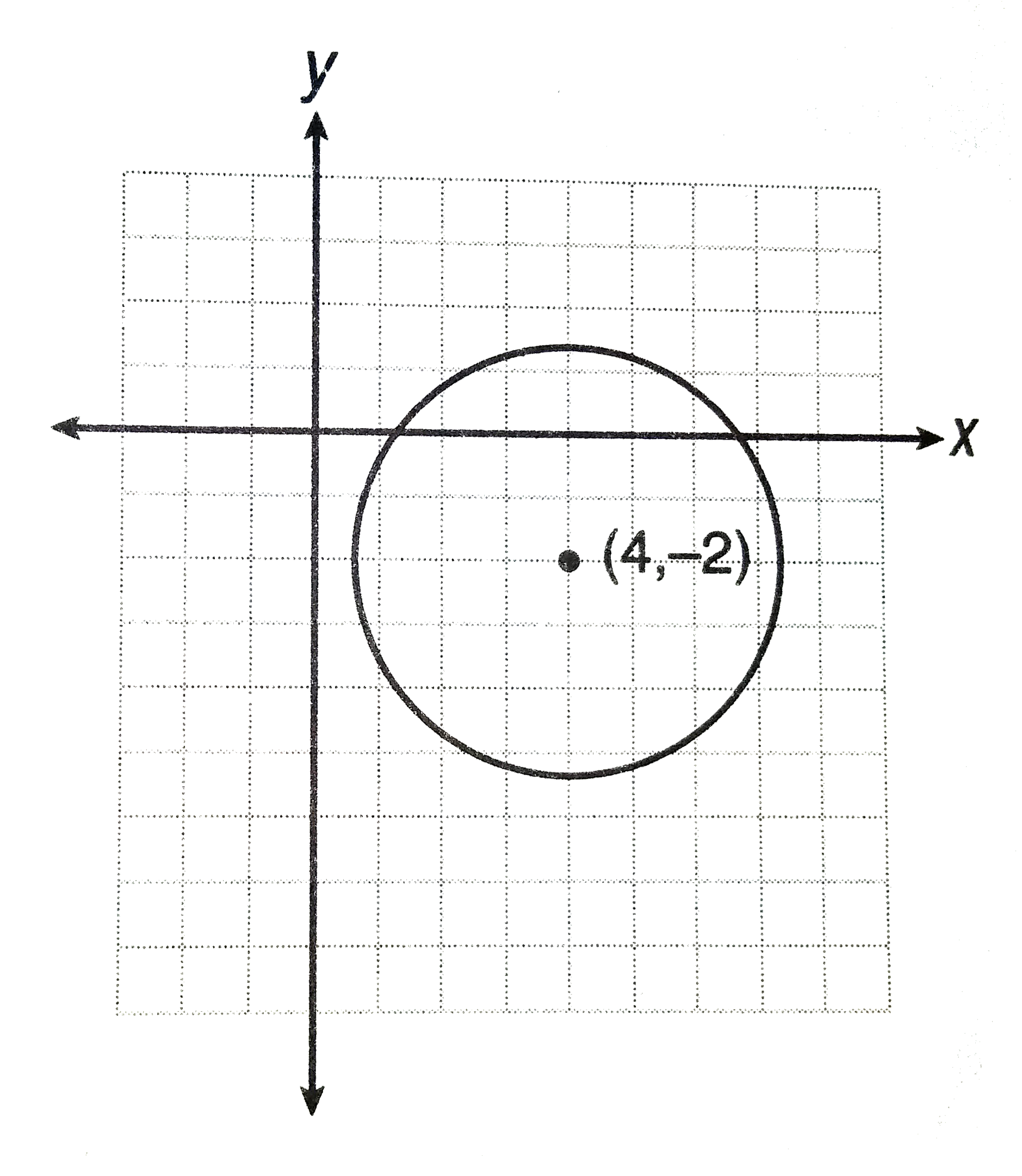
Which of the following could be an equation of the circle above?A(x−4)2+(y+2)2=17B(x+4)2+(y−2)2=17C(x−4)2+(y+2)2=13D(x+4)2+(y−2)2=13In the xy-plane, a circle with center O passes through the point (2, 0) and has a radius of 4. Which of the following could be the equation of circle O?
A(x−2)2+(y+4)2=4B(x−2)2+(y+4)2=16C(x−4)2+(y+2)2=16D(x+2)2+(y−2)2=16A circle in the xy-plane is tangent to the x-axis at -10 and the y-axis at 10. Which of the following is an equation of the circle ?
A(x-10)+(y+10)=100B(x−10)2+(y+10)2=100C(x−10)2+(y−10)2=100D(x+10)2+(y−10)2=100
A circle intersects the X axis at (1,0) and (7,0). If the radius of the circle is 5, what is the sum of the coordinates of the center?
View SolutionFind the equation of the circle which touches the coordinate axes and whose centre lies on the line x−2y=3.
View SolutionIn the figure, ΔABC is inscribed in the circle. The triangle does not contain the center of the circle O. Which one of the following could be the value of x in degree?
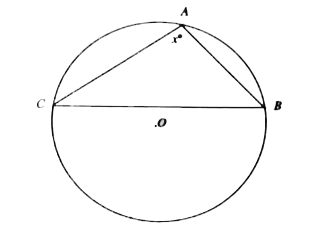
View SolutionA circle in the xy -plane has center ( 5, 7) and radius 2. Which of the following is an equation of the circle?
View SolutionA circle with center (5,-2) is tangent to the y-axis. What is the equation of the circle ?
View Solution
