A circle touches both the coordinate axes and the line $x - y = \sqrt{2} a, a > 0$ , the coordinates of the centre of the circle cannot be

A

(a, a)

B

(a, -a)

C

(-a, a)

D

(-a, -a)

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Let the radius of the circle be r. Then, coordinates of its centre can be (r, r), (-r, -r) and (r, -r).

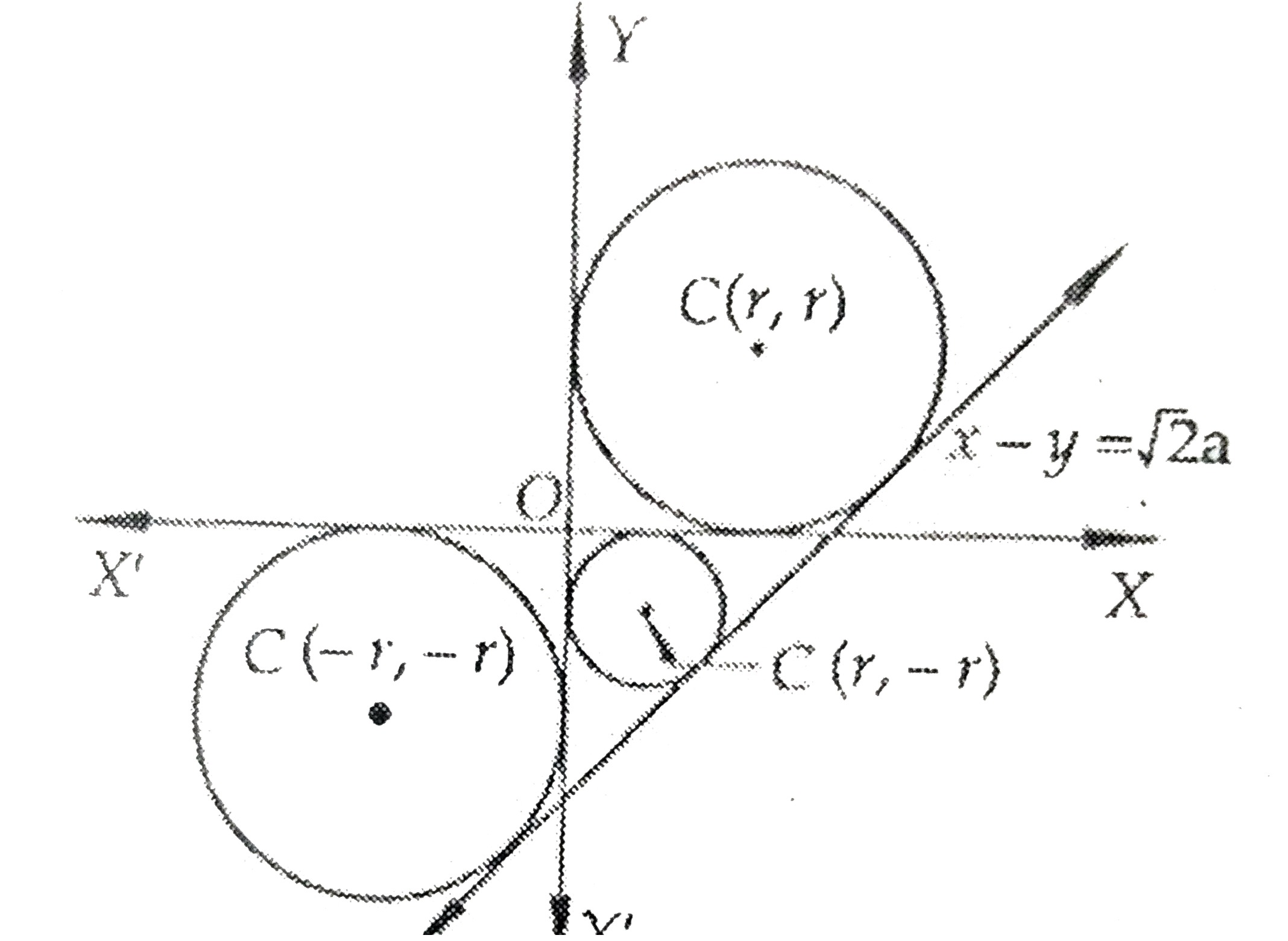
The circle touches $x - y = \sqrt{2} a$
$∴ ∣ ∣ ∣ \frac{- \sqrt{2} a}{\sqrt{2}} ∣ ∣ ∣ = r$ when centre is at (r, r) or (-r, -r)
and $∣ ∣ ∣ \frac{2 r - \sqrt{2} a}{\sqrt{2}} ∣ ∣ ∣ = r$ when centre is at (r, -r)
$\Rightarrow r = a and r = (\sqrt{2} \pm 1) a$

Updated on:21/07/2023

|

Class 12 MATHS CIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

The equation of a circle which touches both the coordinate axes and the line x = 3 is
A $4 x^{2} + 4 y^{2} - 12 x \pm 12 y + 9 = 0$
B $4 x^{2} + 4 y^{2} + 12 x \pm 12 y + 9 = 0$
C $4 x^{2} + 4 y^{2} \pm 12 x - 12 y + 9 = 0$
D $4 x^{2} + 4 y^{2} \pm 12 x + 12 y + 9 = 0$

OBJECTIVE RD SHARMA ENGLISH-CIRCLES-Section I - Solved Mcqs

Home
Ask Doubt
Profile