
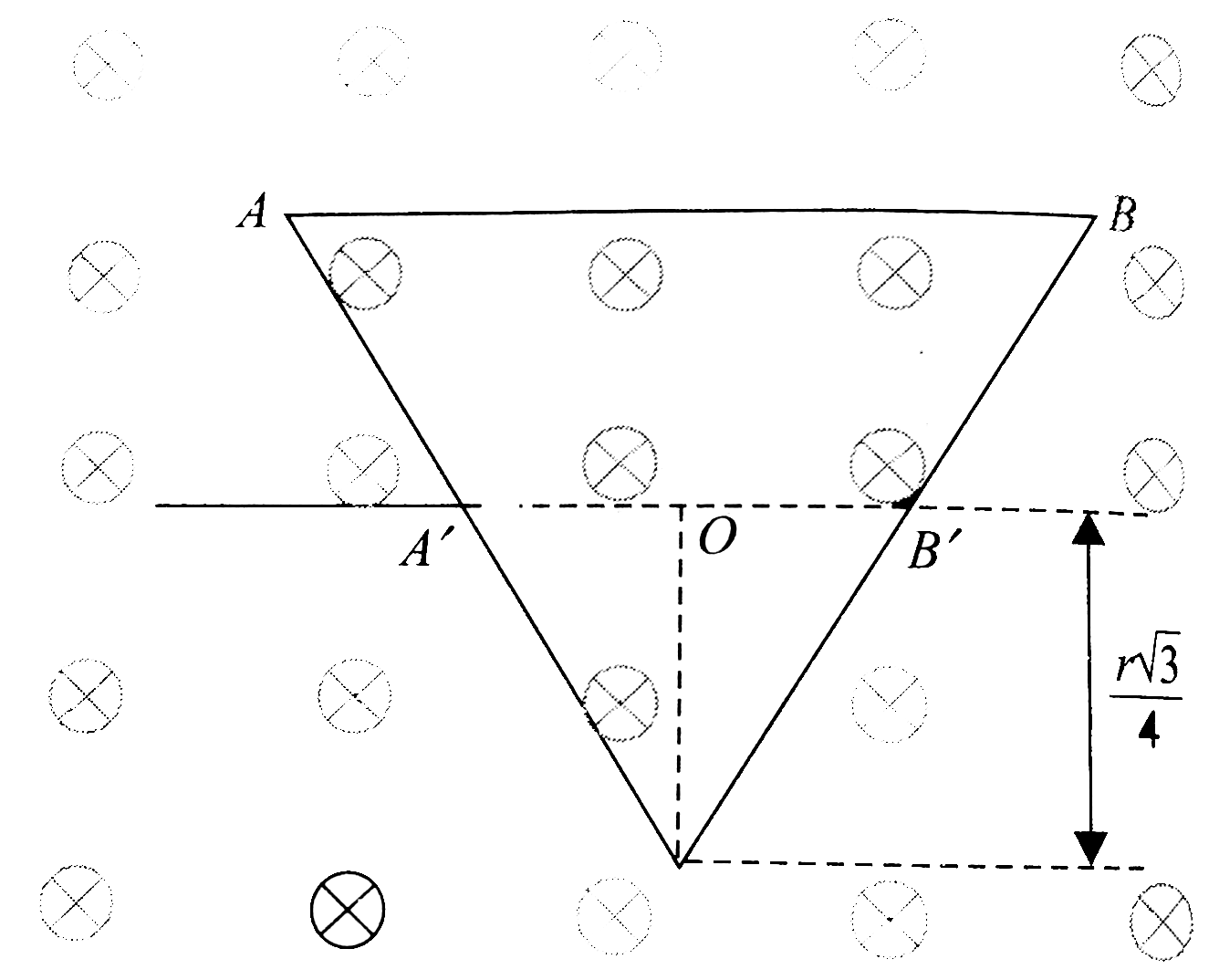
A conducting frame I the shape of an equilateral Delta (mass m, side a) carrying a current l is placed vertically an a horizontal rough surface (coefficient of friction is μ). The frame is free to rotate about y-axis only. A magnetic field exists such that →B=−B0yˆi. Then
The maximum velue of B0 so that the frame does not rotate 2μmgIa2
The maximum value of B0 so that the frame does not rotate μmgIa2
If seen from the top, the frame will hace a tendency to rotate counter clockwise.
if seen from the top, the frame will have a tendency to rotate clockwise.
The correct Answer is:A, D
dF on each of the two symmetric elements
=I×(2dy√3)B0Y×√32
⇒τ=∫√3a02⎞⎟⎠(2B0Iydy√3)×(√32a−y)
=2√3IB0∫√32a(√32a−y)ydy=IB0a38
τfriction=2{μmga∫a20xdx}=μga4
For the frame to rotate
IB0a38<μmga4
⇒B0≤2μmga4
⇒≤2μmgIa2

Topper's Solved these Questions
- TEST PAPERSBook:RESONANCEChapter:TEST PAPERSExercise:PT-01
Explore 30 Videos
- TEST PAPERSBook:RESONANCEChapter:TEST PAPERSExercise:Pt-02
Explore 30 Videos
- TEST PAPERSBook:RESONANCEChapter:TEST PAPERSExercise:PART - II PHYSICS
Explore 107 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCEChapter:SIMPLE HARMONIC MOTION Exercise:Advanced Level Problems
Explore 13 Videos
- TEST SERIESBook:RESONANCEChapter:TEST SERIESExercise:PHYSICS
Explore 127 Videos
Similar Questions
A conductor (rod) of mass m, length l carrying a current i is subjected to a magnetic field of induction B. If the coefficients of friction between the conducting rod and rail is μ, find the value of I if the rod starts sliding.
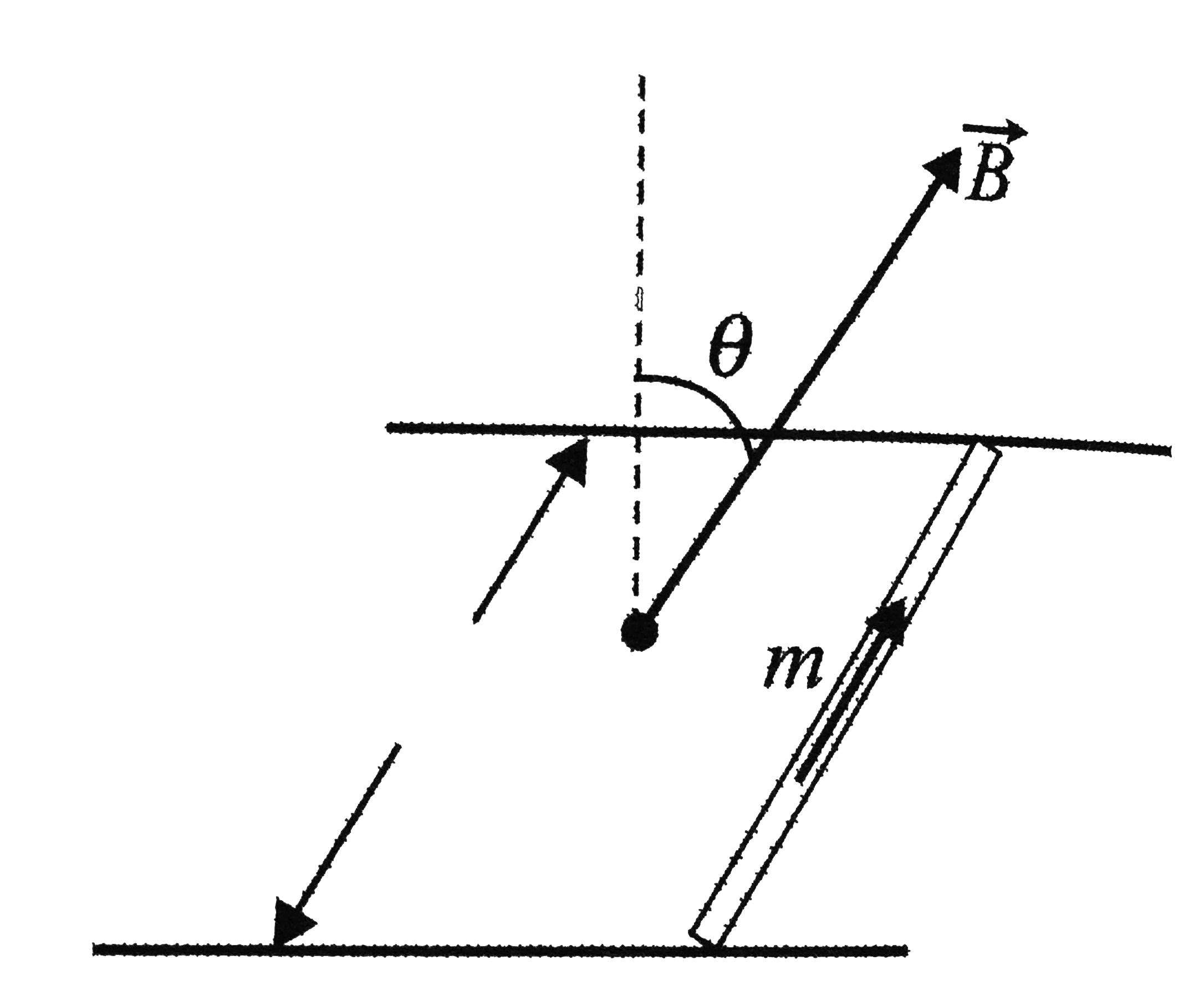
View SolutionA conductor (rod) of mass m, length l carrying a current i is subjected to a magnetic field of induction B. If the coefficients of friction between the conducting rod and rail is μ, find the value of I if the rod starts sliding.
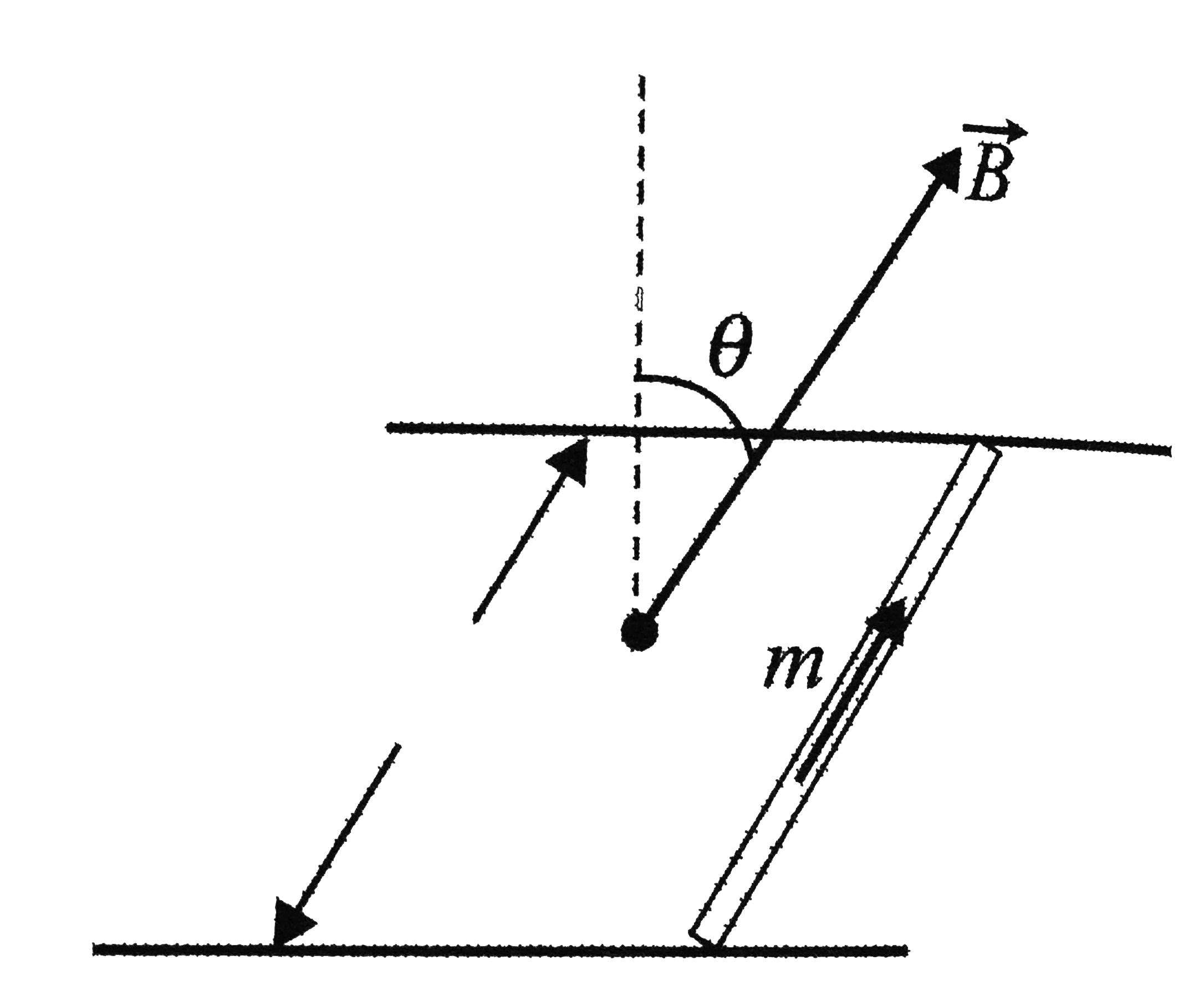
View Solution
Knowledge Check
A conducting frame in the shape of an equilateral triangle (mass m, side a) carrying a current I is placed vertically an a horizontal rough surface (coefficient of friction is μ). The frame is free to rotate about y- axis only. A magnetic field exists such that ¯¯¯B=−B0yˆi. Then
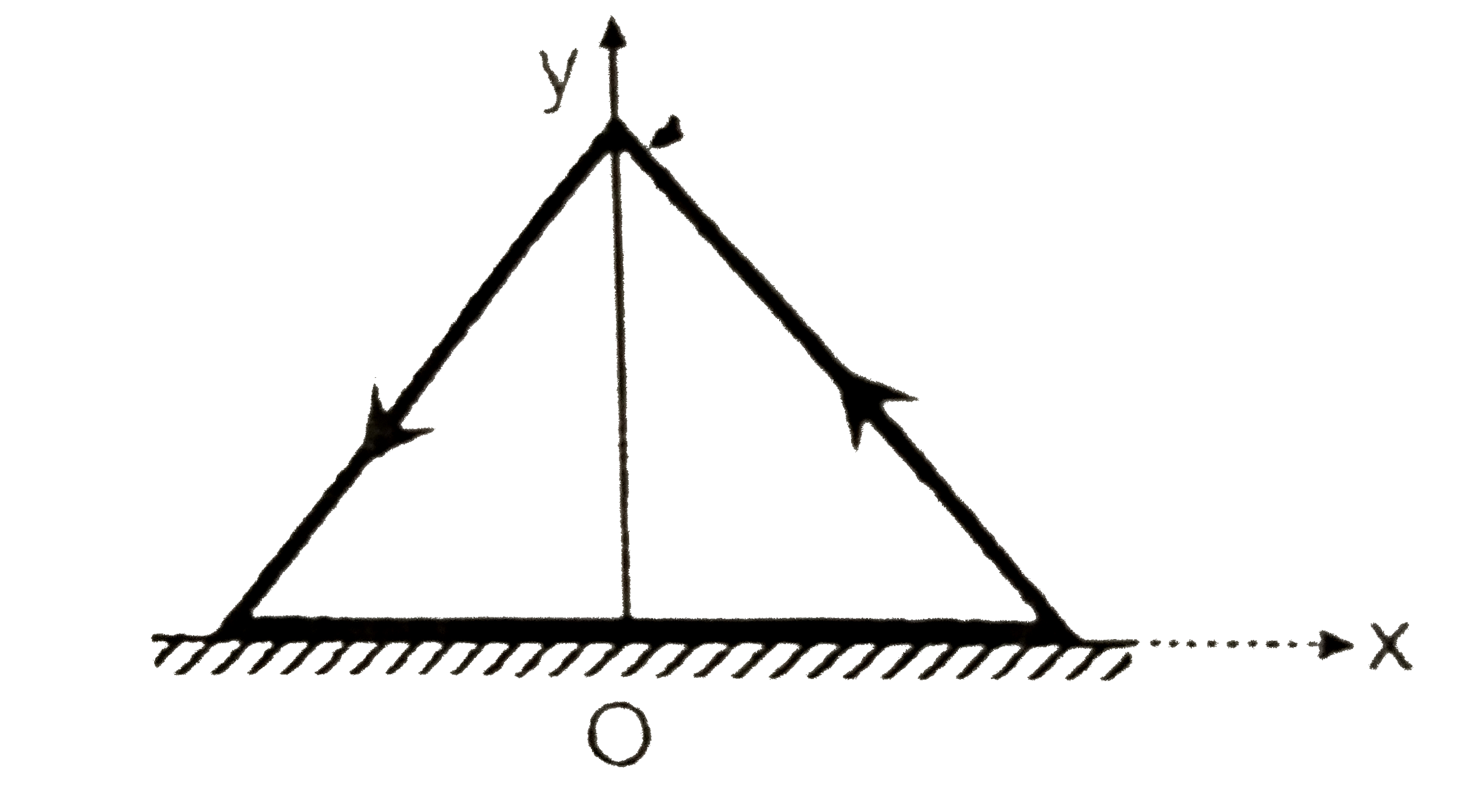 AThe maximum value of B0 so that the fram does not rotate 2μmgIa2BThe maximum value of B0 so that the frame does not rotate μmgIa2CIf seen from the top, the frame will have a tendency to rotate counter clockwise.DIf seen from the top, the frame will have a tendency to rotate clockwise.
AThe maximum value of B0 so that the fram does not rotate 2μmgIa2BThe maximum value of B0 so that the frame does not rotate μmgIa2CIf seen from the top, the frame will have a tendency to rotate counter clockwise.DIf seen from the top, the frame will have a tendency to rotate clockwise.A ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
AωoμkR2gBωog2μkRC2ωoRμkgDωoR2μkgA ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
Aω0μkR2gBω0g2μkRC2ω0RμkgDω0R2μkg
Figure shows an equilateral triangle ABC of side l carrying currents as shown, and placed in a uniform magnetic field B perpendicular to the plane of triangle. The magnitude of magnetic force on the triangle is

View SolutionFigure shows an equilateral triangle ABC of side l carrying currents as shown, and placed in a uniform magnetic field B perpendicular to the plane of triangle. The magnitude of magnetic force on the triangle is

View SolutionA current carrying conducting square frame of side I carrying current I is placed in a uniform transverse magnetic field →B as shown in the figure. Choose the incorrect statement.
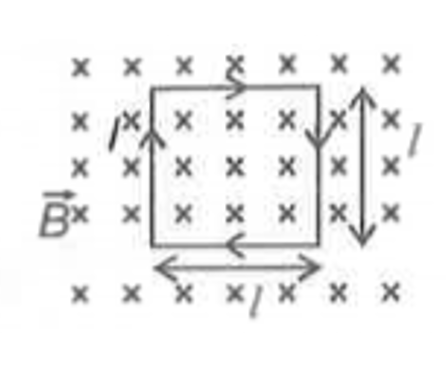
View SolutionFigure shows an equilateral triangle ABC of side l carrying currents as shown, and placed in a uniform magnetic field B perpendicular to the plane of triangle. The magnitude of magnetic force on the triangle is

View SolutionABC is an equilateral triangular frame of mass m and side r. It is at rest under the action of horizontal magnetic field B (as shown) and the gravitational field.
View Solution
