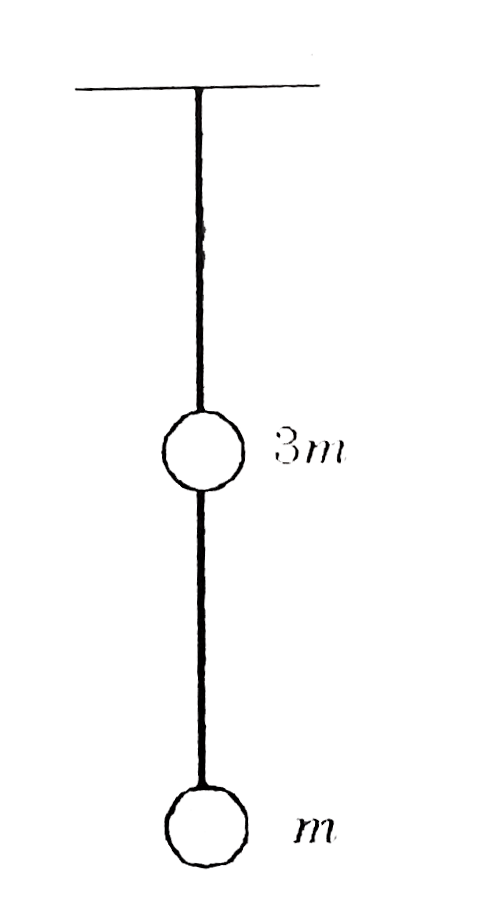
An almost inertia-less rod of length l3.5 m can rotate freely around a horizontal axis passing through its top end. At the bottom end of the roa a small ball of mass m andat the mid-point another small ball of mass 3m is attached. Find the angular frequency (in SI units) of small oscillations of the system about the equilibrium position. Gravitational acceleration is g=9.8 m//s^(2).
The correct Answer is:C, D
Similar Questions
An almost inertia-less rod of length I=3.5m can rotate freely around a horizontal axis passing through its top end. At the bottom end of the rod a small ball of mass m and at the mid-point another small ball of mass 3m is attached. Find the angular frequency ( rad / s ) of small oscillations of the system about the equilibrium position. Gravitational acceleration is g=9.8ms2.
View SolutionA rod of length l and mass m is capable of rotating freely about an axis passing through a hole at the end. The period of oscillation of this physical pendulum is
View Solution
Knowledge Check
A thin uniform rod of length l and mass m is swinging freely about a horizontal axis passing through its end. Its maximum angular speed is ω. Its centre of mass rises to a maximum height of -
A13l2ω2gB16lωgC12l2ω2gD16l2ω2gA thin uniform rod of length l and mass m is swinging freely about a horizontal axis passing through its end. Its maximum angular speed is ω. Its cenre of mass rises to a maximum height of :
A1lω6gB1l2ω2gC1l2ω26gD1l2ω23gMoment of inertia of a thin rod of mass m and length l about an axis passing through a point l/4 from one end and perpendicular to the rod is
Aml212Bml23C7ml248Dml29
A rod of length l and mass m, pivoted at one end, is held by a spring at its mid - point and a spring at far end. The spring have spring constant k. Find the frequency of small oscillations about the equilibrium position.

View SolutionA rod of length l and mass m, pivoted at one end, is held by a spring at its mid - point and a spring at far end. The spring have spring constant k. Find the frequency of small oscillations about the equilibrium position.

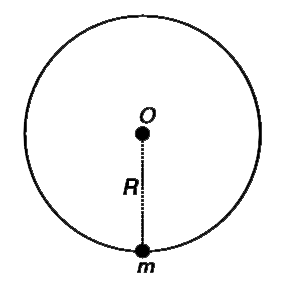
View SolutionA disc of mass M = 2m and radius R is pivoted at its centre. The disc is free to rotate in the vertical plane about its horizontal axis through its centre O. A particle of mass m is stuck on the periphery of the disc. Find the frequency of small oscillations of the system about its equilibrium position.
View SolutionA thin uniform rod of length l and mass m is swinging freely about a horizontal axis passing through its end. Its maximum angular speed is ω. Its centre of mass rises to a maximum height of -
View SolutionFind the frequency of small oscillations of thin uniform vertical rod of mass m and length l hinged at the point O.

View Solution
