A particle of mass m is attached to an end of a light rigid rod of length a. The other end of the rod is fixed, so that the rod can rotate freely in vertical plane about its fixed end. The mass m is given a horizontal velocity u at the lowest point. (a) Prove that when the radius to the mass makes an angle θ with the upward vertical the horizontal component of the acceleration of the mass (measured in direction of u) is [g(2+3cosθ)−u2/a]sinθ (b) If 4ag ltu2 lt5ag, show that there are four points at which horizontal component of acceleration is zero. locate the points.
The correct Answer is:The four points are represented by
θ=−0,πcos−1(u2−2ag3ag) and [2π−cos−1(u2−2ag3ag)]
Topper's Solved these Questions
Similar Questions
A particle of mass m is fixed to one end of a light rigid rod of length l and rotated in a vertical ciorcular path about its other end. The minimum speed of the particle at its highest point must be
View SolutionA particle is attached to an end of a rigid rod. The other end of the rod is hunged and the rod rotates always remaining horizontal. Its angular speed is increasing at contant rate. The mass of the particle is m . The force exerted by the rod on the particle is →F , then:
View Solution
Knowledge Check
A particle of mass m is fixed to one end of a light rigid rod of length l and rotated in a vertical circular path about its other end. The minimum speed of the particle at its highest point must be
AzeroB√glC√1.5glD√2glA particle of mass m is fixed to me one end of a light right rod of length l and rotated in a vertical circular path about its other end. The minmum speed of the particle at its highest point must be
AzeroB√glC√1.5glD√2glA particle is attached to an end of a rigid rod. The other end of the rod is hunged and the rod rotates always remaining horizontal. Its angular speed is increasing at contant rate. The mass of the particle is m . The force exerted by the rod on the particle is →F , then:
AF≥mgBF is constantCThe angle between →F and horizontal plane decreases.DThe angle between →F and the rod decreases.
A particle of mass of mass m is attached to a light rod of lenght l other end of the rod is hinged to a wall . Minimumm velocity (Vo) that should be given to a particle in lowest positiion so that it completes a vertical circle
View SolutionA thin rod MN free to rotation in the vertical plane about the fixed end N is held horizontal when the end M is released the speed of this end when the rod makes an angle α with the horizontal will be proportional to : (see figure)
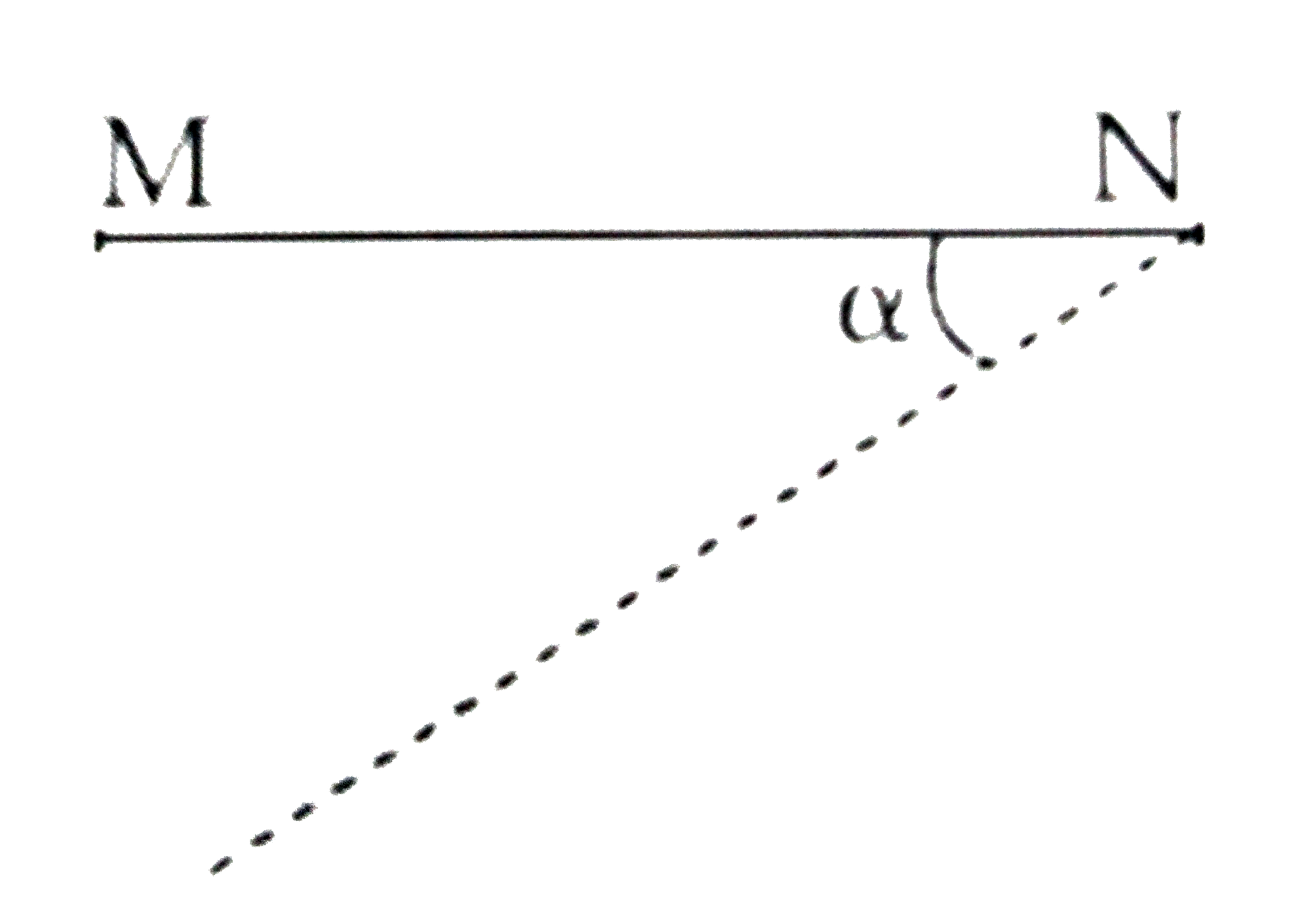
View SolutionA thin rod MN free to rotation in the vertical plane about the fixed end N is held horizontal when the end M is released the speed of this end when the rod makes an angle α with the horizontal will be proportional to : (see figure)

View SolutionTwo particles of mass m each are attached to a light rod of length d, one at its centre and the other at a free end, The rod is fixed at the other end is rotated in a plane at an angular speed ω. Calculate the angular momentum of the particle at the end with respect to the particle at the centre
View SolutionTwo particles of mass m each are attached to a light rod of length d, one at its centre and the other at a free end, The rod is fixed at the other end and is rotated in a plane at an angular speed ω. Calculate the angular momentum of the particle at the end with respect to the particle at the centre
View Solution
