A particle of mass m is fixed to one end of a light rigid rod of length l and rotated in a vertical ciorcular path about its other end. The minimum speed of the particle at its highest point must be
zero
√gl
√1.5gl
√2gl
The correct Answer is:A
To solve the problem of finding the minimum speed of a particle at its highest point when it is rotated in a vertical circular path, we can follow these steps:
1. Understanding the Setup:
- We have a particle of mass m attached to one end of a rigid rod of length l.
- The other end of the rod is fixed, and the particle rotates in a vertical circular path about this fixed point.
2. Identify the Highest Point:
- The highest point in the circular path is where we need to find the minimum speed of the particle. Let's denote this point as A.
3. Forces at the Highest Point:
- At the highest point, the forces acting on the particle are:
- The gravitational force mg acting downwards.
- The tension T in the rod acting downwards as well (since the rod is rigid and the particle is at the top).
4. Centripetal Force Requirement:
- For the particle to maintain circular motion at the highest point, the centripetal force required is provided by the gravitational force and the tension in the rod. Thus, we can write:
mv2l=mg+T
- Here, v is the speed of the particle at the highest point, and l is the length of the rod (which is also the radius r of the circular path).
5. Minimum Speed Condition:
- The minimum speed occurs when the tension T is zero (the particle is just about to lose contact with the rod). Therefore, we set T=0:
mv2l=mg
6. Solving for Minimum Speed:
- Rearranging the equation, we have:
v2=gl
- Taking the square root gives us:
v=√gl
Final Answer:
The minimum speed of the particle at its highest point must be:
v=√gl
- We have a particle of mass m attached to one end of a rigid rod of length l.
- The other end of the rod is fixed, and the particle rotates in a vertical circular path about this fixed point.
- The highest point in the circular path is where we need to find the minimum speed of the particle. Let's denote this point as A.
- At the highest point, the forces acting on the particle are:
- The gravitational force mg acting downwards.
- The tension T in the rod acting downwards as well (since the rod is rigid and the particle is at the top).
- For the particle to maintain circular motion at the highest point, the centripetal force required is provided by the gravitational force and the tension in the rod. Thus, we can write:
mv2l=mg+T
- Here, v is the speed of the particle at the highest point, and l is the length of the rod (which is also the radius r of the circular path).
- The minimum speed occurs when the tension T is zero (the particle is just about to lose contact with the rod). Therefore, we set T=0:
mv2l=mg
- Rearranging the equation, we have:
v2=gl
- Taking the square root gives us:
v=√gl
The minimum speed of the particle at its highest point must be:
v=√gl
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Level 1 Subjective
13 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Level 2 Single Correct
18 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Assertion And Reason
28 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
A stone is tied to one end of a light inexensible string of length l and made to roate on a vertical circle keeping other end of the spring at the centre. If speed of stone at the highest point is v(v>√gl) then its speed at the lowest point is
View SolutionA particles of mass m is fixed to one end of a light spring of force constant k and unstreatched length l. the system is rotated about the other end of the spring with an angular velocity ω in gravity free space. The increase in length of the spring is
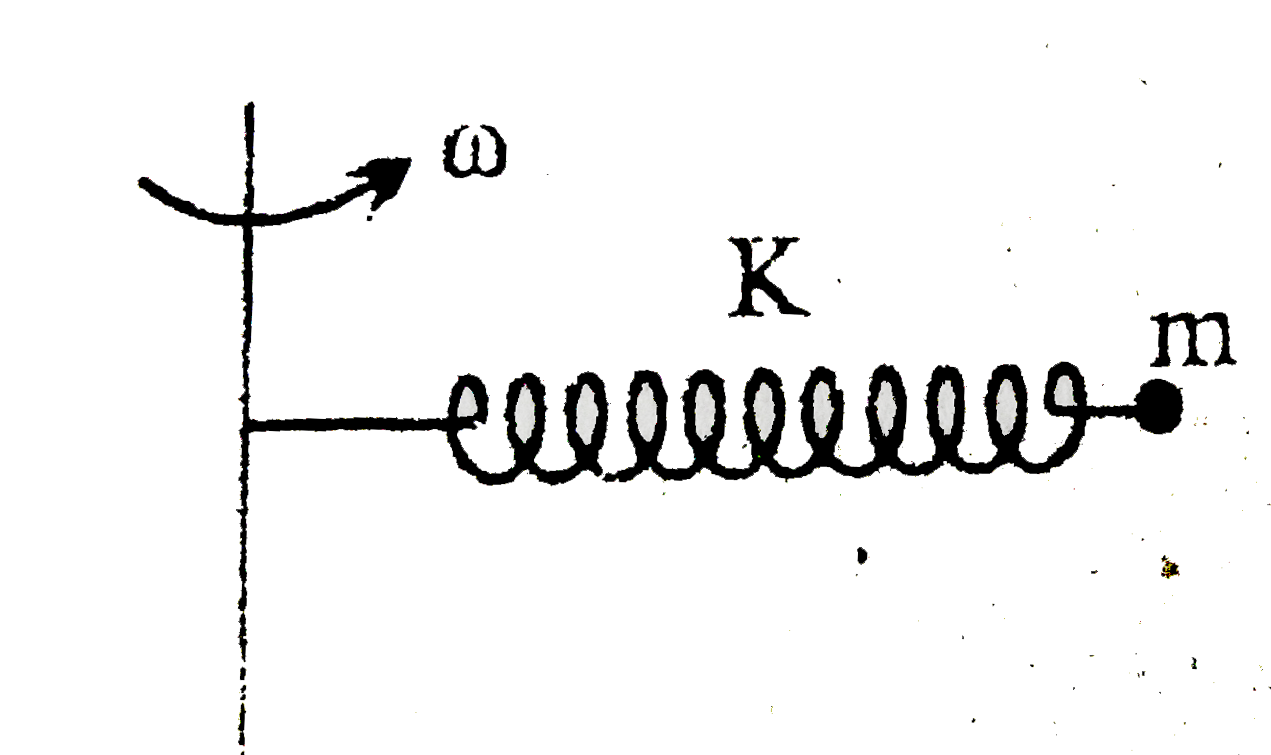
View SolutionA particle is fastened at the end of a string and whirled in a vertical circle with the other end of the string being fixed. The motion of the particle is
View SolutionA particle is rotated in a vertical circle by connecting it to a string of length l and keeping the other end of the string fixed. The minimum speed of the particle when the string is horizontal for which the particle will complete the circle is
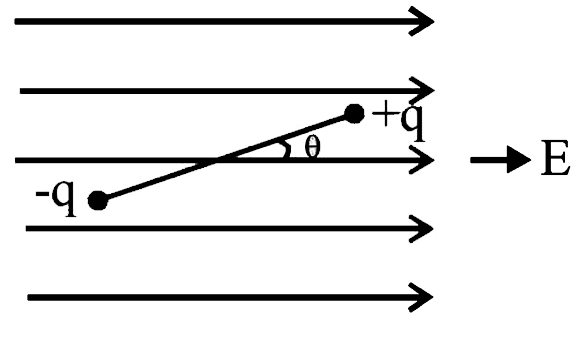
View SolutionA point particle of mass M is attached to one end of a massless rigid non-conducting rod of length L. Another point particle of the same mass is attached to the other end of the rod. The two particles carry charges +q and −q respectively. This arrangement is held in a region of a uniform electric field E such that the rod makes a small angle θ (say of about 5 degree) with the field direction, fig. Find an expression for the minimum time needed for the rod to become parrallel to the field after it is set free.
View SolutionOne end of string of length 1.5 m is tied to a stone of mass 0.4 kg and the other end to a small pivot on a smooth vertical board. What is the minimum speed of the stone required at its lowermost.point so that the string does not slack at any point in its motion along the vertical circle ?
View SolutionA particle of mass m is fixed to one end of a massless spring of spring constant k and natural length l0. The system is rotated about the other end of the spring with an angular velocity ω ub gravity-free space. The final length of spring is
View SolutionA particle of mass 'm' is attached to one end of a string of length 'l' while the other end is fixed to a point 'h' above the horizontal table, the particle is made to revolve in a circle on the table, so as to make P revolution per sec. The maximum value of P if the particle is to be contact with the table is
View SolutionA light rigid rod of length l is hinged at one end and it is free to rotate in a vertical plane. A particle of mass m is attached to another end of the rod. The particle is released from rest at its highest point. Select the correct statement out of the following
View SolutionA particle is rotated in a verticle circle by connecting it to the end of massless rod of length l and keeping the other end of rod fixed. The minimum speed of the particle when the rod is horizontal for which the particle will complete the circle is
View Solution
