Pendulum of a clock consists of very thin sticks of iron and an alloy. At room temperature the iron sticks 1 and 2 have length each. Length of each of the two alloy sticks 4 and 5 is 0 and the length of iron stick 3 (measured up to the centre of the iron bob) is . Thickness of connecting strips are negligible and mass of everything except the bob is negligible. The pendulum oscillates about the horizontal axis shown in the figure. It is desired that the time period of the pendulum should not change even if temperature of the room changes. Find the coefficient of linear expansion () of the alloy if the coefficient of linear expansion for iron is .
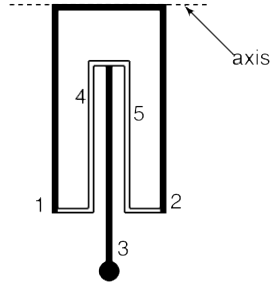
The correct Answer is:
Topper's Solved these Questions
Similar Questions
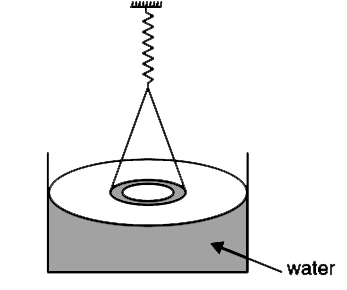
A circular ring has inner and outer radii equal to 10mmand30mm respectively Mass of the ring is m=0.7 g. It gently pulled out vertically from a water surface by a sensitive spring. When the spring is stretched 3.4cm from its equilibrium position the ring is on verge of being pulled out from the water surface. If spring constant is k=0.7Nm–1 find the surface tension of water.
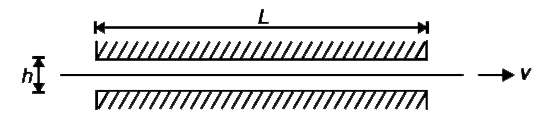
View SolutionDuring a painting process, a thin, flat tape of width b (dimension perpendicular to the plane of the figure) is pulled through a paint filled channel of length L. The density and viscosity of the paint liquid is ρ and η respectively. The tape is pulled at a constant speed v and width of the channel is h. Find the minimum force needed to pull the tape.
View SolutionA stick has a length of 12.132cm and an other stick has a length of 12.4cm then the total length of the stick is...........
View Solutionकाँच , लकड़ी या लोहे पर पारा नहीं चिपकता है, क्योंकि :
View SolutionA stick has a length of 12.132 cm and another stick has a length of 12.4 cm .
(a) If the two sticks are placed end to end , what is their total length ?
(b) If the two sticks and placed sides by side, what is the difference in their lengths ?
View SolutionWhere does the iron filings stick the most in the magnet?
View SolutionThe poles of magnet where Iron is able to stick are
View SolutionA stick has a length of 12.132 cm and another stick has a length of 12.4 cm.
If the two sticks are placed side by side, what is the difference in their lengths ?
View SolutionA stick has a length of l12.132 cm and another stick has a length of 12.4 cm.
(a) If the two sticks are placed end to end, what is their total length ?
(b) If the two sticks are placed side by side, what is the difference in their lengths ?
View SolutionIron fillings stick at the _____________ of bar magnet.
View SolutionA stick has a length of 12.132 cm and another stick has a length of 12.4 cm . (a) If the two sticks are placed end to end, what is their total length? (b) If the two sticks are placed side by side, what is the difference in their lengths?
View SolutionA stick has a length of 12.132cm and another stick has a length of 12.4cm.
If the two sticks are placed end to end, what is their total length?
If the two sticks are placed side by side , what is the difference in their lengths?
View Solutionविद्युत क्रेन से लोहे से बनी वस्तुएँ क्यों चिपक जाती हैं?
View SolutionA triangle is made up of 2 red sticks and 1 blue sticks
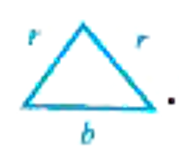
length of a red stick is given by r and that of a blue stick is given by b. Using this information, write an expression for the total length of sticks in the pattern given below:
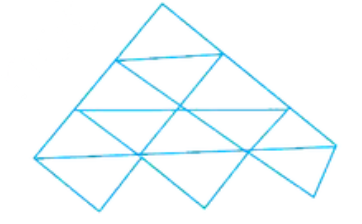
View SolutionA bar magnet is dipped in a leap of small iron filings. It is observed that a cluster of iron filings stick to the ends A and B while there is practically no iron filing stick to its central region. Answer the following questions The central region are called
View Solution
