A block is kept in a room which is at 20∘C. To raise the temperature of the block, heat is given to it at a constant rate of 600 watt (using an electric heater). The temperature of the block rises with time as shown in the graph. The slope of the graph at time t=0 is 3∘Cs−1. Once the temperature rises to 60∘C, the heater is switched off and another heater is switched on to maintain the temperature of the block at 60∘C. This new heater supplies heat at a constant rate of 100 watt. Assume that heat capacity of the block remains constant for the range of temperature involved.
(a) Explain why the slope of the given graph is decreasing with time.
(b) Calculate the heat capacity of the block.
(c) If the 100 W heater is also switched off, what will be initial rate of cooling of the block?
(d) Assuming that rate of heat loss by the block to the surrounding is proportional to difference in its temperature with surrounding, calculate the heat radiated per second by the block when it was at 30∘C.
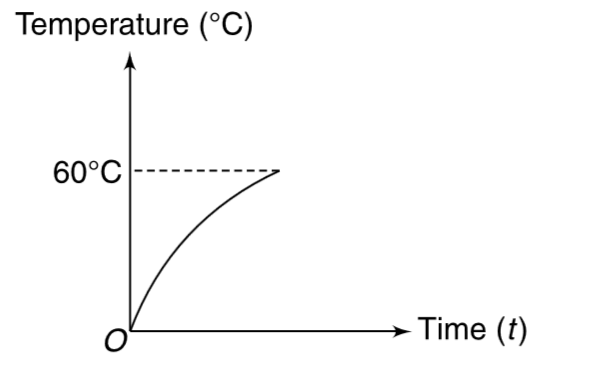
The correct Answer is:(b) 200J∘C−1 (c) 0.5∘C−1 (d) 25W
Step by step video, text & image solution for A block is kept in a room which is at 20^(@)C. To raise the temperature of the block, heat is given to it at a constant rate of 600 watt (using an electric heater). The temperature of the block rises with time as shown in the graph. The slope of the graph at time t = 0 is 3^(@)C s^(-1). Once the temperature rises to 60^(@)C, the heater is switched off and another heater is switched on to maintain the temperature of the block at 60^(@)C. This new heater supplies heat at a constant rate of 100 watt. Assume that heat capacity of the block remains constant for the range of temperature involved. (a) Explain why the slope of the given graph is decreasing with time. (b) Calculate the heat capacity of the block. (c) If the 100 W heater is also switched off, what will be initial rate of cooling of the block? (d) Assuming that rate of heat loss by the block to the surrounding is proportional to difference in its temperature with surrounding, calculate the heat radiated per second by the block when it was at 30^(@)C. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Two lead balls of mass m and 2 m are placed at a separation d. A third ball of mass m is placed at an unknown location on the line joining the first two balls such that the net gravitational force experienced by the first ball is 6Gm2d2 . What is the location of the third ball?
View SolutionA car manufacturer usually tells a optimum speed (V0) at which the car should be driven to get maximum mileage. In order to find the optimum speed for a new model, an engineer of the car company experimented a lot and finally plotted a graph between the extreme time t (defined as number of hours a tank full of petrol lasts) vs the constant speed V at which car was run.
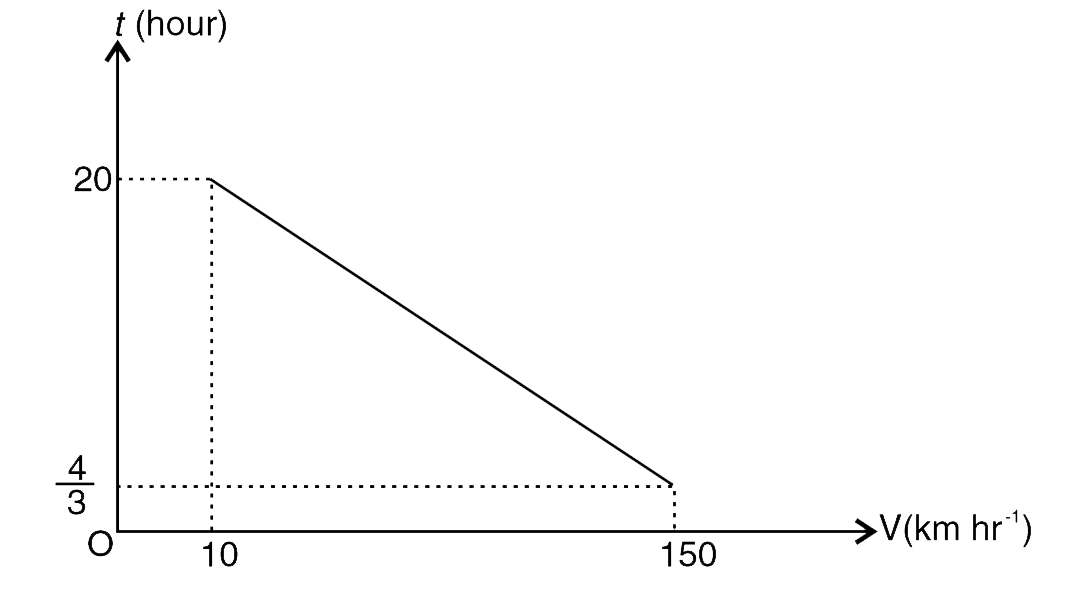
(a) Calculate the optimum speed V0 for this new model.
(b) If the fuel tank capacity of this car is 50 litre, what maximum mileage can be obtained from this car?
View SolutionA metal block of heat capacity 90J/.∘C placed in a room at 25∘C is heated electrically. The heater is switched off when the temperature reaches 35∘C. The temperature of the block rises at the rate of 2∘C/s just after the heater is switched on and falls at the rate of 0.2∘C/s just after the heater is switched off. Assume Newton's law of cooling to hold (a) Find the power of the heater. (b) Find the power radiated by the block just after the heater is switched off. (c ) Find the power radiated by the block when the temperature of the block is 30∘C. (d) Assuming that the power radiated at 30∘C respresents the average value in the heating process, find the time for which the heater was kept on.
View SolutionA block of wood is floating in water at 0^@ C . The temperature of water is slowly raised from 0^@ C to 10^@ C . How will the precentage of volume of block above water level change with rise in temperature?
View SolutionThe temperature of a room heated by heater is 20∘C when outside temperature is −20∘C and it is 10∘C when the outside temperature is −40∘C. The temperature of the heater is
View SolutionA metal block is placed in a room which is at 10∘C for long time. Now it is heated by an electric heater of power 500W till its temperature becomes 50∘C Its initial rate of rise of temperature is 2.5∘C/sec The heater is switched off and now a heater of 100W is required to maintain the temperature of the block at 50∘C The heat radiated per second when the block was 30∘C is given as α watt. Find the value of (α10) (Assume Newton's law of cooling to be valid .
View SolutionA metal block of heat capacity 90J/.∘C placed in a room at 25∘C is heated electrically. The heater is switched off when the temperature reaches 35∘C. The temperature of the block rises at the rate of 2∘C/s just after the heater is switched on and falls at the rate of 0.2∘C/s just after the heater is switched off. Assume Newton's law of cooling to hold (a) Find the power of the heater. (b) Find the power radiated by the block just after the heater is switched off. (c ) Find the power radiated by the block when the temperature of the block is 30∘C. (d) Assuming that the power radiated at 30∘C respresents the average value in the heating process, find the time for which the heater was kept on.
View SolutionA metal ball of mass 1.0 kg is kept in a room at 15^(@)C . It is heated using a heater. The heater supplies heat to the ball at a constant rate of 24 W. The temperature of the ball rises as shown in the graph. Assume that the rate of heat loss from the surface of the ball to the surrounding is proportional to the temperature difference between the ball and the surrounding. Calculate the rate of heat loss from the ball when it was at temperature of 20^(@)C .
View SolutionA metal block is placed in a room which is at 10∘C. It is heated by an electric heater of power 500W till its temperature becomes 50∘C. Its initial rate of rise of temperature is 2.5∘C/sec. The heater is switched off and now a heater of 100W is required to maintain the temperature of the block at 50∘C. (Assume Newtons Law of cooling to be valid)
(i) What is the heat capacity of the block ?
(ii) What is the rate of cooling of block at 50∘C if the 100W heater is also switched off ?
(iii) What is the heat radiated per second when the block was 30∘C ?
View SolutionA metal block is placed in a room which is at 10^(@)C for long time. Now it is heated by an electric heater of power 500 W till its temperature becomes 50^(@)C . Its initial rate of rise of temperature is 2.5^(@)C//sec . The heater is switched off and now a heater of 100W is required to maintain the temperature of the block at 50^(@)C . (Assume Newtons Law of cooling to be valid) What is the heat capacity of the block?
View SolutionA metal block is placed in a room which is at 10^(@)C for long time. Now it is heated by an electric heater of power 500 W till its temperature becomes 50^(@)C . Its initial rate of rise of temperature is 2.5^(@)C//sec . The heater is switched off and now a heater of 100W is required to maintain the temperature of the block at 50^(@)C . (Assume Newtons Law of cooling to be valid) What is the rate of cooling of block at 50^(@)C if the 100W heater is also switched off?
View SolutionA metal block with a heater in it is placed in a room at temperature 293 K. When the heater is switched on it is observed that the temperature of the block rises at the rate of 2∘C/s and when its temperature rises to 30∘C, it is switched off. Just after when heater is switched off, it is observed that the block cools at 0.2∘C/s. If Newton's law of cooling is assumed to be valid, find the power of the heater. Also find the thermal power radiated by the block when it was at 30∘C and at 25∘C. Given that the heat capacityof the block is 80J/∘C.
View SolutionA cubical tank of water of volume 1m3 is kept at a constant temperature of 65∘C by 1 KW heater. At time t=0, the heater is switched off. Find the time taken by the tank to cool down to 50∘C, given the temperature of the room is steady at 15∘C. Density of water =103kgm−3 and specific heat of water =1.0calg−1^(∘)C−1. (Do not assume average temperature during cooling). Take 1KW=240cals−1.
View SolutionA block of wood is floating in water at 0∘C. The temperature of water is slowly raised from 0∘C to 10∘C. How will the precentage of volume of block above water level change with rise in temperature?
View SolutionA metal block is placed in a room which is at 10∘C for long time. Now it is heated by an electric heater of power 500 W till its temperature becomes 50∘C. Its initial rate of rise of temperature is 2.5∘C/sec. The heater is switched off and now a heater of 100W is required to maintain the temperature of the block at 50∘C . (Assume Newtons Law of cooling to be valid)
What is the heat capacity of the block?
View Solution
