A copper slab is 2 mm thick. It is protected by a 2 mm layer of stainless steel on both sides. The temperature on one side of this composite slab is 400∘C and 200∘C on the other side. Value of thermal conductivities are-
kcu=400Wm−1K−1 and ks=16Wm−1K−1
(a) Just by knowing that thermal conductivity of steel is much less than copper, find (approximately) the temperature of the copper slab.
(b) Plot the variation of temperature across the thickness of the composite wall.
The correct Answer is:(a) Temperature drop will take palace almost entirely in steel. The copper plate will have almost uniform temperature pf 400+2002=300∘
(##IJAPHYV02C05E05032_A01##)
Step by step video, text & image solution for A copper slab is 2 mm thick. It is protected by a 2 mm layer of stainless steel on both sides. The temperature on one side of this composite slab is 400^(@)C and 200^(@)C on the other side. Value of thermal conductivities are- k_(cu)=400 Wm^(-1)K^(-1) and k_(s)=16 Wm^(-1)K^(-1) (a) Just by knowing that thermal conductivity of steel is much less than copper, find (approximately) the temperature of the copper slab. (b) Plot the variation of temperature across the thickness of the composite wall. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
Calculate the quantity of heat conducted through 2m2 of a brick wall 12cm thick in 1hour if the temperature on one side is 8∘C and on the other side is 28∘C. (Thermal conductivity of brick =0.13Wm−1K−1)
View SolutionTwo identical metal wires of thermal conductivities K1 and K2 respectively are connected in series. The effective thermal conductivity of the combination is :
View Solution
Knowledge Check
- Question 1 - Select One
If the temperature difference on the two sides of a wall increases from 100∘C to 200∘C, its thermal conductivity
Aremains unchangedBis doubledCis halvedDbecomes four times - Question 2 - Select One
The coefficient of thermal conductivity of copper is nine times of steel in the composite cylindrical bar shown in the figure. What is the temperature at the junction of copper & steel?
 A61∘CB62∘CC65∘CD66∘C
A61∘CB62∘CC65∘CD66∘C - Question 3 - Select One
Two walls of thickness d and d and thermal conductivities k and k are in contact. In the steady state, if the temperature at the outer T1 and T2 , the temperature at the common wall is
Ak1T1d2+k2T2d1k1d2+k2d1Bk1T1+k2d2d1+d2C(k1d1+k2d2T1+T2)T1T2Dk1d1T1+k2d2T2k1d1+k2d2
A composite slab is prepared by pasting two plates of thickness L1 and L2 and thermal conductivites K1 and K2 . The slab have equal cross-sectional area. Find the equivalent conductivity of the composite slab.
View Solution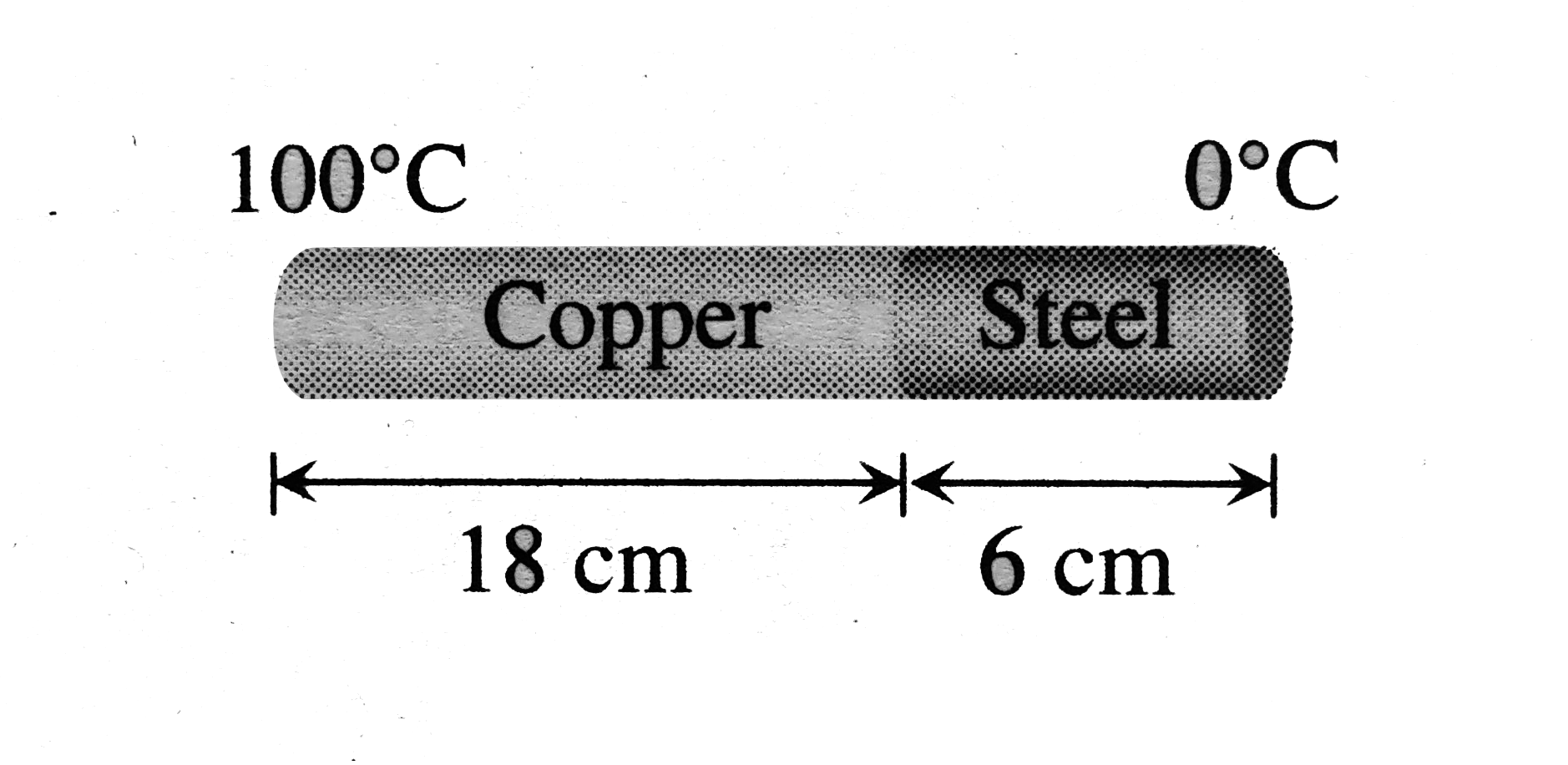
The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?View Solution
The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?View SolutionA slab consists of two layers of different materials of the same thickness and having thermal conductivities K1 and K2. The equivalent thermal conductivity of the slab is
View SolutionTwo rods of the same length and diameter, having thermal conductivities K1 and K2, are joined in parallel. The equivalent thermal conductivity to the combinationk is
View Solution

