The centre of mass of an inhomogeneous sphere is at a distance of 0.3 R from its geometrical centre. R is the radius of the sphere. Find the maximum inclination (θ) of an incline plane on which this sphere can be placed in equilibrium. Assume that friction is large enough to prevent slipping.
The correct Answer is:sin−1(0.3)
Step by step video, text & image solution for The centre of mass of an inhomogeneous sphere is at a distance of 0.3 R from its geometrical centre. R is the radius of the sphere. Find the maximum inclination (theta) of an incline plane on which this sphere can be placed in equilibrium. Assume that friction is large enough to prevent slipping. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
The centre of neutral conducting sphere of radius "R" is at distance 'd' from a point charge "Q" .The potential of the sphere is
View SolutionIf a sphere of radius 10 cm is intersected by a plane at a distance 8 cm from its centre, what is the radius of the curve of intersection of the plane and the sphere?
View Solution
Knowledge Check
- Question 1 - Select One
The potential at a distance R/2 from the centre of a conducting sphere of radius R will be
A0BQ8πε0RCQ4πε0RDQ2πε0R - Question 2 - Select One
The radius of gyration of a solid sphere of radius r about a certain axis is r. The distance of this axis from the centre of the sphere is
ArB0.5 rC√0.6rD√0.4r - Question 3 - Select One
A sphere is rolling down a plane of inclination θ to the horizontal. The acceleration of its centre down the plane is
AgsinθBless than gsinθCgreater than gsinθDzero
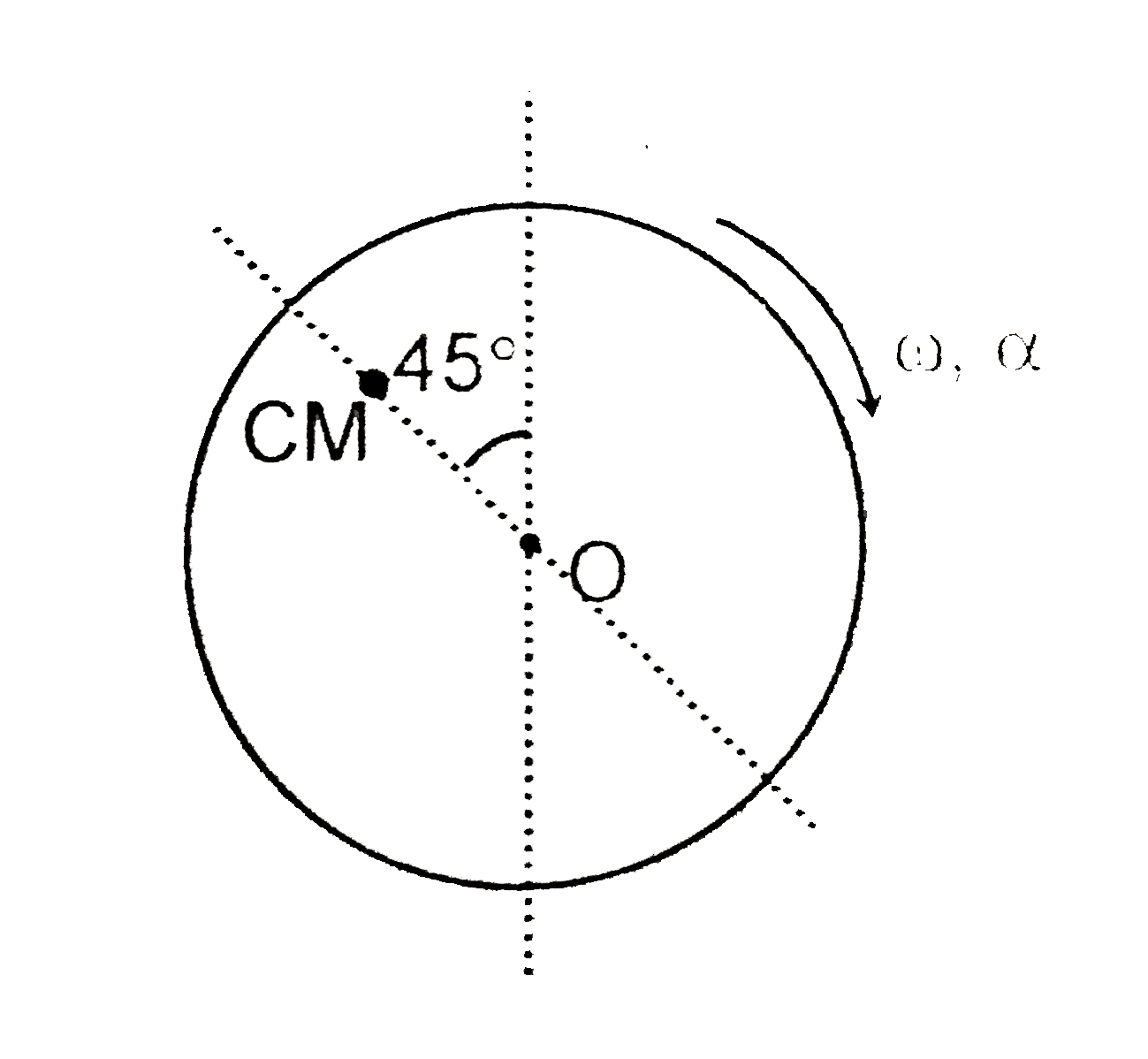
A solid sphere of mass m and radius R is rolling without slipping on a rough horizontal surface with angular acceleration α. Centre of mass of sphere lies at a distance R√2 from the centre of sphere. Find the normal force applied by sphere on the surface at an instant when line joining centre of mass of sphere and centre of sphere makes an angle 45∘ with the vertical and angular velocity of sphere at this instant is ω.View SolutionA solid sphere of mass m rolls without slipping on an inclined plane of inclination θ. The linear acceleration of the sphere is
View SolutionCharge density of a sphere of radius R is ρ=ρ0r where r is distance from centre of sphere.Total charge of sphere will be
 View Solution
View SolutionA sphere of radius R has a charge density σ. The electric intensity at a point at a distance r from its centre is
View SolutionA conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
View Solution

