A thin uniform steel chain is 10 meters long with a mass density of 2 kilograms per meter. One end of the chain is attached to a horizontal axis having radius that is small compared to the legnth of the chain. If the chain initially hangs vertically, the work required to slowly wind it up on to the axle is closest to
100 J
200 J
1000 J
2000 J
The correct Answer is:C
To solve the problem of calculating the work required to wind up a thin uniform steel chain of length 10 meters and mass density of 2 kg/m, we can follow these steps:
1. Determine the total mass of the chain:
The mass density (λ) of the chain is given as 2 kg/m. The total length (L) of the chain is 10 m. Therefore, the total mass (M) of the chain can be calculated as:
M=λ×L=2kg/m×10m=20kg
2. Identify the small segment of the chain:
Consider a small segment of the chain of length dy at a height y from the bottom of the chain. The mass of this small segment (dm) can be expressed as:
dm=λ×dy=2kg/m×dy
3. Calculate the weight of the small segment:
The weight (dW) of this small segment is given by:
dW=dm×g=(2dy)×g
where g is the acceleration due to gravity (approximately 9.81m/s2).
4. Determine the work done to lift this small segment:
The work done to lift this small segment from height y to the horizontal axis (which is at height 0) is:
dW=(2dy)×g×y
5. Integrate to find the total work done:
To find the total work done to lift the entire chain, we integrate dW from y=0 to y=10:
W=∫100(2g⋅y)dy
W=2g∫100ydy
W=2g(y22)100
W=2g(1022−0)=2g⋅50=100g
6. Substitute the value of g:
Using g≈9.81m/s2:
W=100×9.81=981J
7. Final result:
The work required to slowly wind up the chain onto the axle is approximately:
W≈981J
Conclusion:
The closest answer to the work required to slowly wind up the chain is approximately 981 Joules.
The mass density (λ) of the chain is given as 2 kg/m. The total length (L) of the chain is 10 m. Therefore, the total mass (M) of the chain can be calculated as:
M=λ×L=2kg/m×10m=20kg
Consider a small segment of the chain of length dy at a height y from the bottom of the chain. The mass of this small segment (dm) can be expressed as:
dm=λ×dy=2kg/m×dy
The weight (dW) of this small segment is given by:
dW=dm×g=(2dy)×g
where g is the acceleration due to gravity (approximately 9.81m/s2).
The work done to lift this small segment from height y to the horizontal axis (which is at height 0) is:
dW=(2dy)×g×y
To find the total work done to lift the entire chain, we integrate dW from y=0 to y=10:
W=∫100(2g⋅y)dy
W=2g∫100ydy
W=2g(y22)100
W=2g(1022−0)=2g⋅50=100g
Using g≈9.81m/s2:
W=100×9.81=981J
The work required to slowly wind up the chain onto the axle is approximately:
W≈981J
The closest answer to the work required to slowly wind up the chain is approximately 981 Joules.
Topper's Solved these Questions
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (A)
Explore 32 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (B)
Explore 3 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART- C
Explore 4 Videos
- SEMICONDUCTORSBook:BANSALChapter:SEMICONDUCTORSExercise:CBSE Question
Explore 32 Videos
Similar Questions
A unifrom chain of mass M and length L lies on a table with nth part of it hanging off the table. Find the work required to slowly pull the hanging part up to the table.
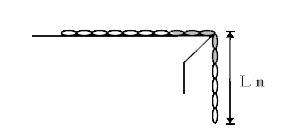
View SolutionA uniform chain is held on a frictionless table with one third of its length hanging over the edge. IF the chain has a length l and a mass m, how much work is required to pull the hanging part back on the table ?
View Solution
Knowledge Check
A uniform chain of mass m & length L is kept on a smooth horizontal table such that Lnportion of the chaing hangs from the table. The work dione required to slowly bringsthe chain completely on the table is
AmgLnBmgL2CmgLn2DmgL2n2A uniform chain of length L and mass M is lying on a smooth table and one-third of its length is hanging vertically down over the edge of the table. If g is the acceleration due to gravity, the work required to pull the hanging part on to the table is
AMgLBMgL/3CMgL/9DMgL/18A chain of length L and mass per unit length ρ is piled on a horizontal surface. One end of the chain is lifted vertically with constant velocity by a force P.
AP as function of height x of the end above the surface will be ρ(gx+v2)Bno energy will loss in this process.Cwork done by force will be 12ρgL2+ρv2LDloss in energy 12ρgLv2
A thin, uniform chain is hanging vertically and its bottom end is touching a table . The mass of the chain is m and its length is l . At the moment t=0, the chain is released. Calculate the force that the chain exerts on the table after t seconds.
View SolutionA uniform flexible chain of mass m and length 2l hangs in equilibrium over a smooth horizontal pin of negligible diameter. One end of the chain is given a small vertical displacement so that the chain slips over the pin. The speed of chain when it leaves the pin is
View SolutionA uniform chain of length L and mass M is lying on a smooth table and one third of its length is hanging vertically down over the edge of the table. If g is acceleration due to gravity, work required to pull the hanging part on to the table is
View SolutionA uniform flexible chain of mass m and length 2 hangs in equilibrium over a smooth horizontal pin of negligible diameter. One end of the chain is given a small vertical displacement so that the chain slips over the pin. The speed of chain when it leaves pin is-
View SolutionA uniform flexible chain of mass m and length l hangs in equilibrium over a smooth horizontal pin of neglible diameter. One end of the chain is given a small verticle displacement so that the chain slips over the pin. The speed of chain when it leaves pin is
View Solution